In triangle ABC , the equation of side BC is x−y=0. The circumcenter and orthocentre of triangle are (2, 3) and (5, 8), respectively. The equation of the circumcirle of the triangle is Solution in Tamil
x2+y2−4x−6y−27=0
x2+y2−4x−6y−36=0
x2+y2−4x−6y−24=0
x^(2)+y^(2)-4x-6y-15=0
The correct Answer is:2
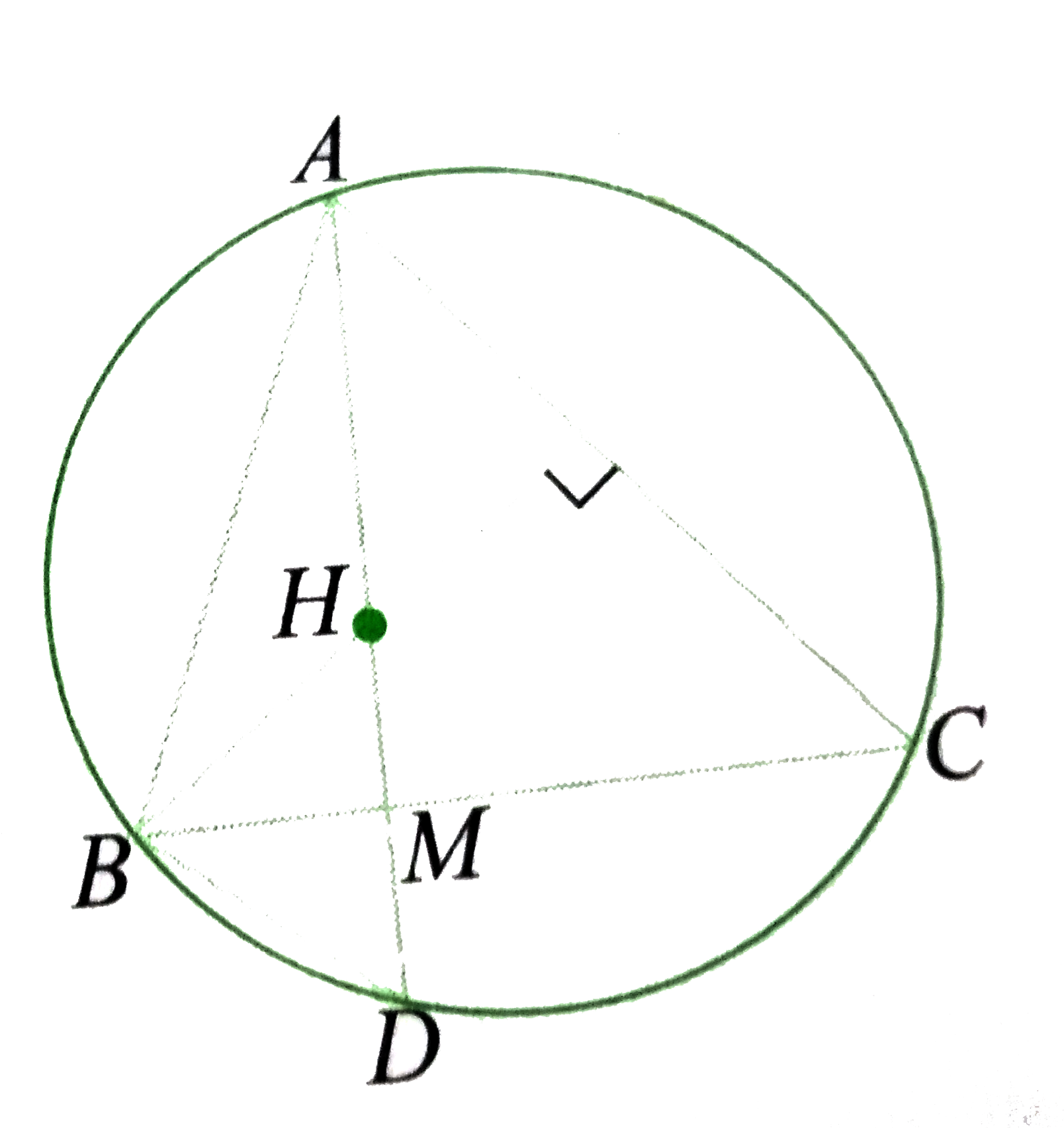
Let the orthocenter be H(5,8)
Now, ∠HBM=π2−C
Also, ∠DBC=∠DAC=π2−C
Hence, ΔCMH and ΔBMD are congruent. Therefore,
HM=MD
D is the image of H in the line x−y=0, which is D(8,5).
Thus, the equation of the circumcircle is
(x−2)2+(y−3)2=(8−2)2+(5−3)2
i.e., x2+y2−4x−6y−6y−27=0
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Multiple)25videos
CIRCLE
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Comprehension)46videos
CIRCLE
- BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.65videos
CIRCLE
- BOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension11videos
BINOMIAL THEOREM
- BOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank16videos
CIRCLES
Similar Questions
about to only mathematics
09:04View SolutionEach side of a square is of length 6 units and the centre of the square Is ( - 1, 2). One of its diagonals is parallel to x+y=0. Find the co-ordinates of the vertices of the square.
02:50View SolutionIf the circle x2+y2+2x+3y+1=0 cuts x2+y2+4x+3y+2=0 at AandB , then find the equation of the circle on AB as diameter.
View SolutionConsider a G.P. with first term (1+x)n, |x|<1, common ratio 1+x2 and number of terms (n+1). Let 'S' be sum of all the terms of the G.P., then
The coefficient of xn is 'S' is08:03View SolutionIf ax+by=10 is the chord of minimum length of the circle (x−10)2+(y−20)2=729 and the chord passes through (5,15) then the value of (4a+2b) is
View SolutionIn triangle ABC , the equation of side BC is x−y=0. The circumcenter and orthocentre of triangle are (2, 3) and (5, 8), respectively. The equation of the circumcirle of the triangle is x2+y2−4x+6y−27=0 x2+y2−4x−6y−27=0 x2+y2+4x+6y−27=0 x2+y2+4x+6y−27=0
04:33View SolutionThe equations of the sides of a triangle are x+y-5=0, x-y+1=0, and y-1=0. Then the coordinates of the circumcenter are
07:05View SolutionIf O and O' denote respectively the circumcente and orthocentre of ΔABC, thenO'A+O'B+O'C is equal orthocentre of delta ABC, then O'A+O'B+O'C is equal to
02:38View SolutionThe orhtcentre and the centroid of ΔABC are (5,8) and (3,143) respectively. The equation of the side BC is x-y=0 , Given that the image of the orthocentre of a triangle with respect to any side lies on the circumcircle of that triangle, then the diameter of the circumcircle of ΔABC is
01:46View SolutionThe orthocentre of the triangle whose sides are given by equations x=1,y=0 and x+y−2=0, is
04:20View SolutionIn triangle ABC , the equation of the right bisectors of the sides AB and AC are x+y=0 and y−x=0 , respectively. If A≡(5,7) , then find the equation of side BC.
06:55View SolutionThe equations of the perpendicular bisectors of the sides ABandAC of triangle ABC are x−y+5=0 and x+2y=0 , respectively. If the point A is (1,−2) , then find the equation of the line BC.
11:28View SolutionIn triangle ABC , the equation of side BC is x−y=0. The circumcenter and orthocentre of triangle are (2, 3) and (5, 8), respectively. The equation of the circumcirle of the triangle is a) x2+y2−4x+6y−27=0 b) x2+y2−4x−6y−27=0 c) x2+y2+4x−6y−27=0 d) x2+y2+4x+6y−27=0
03:52View SolutionThe equations of the sides of a triangle are x+y-5=0, x-y+1=0, and y-1=0. Then the coordinates of the circumcenter are
10:48View SolutionIf a triangle ABC,A≡(1,10), circumcenter ≡(−13,23), and orthocentre ≡(113,43) , then the coordinates of the midpoint of the side opposite to A are
03:43View SolutionThe equations of the perpendicular bisectors of the sides ABandAC of triangle ABC are x−y+5=0 and x+2y=0 , respectively. If the point A is (1,−2) , then find the equation of the line BC.
02:57View Solution

