Question

Let $S$
be a square of nit area. Consider any quadrilateral, which has none
vertex on each side of $S\stackrel{.}{}$
If $a,b,candd$
denote the lengths of the sides of het quadrilateral, prove that $2\le a^2+b^2+c^2+x^2\le 4.$

Explanation

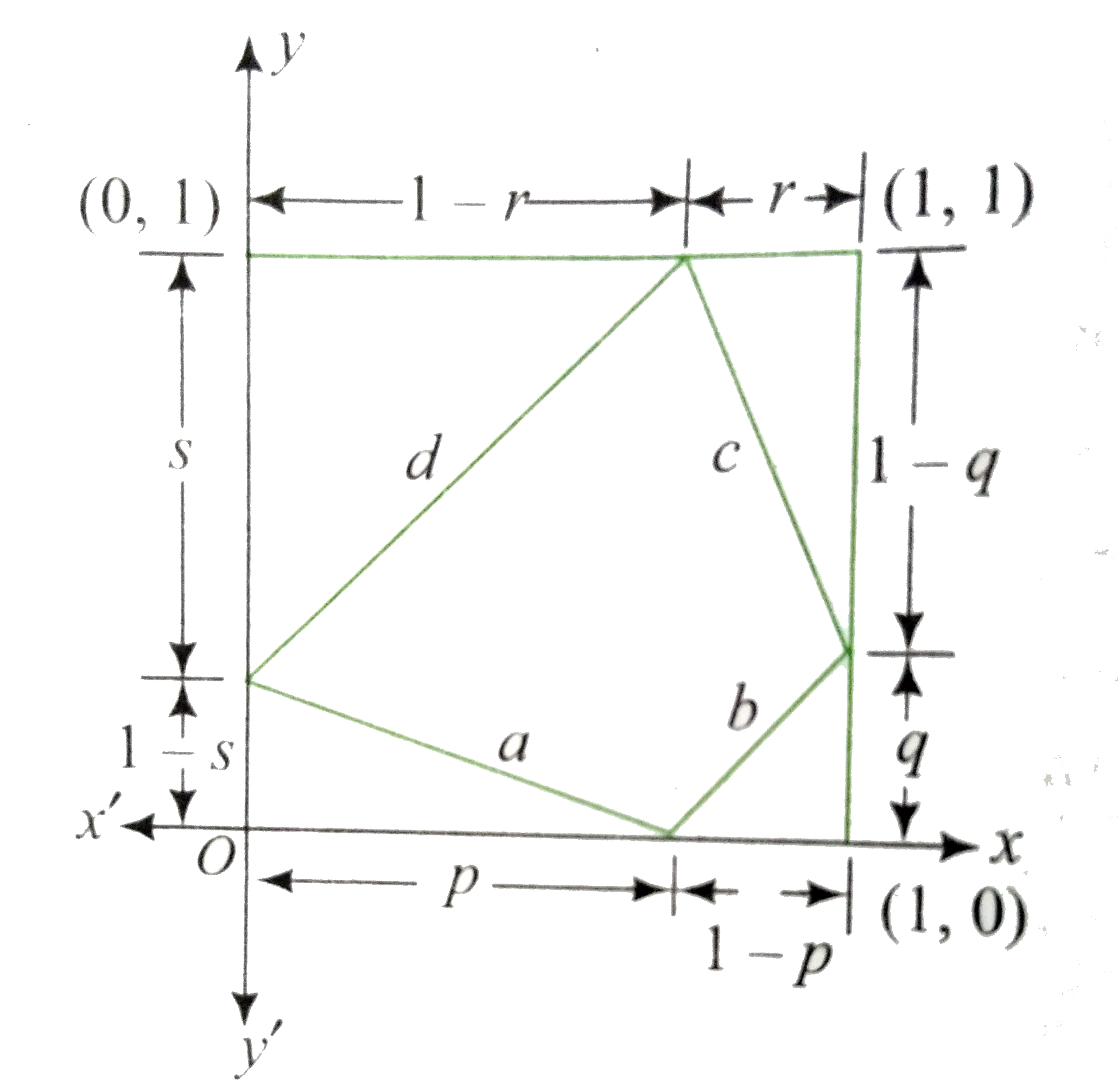
From the figure
`a^(2) = p^(2) + (1 - s)^(2)`
b^(2) = (1 - p)^(2) + q^(2)`
`a^(2) + b^(2) + c^(2) + d^(2)= [ p^(2) + (1 - p)^(2)] + [q^(2) + ( 1 - q)^(2) ] `
` [ r^(2) + (1 - r)^(2)] + [s^(2) + ( 1 - s)^(2) ]` , where p , q , r, s `in`[0, 1]
Now consider the function
`y = x^(2) + (1 - x)^(2), 0 le x le 1`
`rArr y = 2x^(2) - 2x + 1 = 2 (x - (1)/(2))^(2) + (1)/(2)`
Hence, minimum value is `1//2` when x = `1//2` and maximum value is at x = 1 , which is 1. Therefore, minimum value of `a^(2) + b^(2) + c^(2) d^(2)` is `1//2+ 1//2 + 1//2 +1//2 = 2` and maximum value is `1 + 1 + 1 + 1 = 4`.