four fair dice D1,D2,D3andD4 each having six faces numbered 1,2,3,4,5 and 6 are rolled simultaneously. The probability that D4 shows a number appearing on one of D1,D2,D3 is Solution in Tamil
91216
108216
125216
127216
The correct Answer is:A
As one of the dice shows a number appearing on one of P1,P2andP3 .
Thus, three cases arise.
(i) All show same number.
(ii) Number appearing on D4 oppears on any one of D1,D2andD3
(iii) Number appearing on D4 appears on any two of D1,D2andD3
Sample space =6×6×6×6=64 favorite events =Case I or Case II or Case III
CaseI First we should select one number for D4 winch appears on all i.e ^6C1×1
Case II For D4 there are ^6C1 ways. Now, it appears on any one of D1,D2andD3i.e3C1×1
For other two there are 5×5 ways
⇒6C1×3C1×1×5×5
For D4 there are ^6C1 ways now it apperes on any two of D1,D2andD3
⇒3C2×12
For another one there are 5 ways
⇒6C1×3C2×12×5
Thus ,Probability =6C1+6C1×3C1×52+6C1×3C2×564
=6(1+75+15)64
=91216
Similar Questions
The equation of the plane passing through A(x1,y1,z1) and
containg the line x−x2d1=y−y2d2=z−z2d3 is
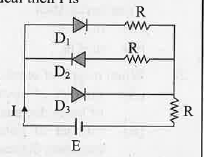
View Solutionin the foll. Circuit of PN junction , diodes D1,D2andD3 are ideal then I is
View SolutionIf d1,d2,d3 be the three distinct diagonals of a regular octagon, such that d1<d2<d3, find the ratio of the inradius to the circumradius of the octagon.
View SolutionIf distances of (−1,2,3) from x,y,z axis are d1,d2,d3 respectively and the distances from xy,yz,zx planes are d4,d5,d6 then the value of 6∑i=1di , is ___
View SolutionIf D=diag(d1,d2,d3,…,dn) where d≠0 for all I=1,2,…,n, then D−1is equal to
View SolutionIf D=diag(d1,d2,d3,…,dn) where d≠0 for all I=1,2,…,n, then D−1is equal to
View SolutionLet D = diag [d1,d2,d3] where none of d1,d2,d3 is 0. prove that: D−1=diag[d−11,d−12,d−13].
View SolutionIf A = diag (d1,d2,d3,….dn) then det A is equal to
View Solution
