In Schrodinger wave mechanical model Ψ2(r,θ,ϕ) represents :Solution in Punjabi
amplitude of electron wave
probability density of electron
total probability of finding electron around the nucleus
orbit
Step by step video & image solution for In Schrodinger wave mechanical model Psi^(2)(r,theta,phi) represents : by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Which hybrid orbital is used by carbon atoms in the following molecules? CH3-CH3
View SolutionA small piece of mass m moves in such a way the P.E. =−12mkr2. Where k is a constant and r is the distance of the particle from origin. Assuming Bohr's model of quantization of angular momentum and circular orbit, r is directly proportional to :
View SolutionCalculate ΔrG for the reaction at 27∘C
H2(g)+2Ag+(aq)⇔2Ag(s)+2H+(aq)
Given : PH2=0.5 bar, [Ag+]=10−5M,
[H+]=10−3M,ΔrG∘[Ag+(aq)]=77.1kJ/mol
View SolutionGiven that (sec theta+tan theta)(sec phi+tan phi)(sec psi+tan psi)=(sec theta-tan theta)(sec phi-tan phi)(sec psi-tan psi) then Prove that value of each side +-1 is
View SolutionIn Schrodinger wave mechanical model Ψ2(r,θ,ϕ) represents :
View SolutionIn schrodinger wave equation ∇2ψ+?π2mh2(E−V)ψ=0 ? is- "
View SolutionIf x=cosθcosϕ+sinθsinϕcosψ,y=cosθsinϕ−sinθcosϕcosψ and z=sinθsinψ, then x2+y2+z2 equals :
View SolutionIn Schrodinger wave mechanical model Ψ2(r,θ,ϕ) represents :
View SolutionWhen Schrodinger wave equation in polar coordinates is solved the solution for Phi is of the form Psi (r, theta , phi)= R(r) , Y(theta , phi) . Here R(r) is radial part of wave function and Y(theta, phi) is angular part of the wave function. The region or space where probability of finding electron is zero is called nodal surface. If the probability of finding electron is zero then Psi^2 (r, theta, phi)=0 implies Psi (r, theta, phi)=0 If the radial wave function is equal to zero we get radial node and if angular part is equal to zero we get angular nodes. Total no. of nodes for any orbital = n - 1. Where ‘n’ is principal quantum number. The d-orbital with two nodal surfaces formed cones
View SolutionWhen Schrodinger wave equation in polar coordinates is solved the solution for Φ is of the form Ψ(r,θ,ϕ)=R(r),Y(θ,ϕ). Here R(r) is radial part of wave function and Y(θ,ϕ) is angular part of the wave function. The region or space where probability of finding electron is zero is called nodal surface. If the probability of finding electron is zero then Ψ2(r,θ,ϕ)=0⇒Ψ(r,θ,ϕ)=0
If the radial wave function is equal to zero we get radial node and if angular part is equal to zero we get angular nodes. Total no. of nodes for any orbital = n - 1. Where ‘n’ is principal quantum number.
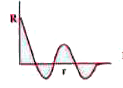represents
View SolutionWhen Schrodinger wave equation in polar coordinates is solved the solution for Φ is of the form Ψ(r,θ,ϕ)=R(r),Y(θ,ϕ). Here R(r) is radial part of wave function and Y(θ,ϕ) is angular part of the wave function. The region or space where probability of finding electron is zero is called nodal surface. If the probability of finding electron is zero then Ψ2(r,θ,ϕ)=0⇒Ψ(r,θ,ϕ)=0
If the radial wave function is equal to zero we get radial node and if angular part is equal to zero we get angular nodes. Total no. of nodes for any orbital = n - 1. Where ‘n’ is principal quantum number.
Number of radial nodes for 4f orbital
View SolutionPsi^2 (r , theta , phi) represents (for schrodinger wave mechanical model)
View SolutionIn the schrodinger wave equation, psi represents______.
View SolutionIn Schrodinger 's wave equation , the symbol Ψ represents the
View SolutionQuantum Mechanical Model and Schrodinger Wave Equation||Wave Function for Atomic Orbitals and their Graphs||Radial Probability Distribution Function and Graph
View Solution