A playground in the town is in the form of a kite. The perimeter is 106 metres. If one of its sides is 23 metres, what are the lengths of other three sides?
To find the lengths of the other three sides of the kite-shaped playground, we can follow these steps:
Step 1: Understand the properties of a kite
A kite has two pairs of adjacent sides that are equal. This means if one side is given, the side directly opposite it will be equal to it.
Step 2: Identify the known values
We know:
- The perimeter of the kite is 106 meters.
- One of the sides (let's say AB) is 23 meters.
Step 3: Set up the equation for the perimeter
The perimeter of the kite can be expressed as:
AB+BC+CD+DA=106
Since AB = DA = 23 meters (because they are equal), we can rewrite the equation as:
23+BC+CD+23=106
Step 4: Simplify the equation
Combine the known values:
46+BC+CD=106
Step 5: Substitute the equal sides
Since BC and CD are equal (let's denote them as X), we can substitute:
46+X+X=106
This simplifies to:
46+2X=106
Step 6: Solve for X
To isolate X, subtract 46 from both sides:
2X=106−46
2X=60
Now, divide by 2:
X=30
Step 7: Determine the lengths of all sides
Now we can summarize the lengths of the sides:
- AB = 23 meters
- AD = 23 meters
- BC = 30 meters
- CD = 30 meters
Final Answer
The lengths of the sides of the kite are:
- Two sides of 23 meters each (AB and AD)
- Two sides of 30 meters each (BC and CD)
---
A kite has two pairs of adjacent sides that are equal. This means if one side is given, the side directly opposite it will be equal to it.
We know:
- The perimeter of the kite is 106 meters.
- One of the sides (let's say AB) is 23 meters.
The perimeter of the kite can be expressed as:
AB+BC+CD+DA=106
23+BC+CD+23=106
Combine the known values:
46+BC+CD=106
Since BC and CD are equal (let's denote them as X), we can substitute:
46+X+X=106
This simplifies to:
46+2X=106
To isolate X, subtract 46 from both sides:
2X=106−46
2X=60
X=30
Now we can summarize the lengths of the sides:
- AB = 23 meters
- AD = 23 meters
- BC = 30 meters
- CD = 30 meters
The lengths of the sides of the kite are:
- Two sides of 23 meters each (AB and AD)
- Two sides of 30 meters each (BC and CD)
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBook:NCERT EXEMPLARChapter:UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYExercise:APPLICATIONS, GAMES AND PUZZLES
Explore 3 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBook:NCERT EXEMPLARChapter:UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYExercise:THINK AND DISCUSS
Explore 2 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBook:NCERT EXEMPLARChapter:UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYExercise:THINK AND DISCUSS
Explore 2 Videos
- SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTBook:NCERT EXEMPLARChapter:SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTExercise:Exercise
Explore 52 Videos
- VISUALISING SOLID SHAPES Book:NCERT EXEMPLARChapter:VISUALISING SOLID SHAPES Exercise:EXERCISE
Explore 91 Videos
Similar Questions
Knowledge Check
If the perimeter of a rectangular plot is 34 metres and its area is 60 square metres, what is the length of each of the shorter side?
A10mB15mC17mD5mIf the perimeter of a regular hexagon is x metres, then the length of each of its sides is
A(x + 6) metresB(x + 6) metresC(x ÷ 6) metresD(6 ÷ x) metresThe area of a triangle is 615 m2. If one of its sides is 123 metre, find the length of the perpendicular dropped on that side from opposite vertex.
A15 metresB12 metresC10 metresDNone of these
If perimeter of a regular hexagon is 108 cm, what is the length of its sides ?
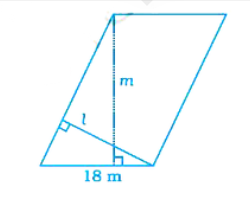
View SolutionPerimeter of a parallelogram shaped land is 96 m and its area is 270 square metres। If one of the sides of this parallelogram is 18 m, find the length of the other side। Also, find the lengths of altitudes 1 and m (Fig. 9.42).
View SolutionIf one side of a rhombus if 17 cm and length of one of its diagonals is 16 cm. What is the length of other diagonal (incm)?
View SolutionA triangle has a perimeter of 200. If two of its sides are equal and the third side is 20 more than the equal sides, what is the length of the third side?
View SolutionIf sum of two sides of a triangle is 17cm and its perimeter is 30cm, then what is the length of third side.
View Solution