Length of tape required to cover the edges of a semicircular disc of radius 10 cm is
62.8 cm
51.4 cm
31.4 cm
15.7 cm
The correct Answer is:B
To find the length of tape required to cover the edges of a semicircular disc with a radius of 10 cm, we will follow these steps:
Step 1: Understand the shape
We have a semicircular disc, which means it is half of a full circle. The edges we need to cover include the curved edge and the straight edge (diameter).
Step 2: Calculate the circumference of a full circle
The formula for the circumference (C) of a full circle is given by:
C=2πr
where r is the radius of the circle.
Step 3: Calculate the circumference of the semicircle
Since we only need the curved part of the semicircle, we take half of the circumference of the full circle:
Curved part=12×2πr=πr
Step 4: Calculate the diameter of the semicircle
The diameter (d) of the semicircle is given by:
d=2r
Step 5: Combine the lengths
To find the total length of tape required, we need to add the length of the curved part and the diameter:
Total Length=Curved part+Diameter
Total Length=πr+2r
Step 6: Substitute the radius
Now, substitute r=10 cm into the equation:
Total Length=π×10+2×10
Total Length=10π+20
Step 7: Calculate the numerical value
Using π≈3.14:
Total Length=10×3.14+20
Total Length=31.4+20
Total Length=51.4 cm
Final Answer
The length of tape required to cover the edges of the semicircular disc is 51.4 cm.
---
We have a semicircular disc, which means it is half of a full circle. The edges we need to cover include the curved edge and the straight edge (diameter).
The formula for the circumference (C) of a full circle is given by:
C=2πr
where r is the radius of the circle.
Since we only need the curved part of the semicircle, we take half of the circumference of the full circle:
Curved part=12×2πr=πr
The diameter (d) of the semicircle is given by:
d=2r
To find the total length of tape required, we need to add the length of the curved part and the diameter:
Total Length=Curved part+Diameter
Total Length=πr+2r
Now, substitute r=10 cm into the equation:
Total Length=π×10+2×10
Total Length=10π+20
Using π≈3.14:
Total Length=10×3.14+20
Total Length=31.4+20
Total Length=51.4 cm
The length of tape required to cover the edges of the semicircular disc is 51.4 cm.
Topper's Solved these Questions
- PERIMETER AND AREABOOK - NCERT EXEMPLARCHAPTER - PERIMETER AND AREAEXERCISE - Exercise (Fill in the blanks)
19 Videos
- PERIMETER AND AREABOOK - NCERT EXEMPLARCHAPTER - PERIMETER AND AREAEXERCISE - Exercise (True or false)
7 Videos
- PERIMETER AND AREABOOK - NCERT EXEMPLARCHAPTER - PERIMETER AND AREAEXERCISE - Think and Discuss
7 Videos
- LINES AND ANGLES BOOK - NCERT EXEMPLARCHAPTER - LINES AND ANGLES EXERCISE - Exercise (fill in the blanks)
3 Videos
- PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESBOOK - NCERT EXEMPLARCHAPTER - PRACTICAL GEOMETRY SYMMETRY AND VISUALISING SOLID SHAPESEXERCISE - EXERCISE
108 Videos
Similar Questions
Knowledge Check
A circular disc of radius 20cm is cut from one edge of a larger circular disc of radius 50cm. The shift of centre of mass is
A5.7cmB−5.7cmC3.2cmD−3.2cmIn the figure shown a hole of radius 2 cm is made in a semicircular disc of radius 6π at a distance 8 cm from the centre C of the disc. The distance of the centre of mass of this system from point C is :
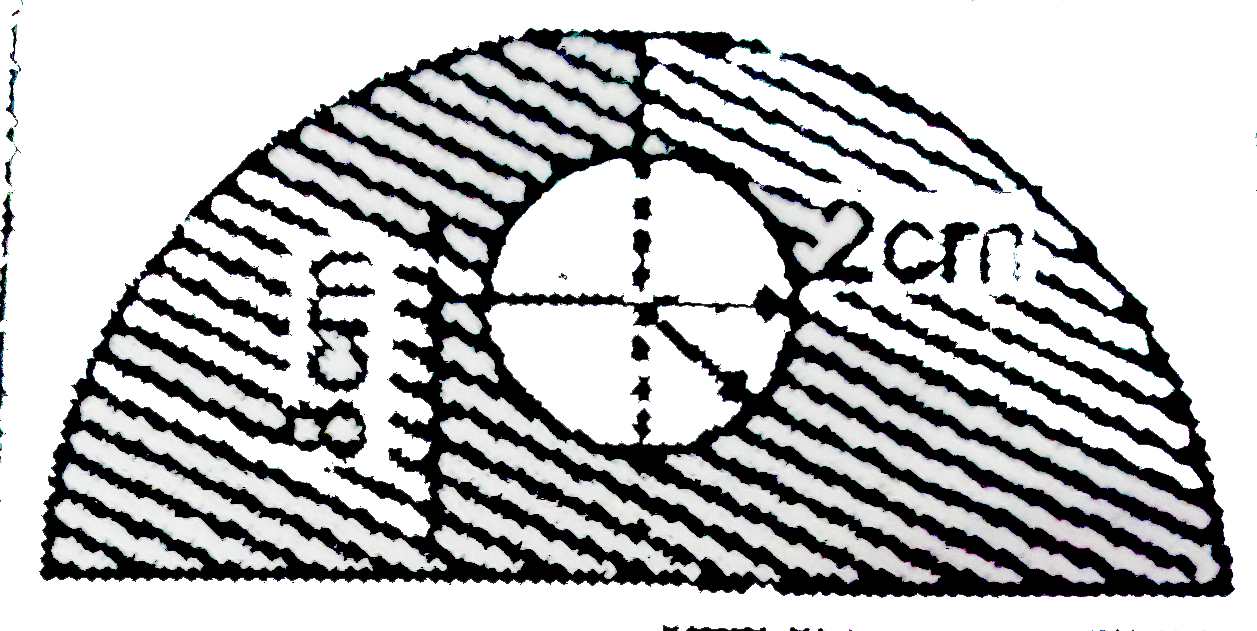 A4 cmB8cmC6 cmD12 cm
A4 cmB8cmC6 cmD12 cmWhat is the area between a square of side 10 cm and two inverted semicircular, cross-sections each of radius 5 cm inscribed in the square?
A17.5cm2B18.5cm2C20.5cm2D21.5cm2
Calculate the moment of inertia of a circular disc of radius 10cm, thickness 5mm and uniform density 8gcm−3, about a transverse axis through the centre of the disc.
View SolutionA closed aquarium of dimensions 30cm×25cm×20cm is made up entirely of glass plates held together with tapes. The total length of tape required to hold the plates together (ignore the overlapping tapes) is (a) 75 cm (b) 120 cm (c) 150 cm (d) 300 cm
View SolutionCalculate the moment of Inertia of a semicircular disc of mass M and radius R about an axis passing through its centre and perpendicular to its plane.
View SolutionFrom a semicircular disc of radius r1, another semicircular disc of radius r2 is removed. Find the c.m. of the remaining position.
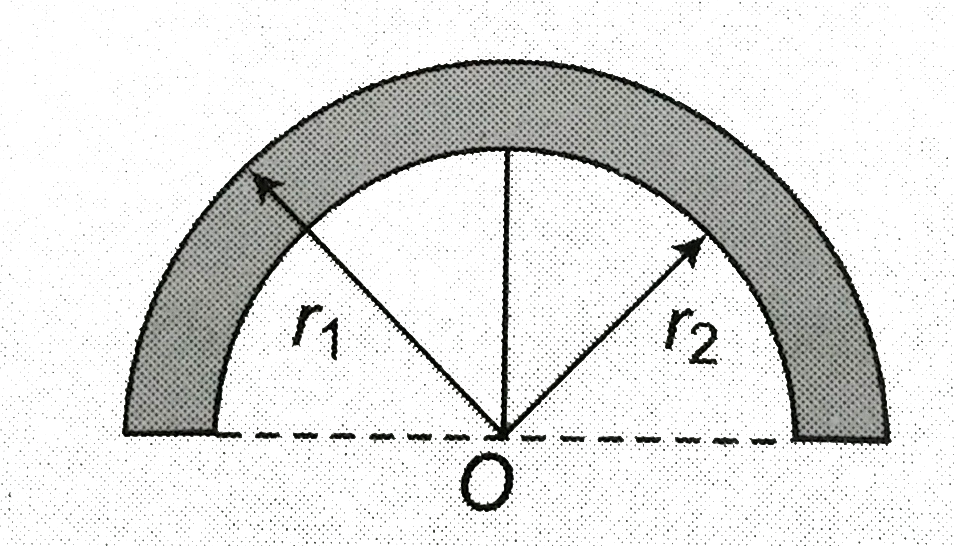
View SolutionFigure shows a composite body made up by welding a thin rod (of mass m and length r) normally at the centre P of a thin semicircular disc (of mass m and radius r). What are corrdinates of centre of mass of the system ?

View Solution