Two line segments may intersect at two points
To determine whether the statement "Two line segments may intersect at two points" is correct or incorrect, we can analyze the properties of line segments and their intersections.
1. Understanding Line Segments: A line segment is a part of a line that is bounded by two distinct endpoints. For example, let's define two line segments: PQ and MN.
2. Drawing the First Line Segment: Let's draw the first line segment, PQ. We can represent it as follows:
- Point P (starting point)
- Point Q (ending point)
3. Drawing the Second Line Segment: Now, we will draw the second line segment, MN. We can position it in various ways relative to PQ:
- It can intersect PQ at one point.
- It can be parallel to PQ and not intersect at all.
- It can overlap with PQ, but still, they will intersect at just one point.
4. Analyzing Intersections:
- If we position the two line segments such that they cross each other, they will intersect at exactly one point.
- If we try to draw them in such a way that they intersect at two points, we realize that this is not possible because a line segment can only connect two endpoints.
5. Conclusion: Based on our analysis, we can conclude that two line segments cannot intersect at two points. They can either intersect at one point, be parallel (not intersecting), or be collinear (overlapping), but never intersect at two distinct points.
6. Final Statement: Therefore, the statement "Two line segments may intersect at two points" is incorrect. The correct statement is that two line segments intersect at most at one point.
- Point P (starting point)
- Point Q (ending point)
- It can intersect PQ at one point.
- It can be parallel to PQ and not intersect at all.
- It can overlap with PQ, but still, they will intersect at just one point.
- If we position the two line segments such that they cross each other, they will intersect at exactly one point.
- If we try to draw them in such a way that they intersect at two points, we realize that this is not possible because a line segment can only connect two endpoints.
Topper's Solved these Questions
- GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - GEOMETRYEXERCISE - Exercise
56 Videos
- GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - GEOMETRYEXERCISE - Exercise (Fill in the blanks to make the statements true:)
21 Videos
- FRACTIONS AND DECIMALS BOOK - NCERT EXEMPLARCHAPTER - FRACTIONS AND DECIMALS EXERCISE - ACTIVITY
1 Videos
- INTEGERS BOOK - NCERT EXEMPLARCHAPTER - INTEGERS EXERCISE - Exercise
42 Videos
Similar Questions
Tell which of the following statements are true (T) and which are false (F): The intersection of two segments may be segment. Two segments may intersect at a point which is not any end-point of either segments containing it. Every ray is a segment. Every segment is a ray.
View SolutionWhich of the following statements are true ?
(i) A line segment has no definite length
(ii) A ray has no end point
(iii) A line has definite length
(iv) A line AB is the same as line BA
(v) A ray AB is the same as ray BA
(vi) Two distnict points always determine a unique line
(vii) Three lines are concurrent if they have a common point
(viii)T wo distinct lines cannot have more than one point in common
(ix) Two intersecting lines cannot be both parallel to the same line
(x) open half line is the same thing as ray
(xi) Two lines may intersect in two points
(xii) Two lines are parallel only when they have no point in common
View Solution
Knowledge Check
Two line segments PQ and RS intersect at X in such a way that XP=XR. If ∠PSX=∠RQX, then one must have
APR=QSBPS=RQC∠XSQ=∠XRPDar(ΔPXR)=ar(ΔQXS)
Polygons a polygon is a closed curve (figure)formed by the line segments such that : (i)no two line segments intersect except at their end -points.(ii)no two line segments with a common end points are coincident.
View Solution(i) No two line segments intersect except at their endpoints.
View SolutionWrite 'T' for true and 'F' for false in case of the following statement
Two intersecting lines intersect at a point.
View SolutionWhich of the following statements are true and which are false ?
If two line segments do not intersect, they are parallel.
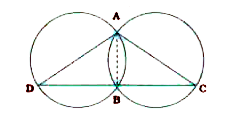
View SolutionTwo circles intersect at two points A and B . AD and AC are diameters to the two circles .Prove that B lies on the line segment DC.
View SolutionTwo circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D and P, Q respectively (see Fig. 10.40). Prove that ∠ACP =∠QCD.
View SolutionTwo circles intersect at two points A and B. AD and AC are diameters to the two circles (see Fig.10.34). Prove that B lies on the line segment DC.
View Solution
