For a system of partice, the vector sum of all the internal forces is....A... Here, A refers to
Non zero
Infinite
Zero
None of the above
To solve the question regarding the vector sum of all the internal forces in a system of particles, we can follow these steps:
1. Understanding Internal Forces:
- Internal forces are the forces that the particles within a system exert on each other. According to Newton's third law, for every action, there is an equal and opposite reaction. This means that if particle A exerts a force on particle B, then particle B exerts an equal and opposite force on particle A.
2. Applying Newton's Third Law:
- For any two particles in the system, the internal forces they exert on each other will cancel each other out when summed. For example, if particle 1 exerts a force F12 on particle 2, then particle 2 exerts a force F21=−F12 on particle 1.
3. Summing Internal Forces:
- When we consider all the internal forces acting within the system, each force has a corresponding equal and opposite force. Therefore, when we take the vector sum of all internal forces, they will cancel each other out.
4. Conclusion:
- Since all internal forces cancel each other, the vector sum of all internal forces in the system is zero. This can be expressed mathematically as:
∑Finternal=0
5. Final Answer:
- Therefore, the vector sum of all the internal forces in a system of particles is zero.
- Internal forces are the forces that the particles within a system exert on each other. According to Newton's third law, for every action, there is an equal and opposite reaction. This means that if particle A exerts a force on particle B, then particle B exerts an equal and opposite force on particle A.
- For any two particles in the system, the internal forces they exert on each other will cancel each other out when summed. For example, if particle 1 exerts a force F12 on particle 2, then particle 2 exerts a force F21=−F12 on particle 1.
- When we consider all the internal forces acting within the system, each force has a corresponding equal and opposite force. Therefore, when we take the vector sum of all internal forces, they will cancel each other out.
- Since all internal forces cancel each other, the vector sum of all internal forces in the system is zero. This can be expressed mathematically as:
∑Finternal=0
- Therefore, the vector sum of all the internal forces in a system of particles is zero.
Topper's Solved these Questions
- BOOK - PHYSICS WALLAHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - NEET PAST 5 YEARS QUESTIONS22videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - PHYSICS WALLAHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - NEET PAST 5 YEARS QUESTIONS22videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - PHYSICS WALLAHCHAPTER - SEMICONDUCTOR ELECTRONICS EXERCISE - NEET PAST 5 YEAR QUESTION23videos
SEMICONDUCTOR ELECTRONICS
- BOOK - PHYSICS WALLAHCHAPTER - THERMAL PROPERTIES OF MATTER EXERCISE - NEET Past 5 Years Questions12videos
THERMAL PROPERTIES OF MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
Consider the following statements
I. The rate of change of the total linear momentum of a system consisting of many particles is proportional to the vector sum of all the internal forces due to inter-particle interaction.
The internal forces can change the kinetic energy of the system of particles are not the linear momentum of the system. Choose the correct option.AOnly IBOnly IICI and IIDNone of these - Question 2 - Select One
The reduction of zinc oxide is done using ...A... Here, A refers to
Acopper matteBblister copperCcokeDNone of these - Question 3 - Select One
Below point 'A' FeO can….A…..Here, A refers to
Abe reduced by carbon monoxide onlyBbe reduced by both carbon monoxide and carbonCbe reduced by carbon onlyDnot be reduced by both carbon monoxide
Assertion : A body can be at rest even when it is under the action of may number of external forces
Reason : Because vector sum of the all external forces is zero .View Solution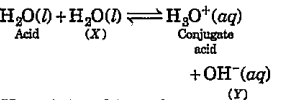
Here, (X) and (Y) refer toView SolutionConsider the following statements
The motion of the CM depends upon the external forces,
The vector sum of internal forces of a system is zero. Choose the correct option.View SolutionA is an essential condition for coherent sources. Here, A refers to
View Solution….A…. Is the sum of atomic masses of the elements present in a molecule here A refers to
View Solution

