What is the radius of the circle whose area is equal to the sum of the areas of two circles whose radii are 15 cm and 8 cm?
उस वृत्त की त्रिज्या कितनी है, जिसका क्षेत्रफल दो वृत्तों के क्षेत्रफलों के योग के बराबर है, जिनकी त्रिज्याएँ 15 सेमी तथा 8 सेमी हैं?
14 cm
15 cm
13 cm
17 cm
To find the radius of the circle whose area is equal to the sum of the areas of two circles with radii 15 cm and 8 cm, we can follow these steps:
Step 1: Calculate the area of the first circle
The formula for the area of a circle is given by:
Area=πr2
For the first circle with radius r1=15 cm:
Area1=π(15)2=π×225=225πcm2
Step 2: Calculate the area of the second circle
For the second circle with radius r2=8 cm:
Area2=π(8)2=π×64=64πcm2
Step 3: Calculate the sum of the areas of the two circles
Now, we add the areas of both circles:
Total Area=Area1+Area2=225π+64π=(225+64)π=289πcm2
Step 4: Set the area of the new circle equal to the total area
Let R be the radius of the new circle. The area of the new circle can also be expressed as:
Area=πR2
Setting this equal to the total area we calculated:
πR2=289π
Step 5: Simplify the equation
We can divide both sides of the equation by π (assuming π≠0):
R2=289
Step 6: Solve for R
Taking the square root of both sides gives us:
R=√289=17cm
Thus, the radius of the circle is 17 cm.
---
The formula for the area of a circle is given by:
Area=πr2
For the first circle with radius r1=15 cm:
Area1=π(15)2=π×225=225πcm2
For the second circle with radius r2=8 cm:
Area2=π(8)2=π×64=64πcm2
Now, we add the areas of both circles:
Total Area=Area1+Area2=225π+64π=(225+64)π=289πcm2
Let R be the radius of the new circle. The area of the new circle can also be expressed as:
Area=πR2
Setting this equal to the total area we calculated:
πR2=289π
We can divide both sides of the equation by π (assuming π≠0):
R2=289
Taking the square root of both sides gives us:
R=√289=17cm
Topper's Solved these Questions
Similar Questions
What is the radius of a circle whose area is equal to the sum of the area of two circles whose radii are 20 cm and 21 cm .
View SolutionThe radii of two circles are 20 cm and 13 cm, respectively. Find the radius of the circle which has a circumference equal to the sum of the circumference of two circles.
दो वृत्त की त्रिज्या क्रमशः 20 सेमी और 13 सेमी है। उस वृत्त की त्रिज्या ज्ञात कीजिए जिसमें दो वृत्त की परिधि के योग के बराबर एक परिधि है।
View Solution
Knowledge Check
The radius of a circle is a side of a square. The ratio of the areas of the circle and the square is
A2:πB1:πCπ:1Dπ:2
What will be the area of a circle whose radius is √5 cm?
एक वृत्त का क्षेत्रफल क्या होगा जिसकी त्रिज्या √5 सेमी है ?
View SolutionThe distance between the centres of two circles is 24 cm. If the radius of the two circles are 4cm and 8cm, then what is the sum of the lengths (in cm) of the direct common tangent and the transverse common tangent?
दो वृत्तों के केन्द्र के मध्य की दूरी 24सेमी. है। यदि दो वृत्त की त्रिज्या 4 सेमी. तथा 8 सेमी है, तो उभयनिष्ठ अनुस्पर्श रेखा तथा तिर्यक उभयनिष्ठ अनुस्पर्श रेखा की लम्बाई (सेमी. में) का योग क्या है?
View SolutionWhat is the area of a triangle whose sides are 3 cm, 4 cm and 5 cm?
उस त्रिभुज का क्षेत्रफल ज्ञात करें जिसकी भुजाएँ 3 सेमी, 4 सेमी तथा 5 सेमी की हैं |
View SolutionThe length of a rectangle is 6 cm more than its breadth. And its perimeter is 100 cm. If the area of this rectangle is nearest equal to the area of a circle, then what will be the circumference of this circle ?
एक आयत की लम्बाई, उसकी चौड़ाई से 6 cm अधिक है और उसका परिमाप 100 cm है | यदि इस आयत का क्षेत्रफल एक वृत्त के क्षेत्रफल के निकटतम बराबर है, तो उस वृत्त की परिधि कितनी है?
View SolutionABCD passes through the centres of the three circles as shown in the figure. AB=2 cm and CD=1 cm. If the area of middle circle is the average of the areas of the other two circles, then what is the length (in cm) of BC?
जैसा कि आकृति में दर्शाया गया है, ABCD तीनों वृत्तों के केन्द्रों से गुजरती है। AB=2 से, मी. तथा CD=1 से. मी. हैं। यदि मध्य वृत्त का क्षेत्रफल, शेष दोनो वृत्तों के क्षेत्रफलो का औसत है, तो BC की लंबाई (से.मी. में) क्या हैं?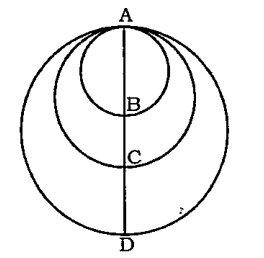
View SolutionThe volume of a right circular cone is equal to the volume of a right circular cylinder. The height and the radius of the cylinder are 9 cm and 20 cm respectively. If the height of the cone is 108 cm, then its radius (in cm) is-
लंब वृत्ताकार शंकु का आयतन लंब वृत्ताकार सिलेंडर के आयतन बराबर है। सिलेंडर की ऊँचाई और त्रिज्या क्रमशः 9 सेमी और 20 सेमी हैं। यदि शंकु की ऊचाई 108 सेमी है, तो उसकी त्रिज्या कितनी (सेमी में) होगी?
View SolutionWhat is the area of a triangle whose sides are 7cm, 24cm and 25 cm?
उस त्रिभुज का क्षेत्रफल ज्ञात करें जिसकी भुजाएँ 7 सेमी, 24 सेमी और 25 सेमी की हैं |
View Solution