A thin disc of mass m and radius r is moving up an inclined plane. Before ascending this plane disc was rolling, horizontally without slipping with speed $υ$ . The maximum height to which it can rise is

$\frac{1}{3} \frac{υ^{2}}{2 g}$

$\frac{2 υ^{2}}{3 g}$

$\frac{3 υ^{2}}{4 g}$

$\frac{υ^{2}}{g}$

Text Solution

Verified by Experts

The correct Answer is:C

A disc of radius $R$ and mass $M$ is rolling horizontally without slipping with speed with speed $v$ . It then moves up an incline as shown in figure. The maximum height upto which it can reach is.
.
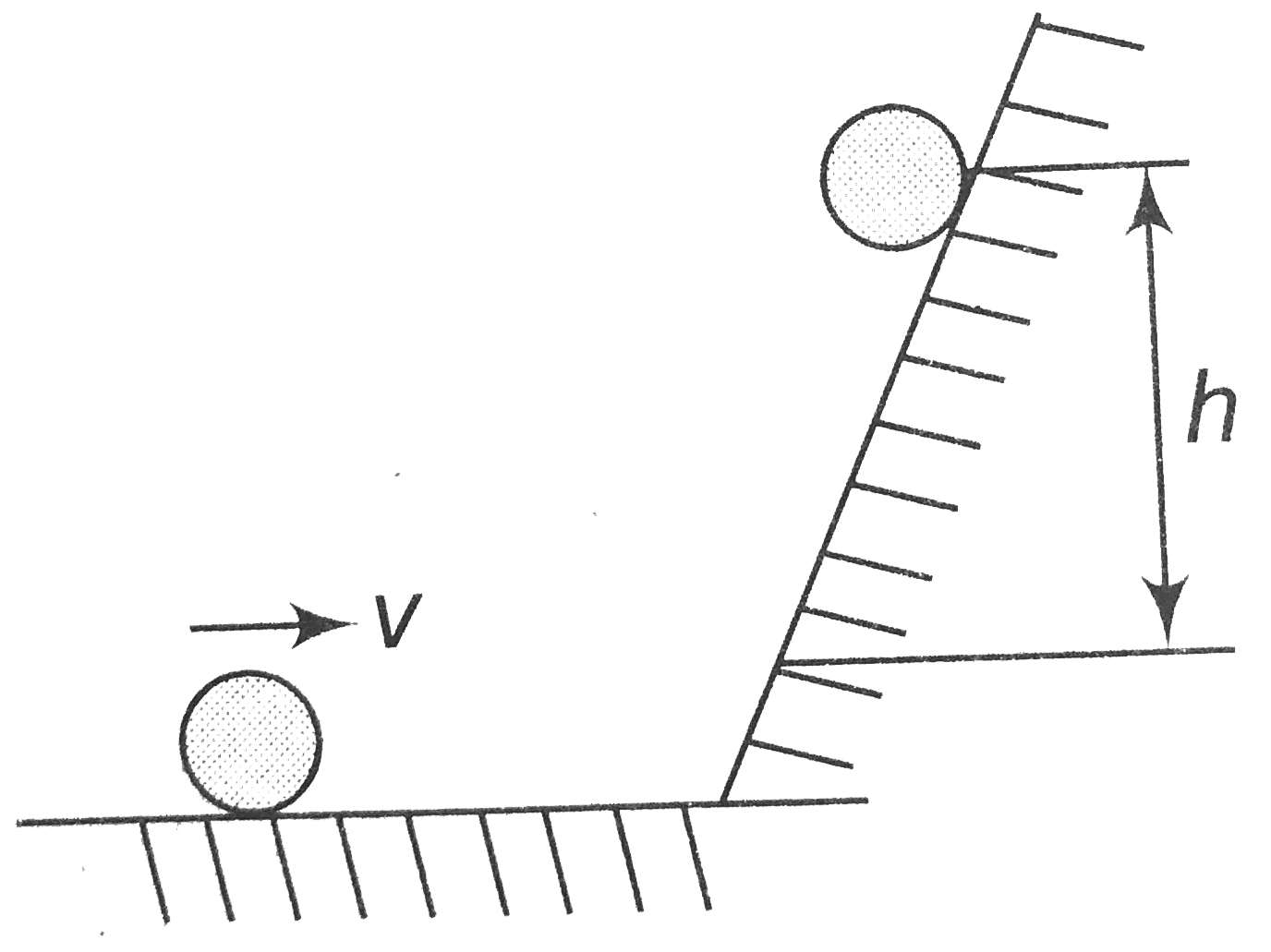A $v^{2} / g$
B $v^{2} / 2 g$
C $v^{2} / 3 g$
D $3 v^{2} / 4 g$
A sphere of mass $m$ and radius $r$ rolls on a horizontal plane without slipping with a speed $u$ . Now it rolls up vertically, then maximum height it would be attain will be
A $\frac{3 u^{2}}{4 g}$
B $\frac{5 u^{2}}{2 g}$
C $\frac{7 u^{2}}{10 g}$
D $\frac{u^{2}}{2 g}$
A sphere of mass m and radius r rolls on a horizontal plane without slipping with the speed u . Now if it rolls up vertically , the maximum height it would attain will be
A $3 u^{2} / 4 g$
B $5 u^{2} / 2 g$
C $7 u^{2} / 10 g$
D $u^{2} / 2 g$