The magnitudes of gravitational fields at distance r1andr2 from the centre of a uniform sphere of radius R are F1andF2 The ratio F1/F2when r1<Randr2<r1 is
r1r2
√r1r2
(r1r2)2
√r2r1
The correct Answer is:A
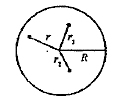
The two points are situated inside the sphere as r1<Randr2<R . Consider a point at distance r .
∴f=G×(Mass of sphere of radius r)r2orF=G×4×π×r3×ρr2×3
or F=(4πGρ3) r = (constant ) r ∴F1F2=r1r2
Similar Questions
The magnitude of the gravitational field at distance r1 and r2 from the centre of a uniform sphere of radius R and mass M are F1 and F2 respectively. Then:
View SolutionThe magnitude of the gravitational field at distance r1andr_2omthecentreofaunifromsphereofradiusRandmassmareF_1 and F_2 respectively. Then:
View Solution
Knowledge Check
The magnitude of the gravitational field at distance r1 and r2 from the centre of a uniform sphere of radius R and mass M are F1 and F2 respectively. Then:
AF1F2=r1r2 if r1>R and r2<RBF1F2=r22r21 if r!>R and r2>RCF1F2=r31r32 if r1<R and r2<RDF1F2=r21r22 if η<R and r2<RThe magnitudes of the gravitational field at distance r1andr2 from the centre of a uniform sphere of radius R and mass M are F1andF2 respectively. Then :
AF1F2=r1r2ifr1<Randr2<RBF1F2=r22r21ifr1>Randr2>RCF1F2=r1r2ifr1>Randr2>RDF1F2=r21r22ifr1<Randr2<RThe magnitudes of the gravitational field at distance r1 and r2 from the centre of a uniform sphere of radius R and mass M are E1 and E2 respectively. Then:
AE1E2=r1r2 if r1<R and r2<RBE1E2=r22r21 if r1<R and r2<RCE1E2=r22r32 if r1<R and r2<RDE1E2=r21r22 if r1<R and r2<R
The magnitude of gravitational field at distances r1 and r2 from the centre of a uniform sphere of radius R and mass M, respectively. Find the ratio of (I1)/(I2)) if r1>R and r2>R.
View SolutionThe magnitude of gravitational field at distances r1 and r2 from the centre of a uniform sphere of radius R and mass M and F1 and F2 respectively. Then,
View SolutionThe magnitude of gravitational field at distances r1 and r2 from the centre of a uniform sphere of radius R and mass M and F1 and F2 respectively. Then,
View SolutionThe magnitude of the gravitational field at distance r1andr_2omthecentreofaunifromsphereofradiusRandmassmareF_1 and F_2 respectively. Then:
View SolutionThe magnitudes of the gravitational field at distance r1 and r2 from the centre of a uniform sphere of radius R and mass M are E1 and E2 respectively. Then:
View Solution
