A tank contains three layers of immiscible liquids. The first layer is of water with refractive index 43 and thickness 8 cm. The second layer is an oil with refractive index 32 and thickness 9 cm while the third layer is of glycerine with refractive index 2 and thickness 4 cm. Find the apparent depth of the bottom of the container.
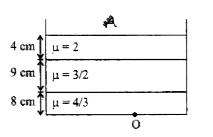
The system now comprises three slabs - one of water, one of oil and one of glycerine. As discussed in this Section and given by equation, the et shift of the system is the sum of the individual shifts in each of the slabs assuming they were in air.
Therefore, Net shift s=t1[1−1μ1]+t2[1−1μ2]+t3[1−1μ3]
s=8[1−34]+9[1−23]+4[1−12]=7cm
The direction of the shift is in the direction of the incident rays which is upwards. Therefore, the apparent depth of the bottom of the container =(4+9+8)−7=14cm
Similar Questions
A tank contains three layers of immiscible liquids. The first layer is of water with refractive index 4/3 and thickness 8cm. The second layer is of oil with refractive index 3/2 and thickness 9cm while the third layer is of glycerine with refractive index 2 and thickness 4cm. Find the apparent depth of the bottom of the container.
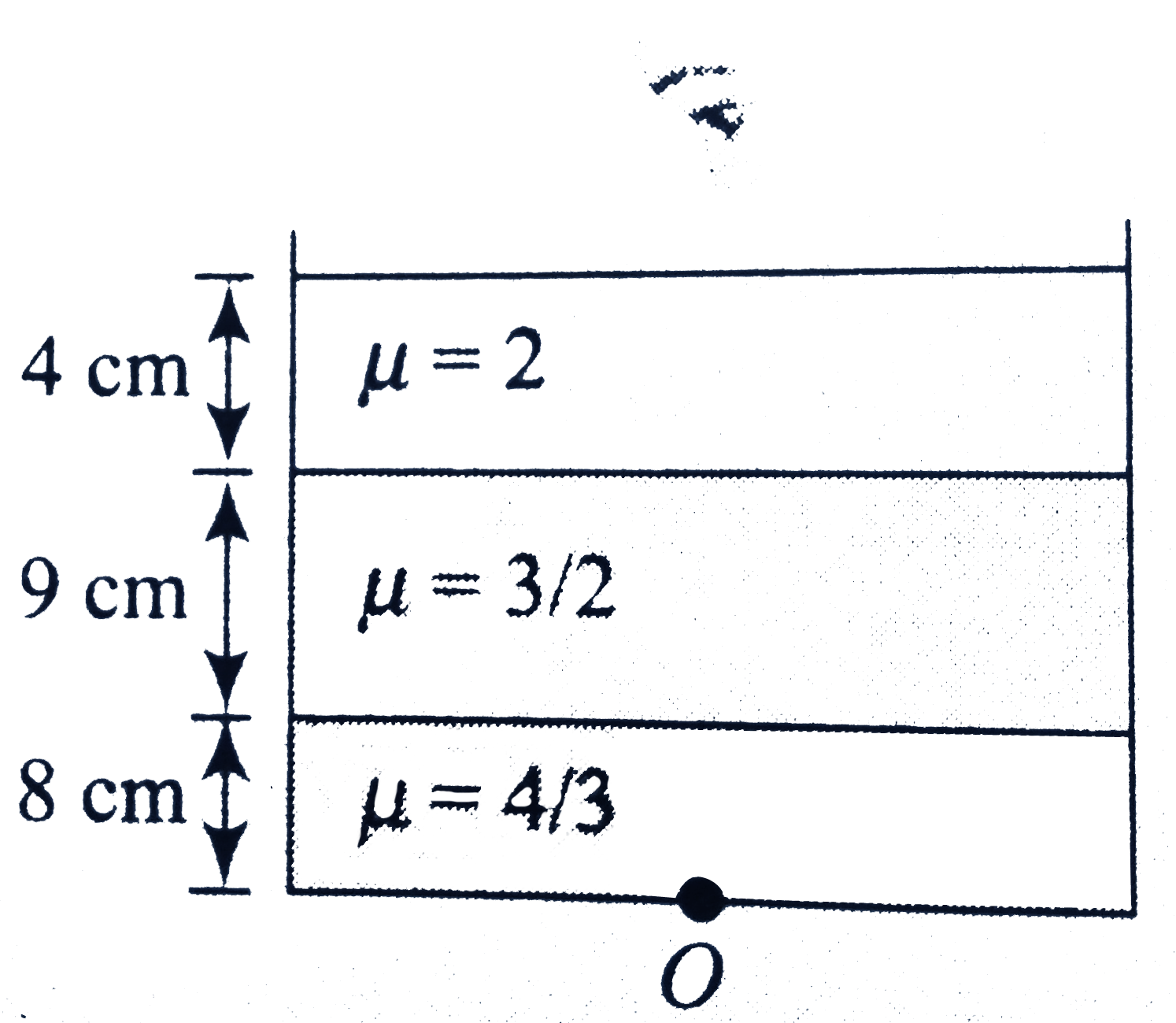
View SolutionA tank contains three layers of immiscible liquids. The first layer is of water with refractive index 4/3 and thickness 8cm. The second layer is of oil with refractive index 3/2 and thickness 9cm while the third layer is of glycerine with refractive index 2 and thickness 4cm. Find the apparent depth of the bottom of the container.

View Solution
Knowledge Check
Refractive index of glass is 32 and the refractive index of water is 43. Then the refractive index of glass with respect to water is
A3/24/3B32×43C32+43D32−43A beaker is filled with two immiscible transparent liquids of refractive indices μ1 and μ2 having thickness of layers d1 and d2 respectively. The apparent depth of the bottom of the beaker is
A(d1×d2d1+d2)((1μ1)+(1μ2))B(μ1×μ2)(d1+d2)C((d1μ2)+(d2μ1))D⎛⎜⎝d1μ1d2μ2⎞⎟⎠A vessel of depth 2d cm is half filled with a liquid of refractive index μ1 and the upper half with a liquid of refractive index μ2. The apparent depth of the vessel seen perpendicularly is
Ad(μ1μ2μ1+μ2)Bd(1μ1+1μ2)C2d(1μ1+1μ2)D2d(1μ1μ2)
A beaker is filled with two immiscible transparent liquids of refractive indices μ1 and μ2 having thickness of layers d1 and d2 respectively. The apparent depth of the bottom of the beaker is
View SolutionFind the refractive index of water with respect to glass if the refractive index of water is 4/3 and the refractive index of glass is 3/2.
View SolutionA pond of depth 20 cm is filled with water of refractive index 4/3. Calculate apparent depth of the tank when viewed normally.
View SolutionRefractive index of water is 4/3 and the refractive index of glass is 3/2. find the refractive index of glass with respect to the refractive index of water.
View SolutionA vessel of depth 2d cm is half filled with a liquid of refractive index μ1 and the upper half with a liquid of refractive index μ2. The apparent depth of the vessel seen perpendicularly is
View Solution
