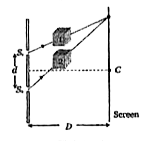
In a Young's double slit experiment, fringes are observed by using light of wavelength 4800 Å, if a glass plate (μ=1.5) is introduced in the path of one of the wave and another plates is introduced in the path of the (μ=1.8) other wave. The central fringe takes the position of fifth bright fringe. The thickness of plate will be
8 micron
80 micron
0.8 micron
4 micron
The correct Answer is:A
Shift due to the first plate x1=βλ(μ1−1)t (upward) br> and shift due to the second x2=βλ(μ2−1)t (downward)
Hence net shift =x2−x1=βλ(μ2−μ1)t⇒5β=βλ(1.8−1.5)t⇒t=5λ0.3=8×10−6m
Similar Questions
In a Young.s double slit experiment, the fringes are displaced by a distance x when a glass plate of refractive index 1.5 is introduced in the path of one of the beams. When this plate is replaced by another plate of same thickness, the shift of fringes is (3/2)x. The refractive index of second plate is
View SolutionA thin sheet of glass (μ=1.520) is introduced normally in the path of one of the two interfering waves. The central bright fringe is observed to shift to position originally occupied by the fifth bright fringe. If λ=5890Å. Find the thickness of the glass sheet.
View Solution
Knowledge Check
- Question 1 - Select One
If a thin transparent plate is introduced, in the path of interfering waves, then entire fringe
Ashifts to leftBshifts to rightCremains sameDshifts upwards. - Question 2 - Select One
In Young's double slit experiment, the fringes are displaced by a distance x when a glass plate of one refractive index 1.5 is introduced in the path of one of the beams. When this plate in replaced by another plate of the same thickness, the shift of fringes is (3/2)x. The refractive index of the second plate is
A1.75B1.5C1.25D1 - Question 3 - Select One
In Young's double slit experiment, the fringes are displaced index 1.5 is introduced in the path of one of the beams. When this plate in replaced by another plate of the same thickness, the shift of fringes is (3/2)x. The refractive index of the second plate is
A(a) 1.75B(b) 1.50C(c) 1.25D(d) 1.00
A thin sheet of glass (μ=1.520) is introduced normally in the path of one of the two interfering waves. The central bright fringe is observed to shift to position originally occupied by the fifth bright fringe. If λ=5890Å. Find the thickness of the glass sheet.
View SolutionIn Young's double slit experiment, the fringes are displaced index 1.5 is introduced in the path of one of the beams. When this plate in replaced by another plate of the same thickness, the shift of fringes is (3/2)x. The refractive index of the second plate is
View SolutionIn Young's double slit experiment the two slits are illuminated with light of wavelength λ .The thickness of a film of refractive index 1.4 to be introduced in the path of one of the interfering beams so that the central image shifts by one fringe width is
View SolutionIn an ideal YDSE when a glass plate (μ=1.5) of thickness t is introduced in the path of one of the interfering beams the intensity at the position where the central maximum occured previously remains unchanged. The maximum thickness of the glass plate is:
View SolutionWhen the mica sheet of thickness 7micros and μ=1.6 is placed in the path of one of interfering beams in the biprism experiment then the central fringe gets at the position of seventh bright fringe.The wavelength of light used will be
View Solution

