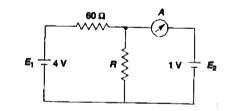
In the circuit shown in Fig, the ammeter A reads zero. If the batteries have negligible internal resistance, the value of R is
10Ω
20Ω
30Ω
40Ω
The correct Answer is:B
Current due to E1 is
I=E1R+60=4R+60 (i)
∴ Potential drop across R = IR. Since there is no current due to E2,
IR=E2=1 ∴=1R. Using this Eq. (i) we get
1R=4R+60, which gives R=20Ω
Similar Questions
In the circuit, the galvanometer G shows zero deflection. If the batteries A and b have negligible internal resistance, the value of the resistor R will be -
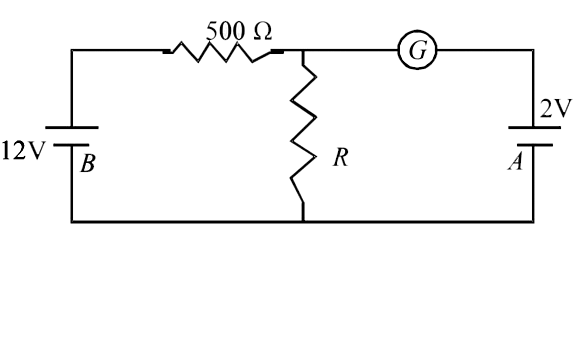
View SolutionIn the circuit the galvanometer G shows zero deflection. If the batteries A and B have negligible internal resistance find the value of the resitor R
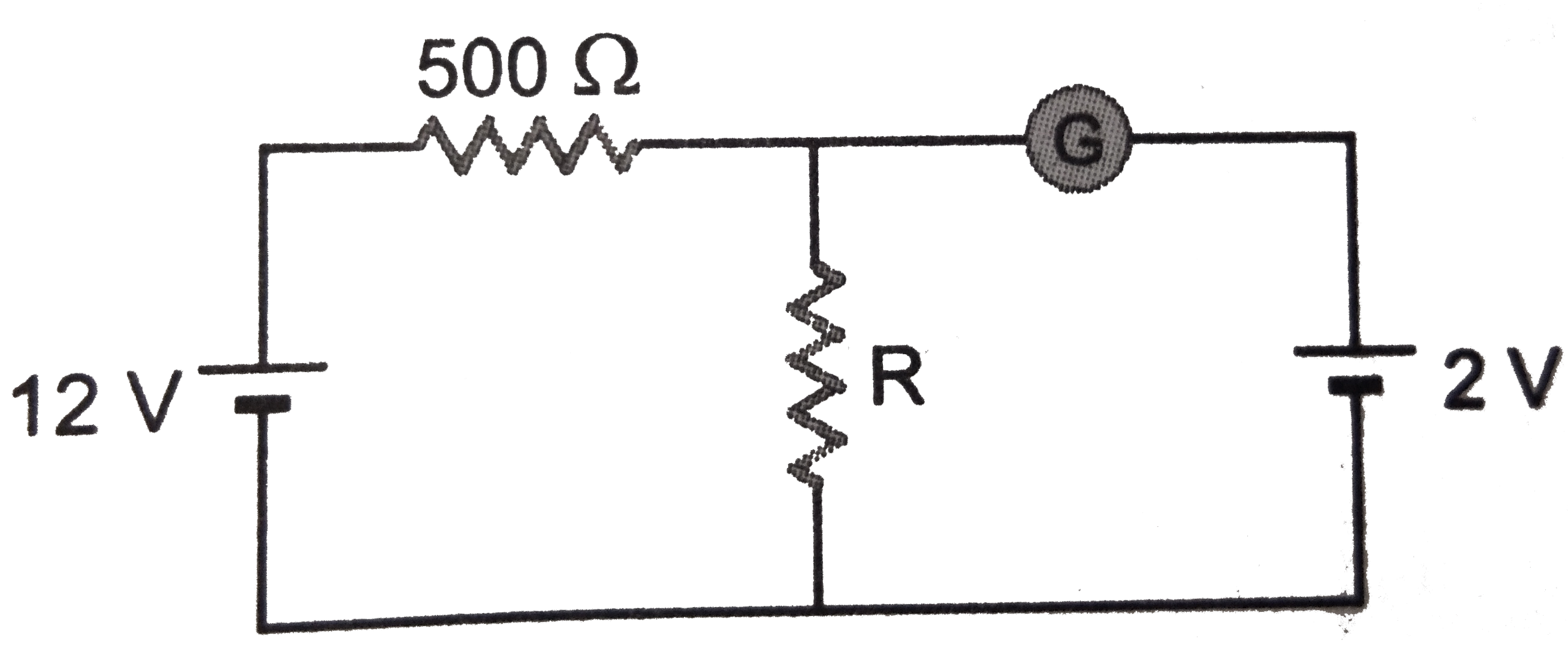
View Solution
Knowledge Check
For the circuit, the galvanometer G shows zero deflection. If the batteries A and B have negligible internal resistance, the value of the resistor R will be
A200ΩB100ΩC500ΩD1000ΩIn the circuit, the galvanometer G shows zero deflection. If the batteries A and B have negligible internal resistance, the value of the resistor R will be
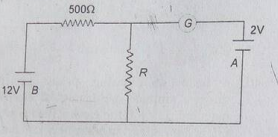A200ΩB100ΩC500ΩD1000ΩIn the circuit, the galvanometer G shows zero deflection. If the batteries A and B have negligible internal resistance, the value of the resistor R will be
A200ΩB100ΩC500ΩD1000Ω
In the circuit shows in Fig. 6.41, the reading of the ammeter is (assume internal resistance of the battery be to zero)
View SolutionIn the circuit shown in Fig.4.20 both the ammeter and the cell have negligible resistance. Three external resistors are identical. When the switch S is opened, the ammeter reads 0.6 A. What will the ammeter read when the switch S is closed ?
View SolutionIn the circuit, the galvanometer G shows zero deflection. If the batteries A and b have negligible internal resistance, the value of the resistor R will be -
View Solution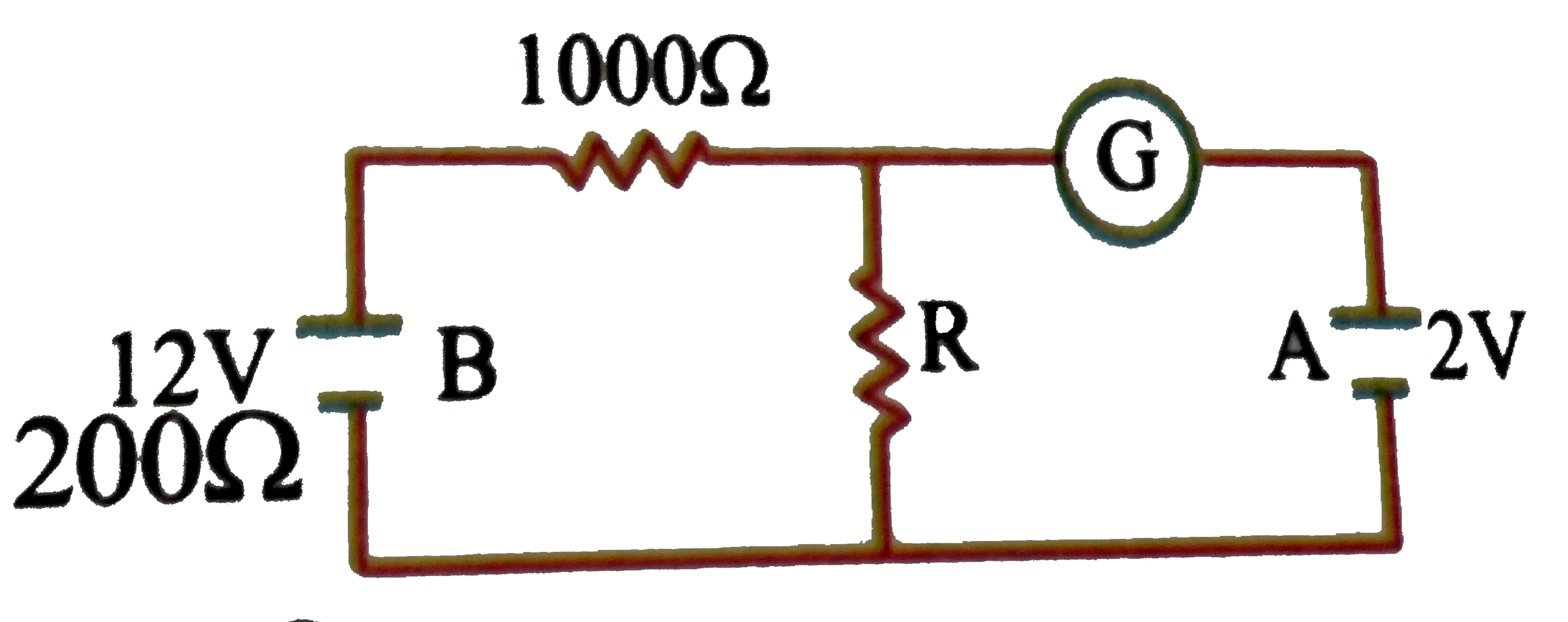
In the circuit the galvanometer G shows zero deflection. If the betteries A and B have negligible internal resistance, the value of the resistor R will be:
View SolutionIn the circuit shows in Fig. 6.41, the reading of the ammeter is (assume internal resistance of the battery be to zero)
View Solution