Assertion : In the given network each resistors is of 2Ω. The resistance across AD is also 2Ω.

Reason : In a balanced Wheatstone bridge no current flows through the diagonal arm.
If both Assertion and Reason are true and Reason is the correct explanation of Assertion.
If both Assertion and Reason are true but Reason is not correct explanation of Assertion.
If Assertion is true but Reason is false.
If both Assertion and Reason are false.
The correct Answer is:A
The network can be redrawn as a wheatstones bridge as shown. As PQ=RS the bridge is balanced.
The 2Ω resistor across BC is an effective. No current flows through it.
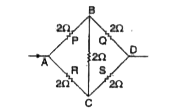
equivalent resistance across
AD=(2+2)(2+2)(2+2)(2+2)=2Ω
Similar Questions
Knowledge Check
In the given circuit diagram if each resistance is of 10Ω, then the current in arm AD will be
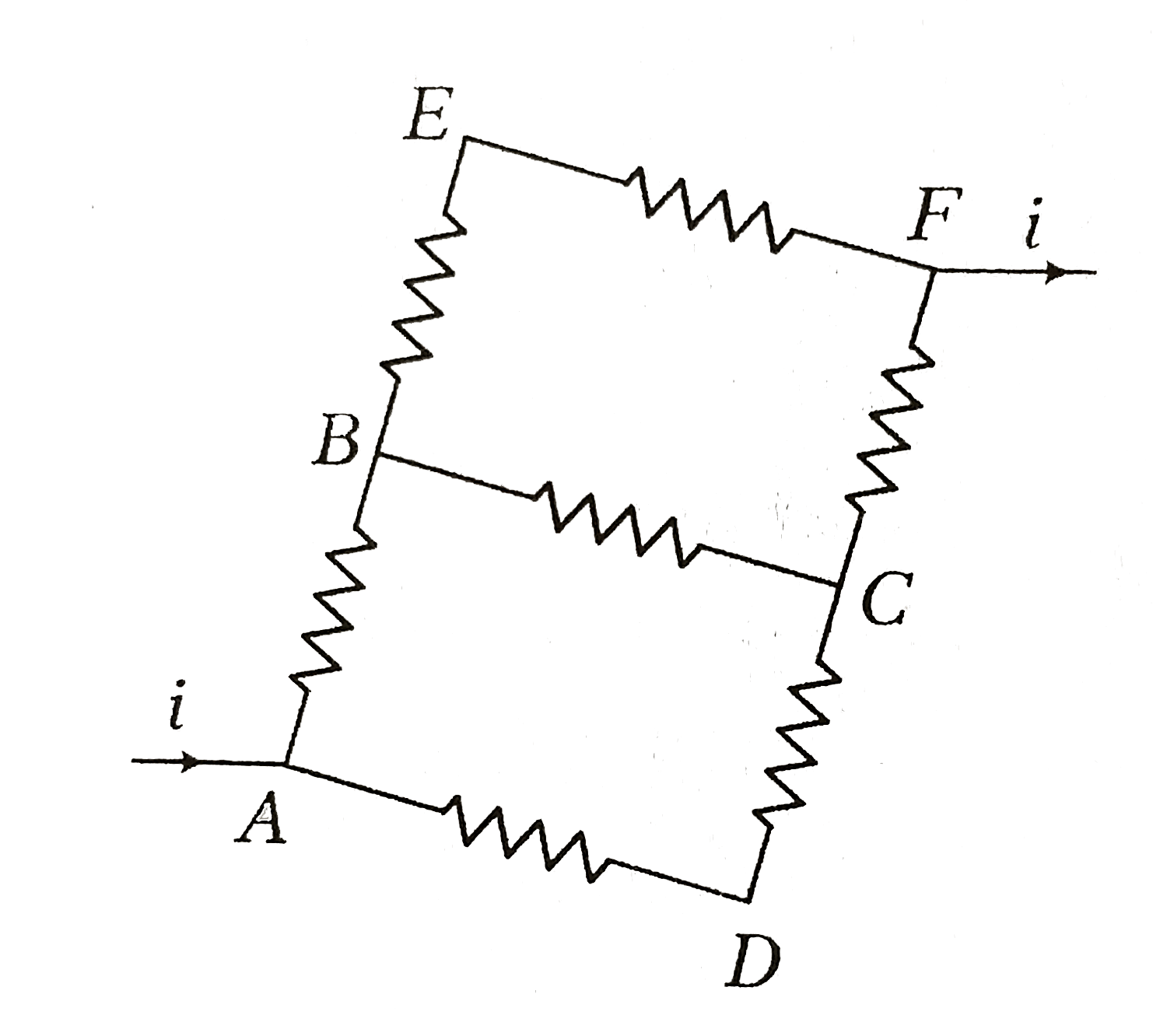 Ai5B2i5C3i5D4i5
Ai5B2i5C3i5D4i5In the shown figure, bridge is balanced, the current flowing through 2Ω resistance is
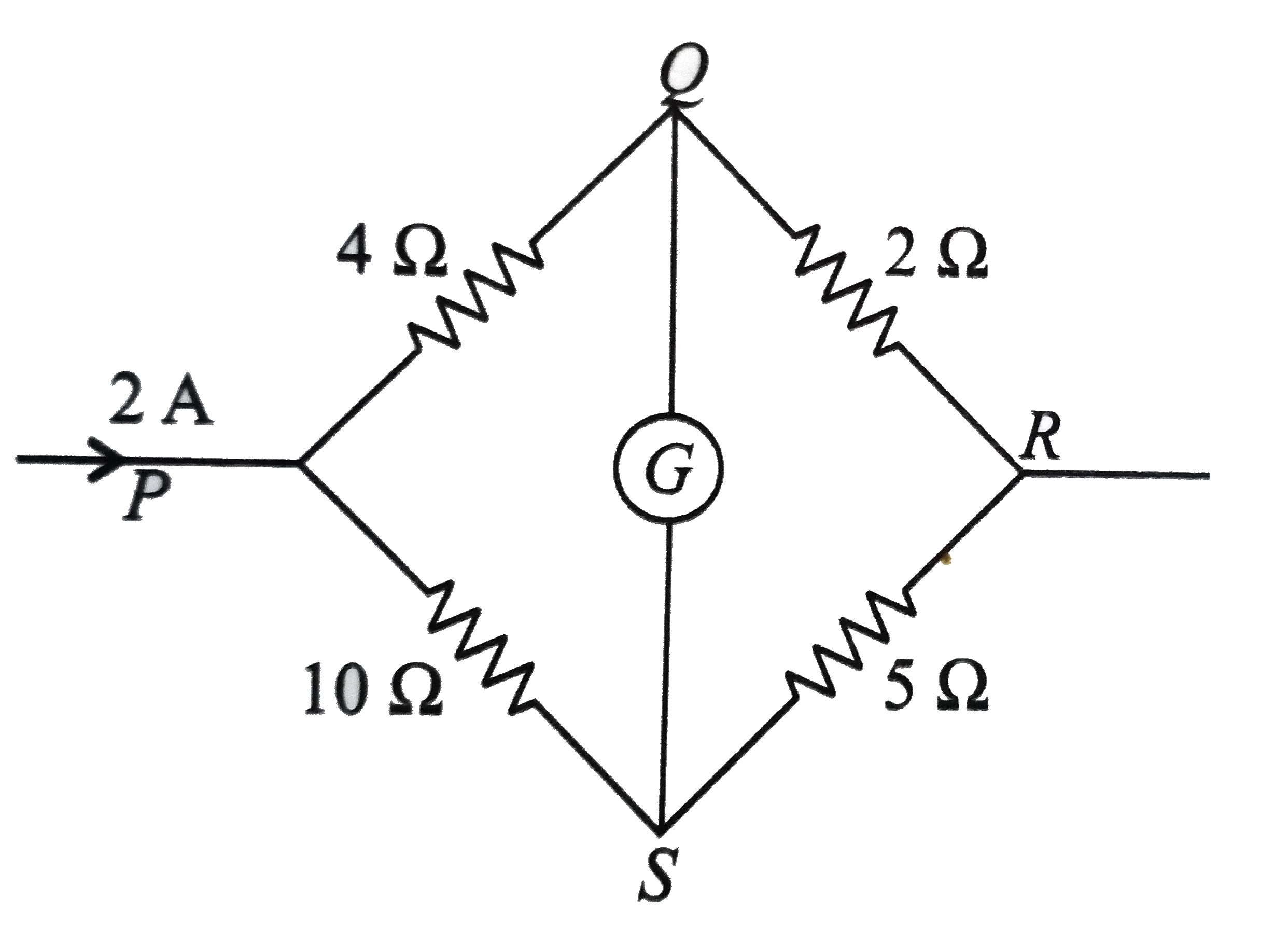 A108AB117AC137AD87A
A108AB117AC137AD87AIn the shown figure, bridge is balanced, the current flowing through 2Ω resistance is
 A107AB117AC137AD87A
A107AB117AC137AD87A
Assertion: When wheatstone bridge is balanced then current through cell depends on resistance of galvanometer.
Reason: At balancec condition current through galvanometer is very high.
View SolutionIn a wheatstone bridge resistance of each of the four sides is 10Ω. If the resistance of the galvanometer is also 10Ω , then effective resistance of the bridge will be
View SolutionAssertion: When wheatstone bridge is balanced then current through cell depends on resistance of galvanometer.
Reason: At balancec condition current through galvanometer is very high.
View SolutionIn the given circuit the resistance of all the resistors is equal to 2Ω, find the current flowing through each resistor under steady state. Select the correct options.
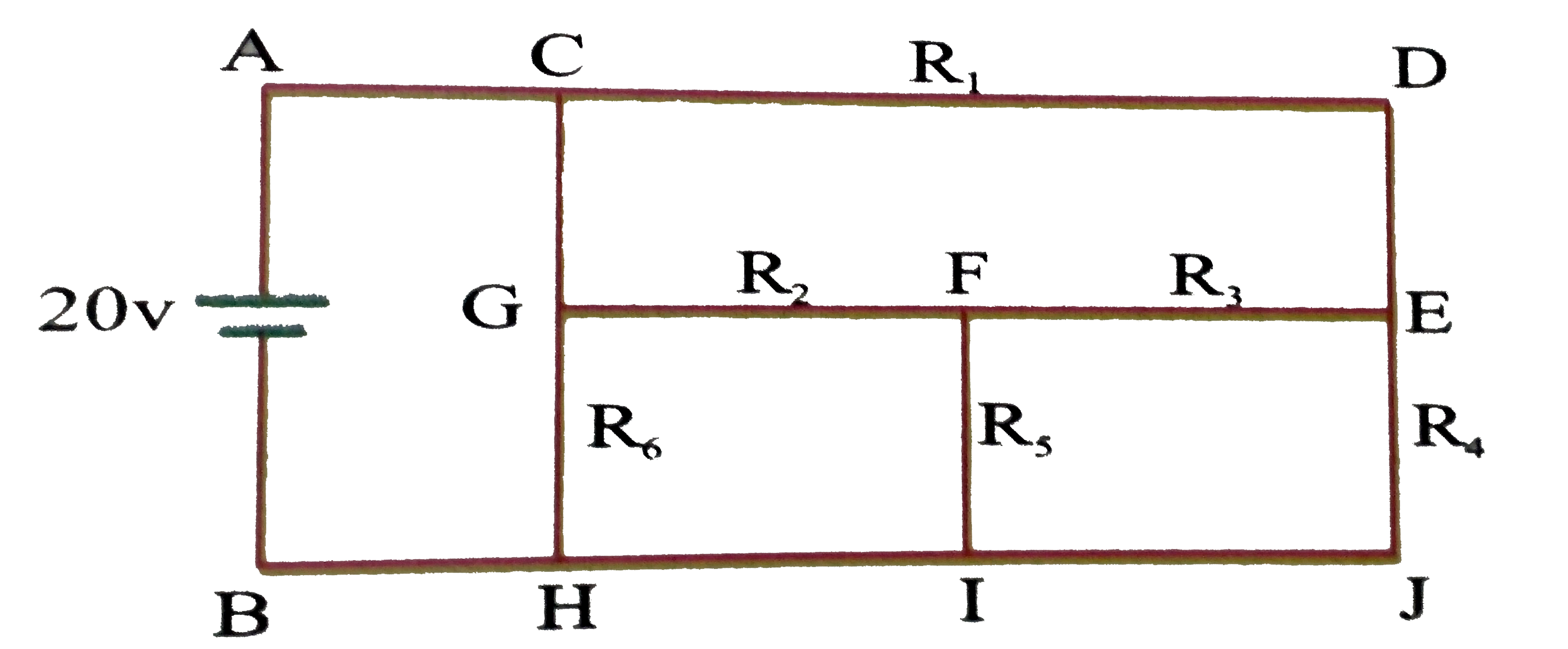
View SolutionIn a wheatstone bridge resistance of each of the four sides is 10Ω. If the resistance of the galvanometer is also 10Ω , then effective resistance of the bridge will be
View Solution
