In a wheatstone bridge resistance of each of the four sides is 10Ω. If the resistance of the galvanometer is also 10Ω , then effective resistance of the bridge will be
10Ω
5Ω
20Ω
40Ω
The correct Answer is:A
To solve the problem of finding the effective resistance of a Wheatstone bridge with given resistances, we can follow these steps:
1. Identify the Resistors:
In a Wheatstone bridge, we have four resistors (R1, R2, R3, R4) and a galvanometer. Given that each resistor has a resistance of 10Ω, we can denote:
- R1 = 10Ω
- R2 = 10Ω
- R3 = 10Ω
- R4 = 10Ω
- Resistance of the galvanometer (Rg) = 10Ω
2. Check for Balance Condition:
The Wheatstone bridge is balanced when the ratio of the resistances is equal:
R1R2=R3R4
Substituting the values:
1010=1010⟹1=1
Since the bridge is balanced, there will be no current flowing through the galvanometer.
3. Combine Resistors in Series:
Since there is no current through the galvanometer, we can treat R1 and R3 as being in series, and R2 and R4 as being in series:
- R_series1 = R1 + R3 = 10Ω + 10Ω = 20Ω
- R_series2 = R2 + R4 = 10Ω + 10Ω = 20Ω
4. Combine Series Resistances in Parallel:
Now, we have two resistances (R_series1 and R_series2) in parallel:
Req=Rseries1×Rseries2Rseries1+Rseries2
Substituting the values:
Req=20Ω×20Ω20Ω+20Ω=400Ω240Ω=10Ω
5. Final Result:
The effective resistance of the Wheatstone bridge is:
Req=10Ω
Conclusion:
The effective resistance of the Wheatstone bridge is 10Ω.
---
In a Wheatstone bridge, we have four resistors (R1, R2, R3, R4) and a galvanometer. Given that each resistor has a resistance of 10Ω, we can denote:
- R1 = 10Ω
- R2 = 10Ω
- R3 = 10Ω
- R4 = 10Ω
- Resistance of the galvanometer (Rg) = 10Ω
The Wheatstone bridge is balanced when the ratio of the resistances is equal:
R1R2=R3R4
Substituting the values:
1010=1010⟹1=1
Since the bridge is balanced, there will be no current flowing through the galvanometer.
Since there is no current through the galvanometer, we can treat R1 and R3 as being in series, and R2 and R4 as being in series:
- R_series1 = R1 + R3 = 10Ω + 10Ω = 20Ω
- R_series2 = R2 + R4 = 10Ω + 10Ω = 20Ω
Now, we have two resistances (R_series1 and R_series2) in parallel:
Req=Rseries1×Rseries2Rseries1+Rseries2
Substituting the values:
Req=20Ω×20Ω20Ω+20Ω=400Ω240Ω=10Ω
The effective resistance of the Wheatstone bridge is:
Req=10Ω
The effective resistance of the Wheatstone bridge is 10Ω.
Similar Questions
In a Wheatstone's brigde all the four arms have equal resistance R. If the resistance of the galvanometer arm is also R, the equivalent resistance of the combination as seen b the battery is
View SolutionFour resistors each of resistance 2 Ω are connected in parallel. What is the effective resistance ?
View Solution
Knowledge Check
In a wheatstone bridge if the battery and galvanometer are interchanged then the deflection in galvanometer will
Achange in previous directionBnot changeCchange in opposite directionDnone of these.
The resistance of each arm of the wheat stone bridge is 10Ω. A resistance of 10Ω is connected in series with galvanometer then the equivalent resistance across the battery will be:-
View SolutionWhat resistance must be connected to a 15 Ω resistance to provide an effective resistance of 6Ω ?
View SolutionIn above question if resistance of meter bridge wire is 1 Ω /cm then the value of current / is
View SolutionThe resistance of the four arms A, B, C and D of a wheatstone bridge as shown in the figure are 10Ω, 20Ω ,30Ω and 60Ω respectively . The emf and internal resistance of the cell are 15V and 2.5Ω respectively . If the galvanometer resistance is 80Ω then the net current drawn from the cell will be
_PHY_E05_111_Q01.png)
View SolutionIn the network shown in figure each resistance is 1Ω . The effective resistance between A and B is

View Solution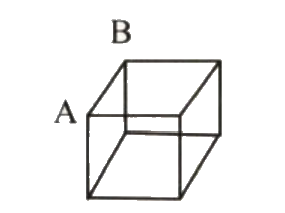
In the above arrangement, each side of the cube has 1Ω resistance and it is known that the effective resistance between A and B is 59Ω. Now, if the resistor between A and B is removed, then find the new effective resistance (in Ω) between the same two points.
View SolutionConsider a Wheatstone bridge with resistance and capacitance connected as shown Find the condition on the resistance and the capacitance such that the bridge remains balanced at all times.
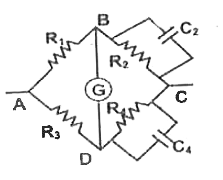
View Solution
