Assertion : Two parallel long wires separated by 2r carry current in the same direction. At P, a point at a distancer from a wire, the magnetic field will always be zero.
Reason : The point P will always be equidistant from the wires.
If both Assertion and Reason are true and Reason is the correct explanation of Assertion.
If both Assertion and Reason are true but Reason is not correct explanation of Assertion
If Assertion is true but Reason is false
If both Assertion and Reason are false.
The correct Answer is:D
Only if the point Pisat a distancer from both the wires, i.e., it lies between the wires will the magnetic field due to each wire cancel provided the current in the wires is equal. The magnitude of the magnetic fields due to the two wires is same but as the directions are opposite the net magnetic field is zero.
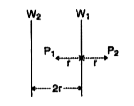
ccording to the statement the point Pis at a distancer from one wire. Therefore, it need not necessarily lie between the two wires. Consider the two positions P1andP2, at a distancer from wire W1. At P1 the magnetic field is zero but at P2 the magnetic field due to current in W1andW2 add up and cannot be zero. The reason is wrong and consequently the assertion too is incorrect.
Similar Questions
Two thin, long, parallel wires, separated by a distance 'd' carry a current of 'i' A in the same direction. They will
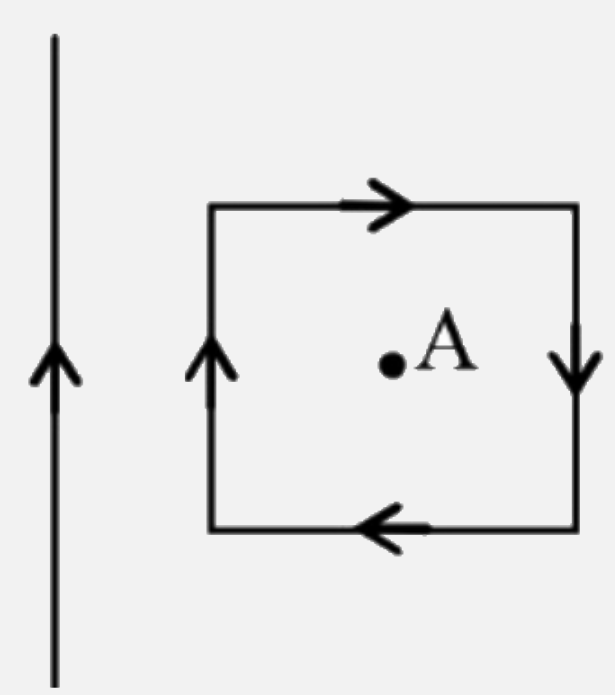
View SolutionA wire is parallel to a square coil . The coil and wire carry currents in the directions Shown . Then, at any point A within the coil the magnetic field will be -
View Solution
Knowledge Check
Two thin, long, parallel wires, separated by a distance 'd' carry a current of 'i' A in the same direction. They will
Arepel each other with a force of μ0i2/(2πd)Battract each other with a force of μ0i2/(2πd)Crepel each other with a force of μ0i2/(2πd2)Dattract each other with a force of μ0i2/(2πd2)Two infinitely long wires each carrying current I along the same direction are made into the geometry as shown in the figure. The magnetic field at the point P is
 Aμ0IπrBμ0Ir(1π+14)CZeroDμ0I2πr
Aμ0IπrBμ0Ir(1π+14)CZeroDμ0I2πrTwo long parallel wires carry equal current I flowing in the same direction are at a distance 2d apart. The magnetic field B at a point lying on the perpendicular line joining the wires and at a distance x from the midpoint is
Aμ0idπ(d2+x2)Bμ0ixπ(d2−x2)Cμ0ix(d2+x2)Dμ0id(d2+x2)
A wire is parallel to a square coil . The coil and wire carry currents in the directions Shown . Then, at any point A within the coil the magnetic field will be -

View SolutionTwo long parallel wires carry equal current I flowing in the same direction are at a distance 2d apart. The magnetic field B at a point lying on the perpendicular line joining the wires and at a distance x from the midpoint is
View SolutionTwo parallel wires carry equal currents of 10A along the same direction and are separated by a distance of 2.0 cm. Find the magnetic field at a point which is 2.0 cm away from each of these wires.
View SolutionTwo parallel wires carry equal currents of 10A along the same direction and are separated by a distance of 2.0 cm. Find the magnetic field at a point which is 2.0 cm away from each of these wires.
View SolutionTwo long parallel wires carry equal current I flowing in the same direction are at a distance 2d apart. The magnetic field B at a point lying on the perpendicular line joining the wires and at a distance x from the midpoint is
View Solution
