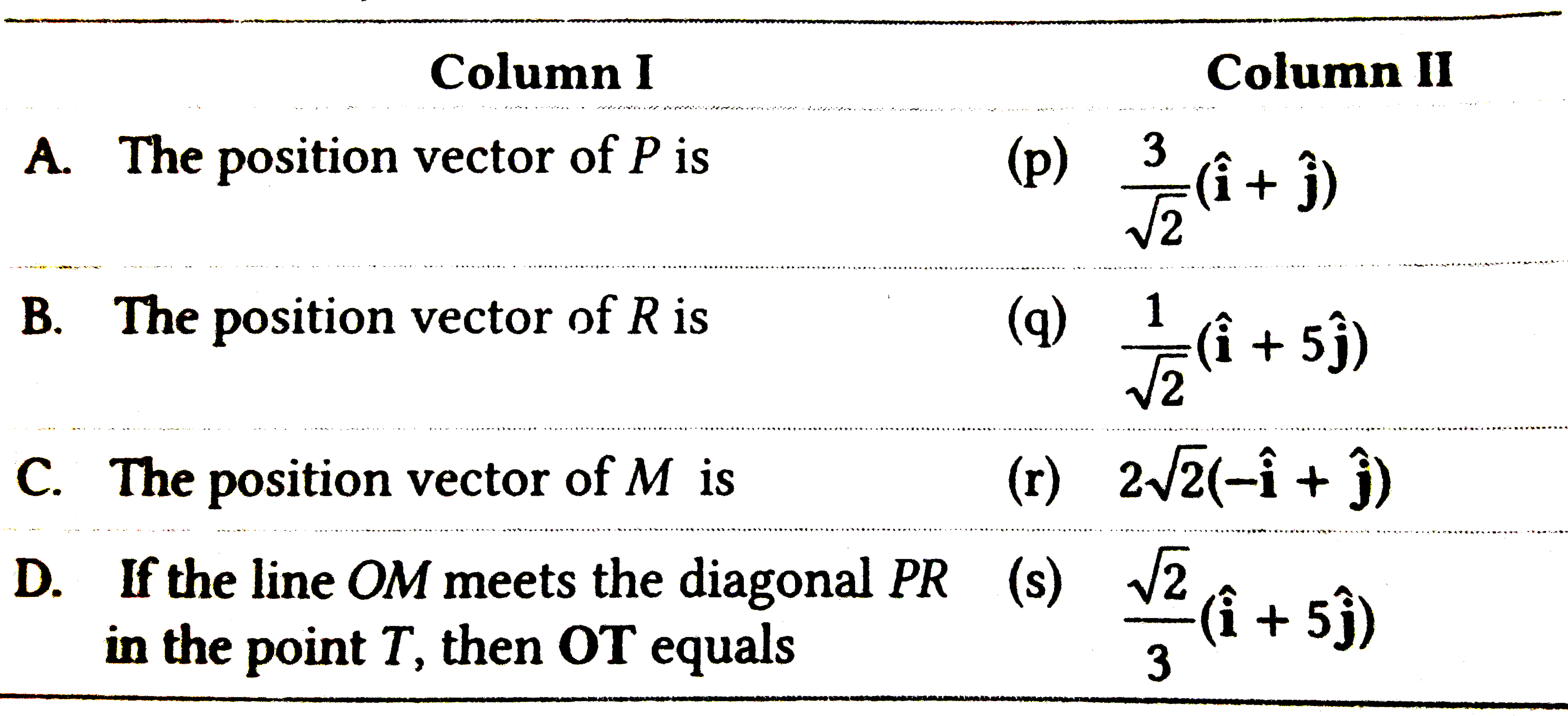
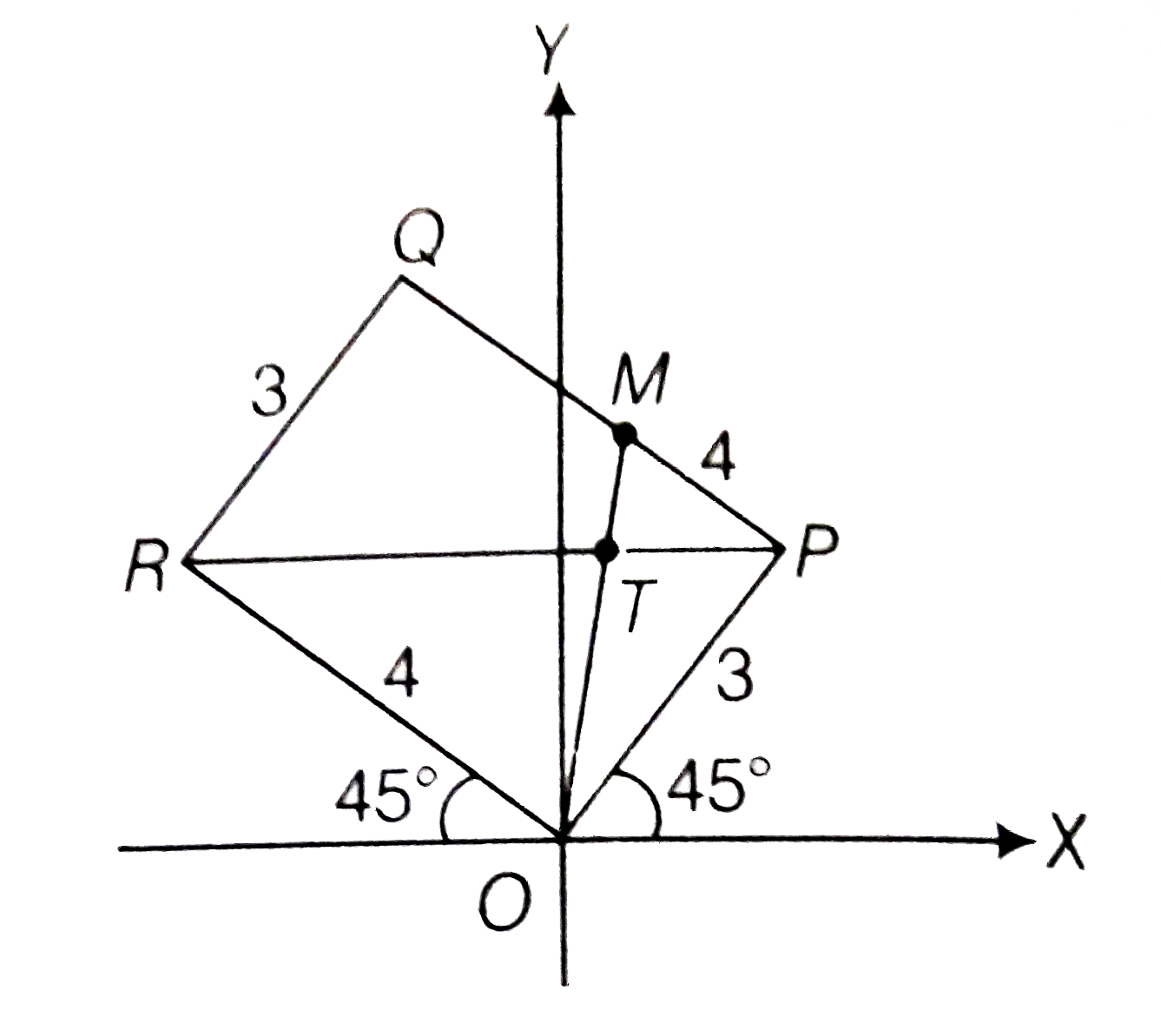
In the Cartesian plane, a man starts at origin and walks a distance of 3 units of the north-east direction and reaches a point P. from P, he walks a distance of 4 units in the north-west direction to reach a point Q. construct the parallelogram OPQR with OP and PQ as adjacent sides. let M be the mid-point of PQ.
Solution in Gujrati

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C, D

(A) Let $ˆ i and ˆ j$ be the unit vectors along OX and OY respectively.
Now, OP=3 and $a \cap g \leq X O P = 45^{\circ}$ implies that
$O P = (3 {cos 45}^{\circ}) ˆ i + (3 {sin 45}^{\circ}) ˆ j = \frac{3}{\sqrt{2}} (ˆ i + ˆ j)$
(B) Again $∠ X O R = 135^{\circ} and O R = 4$ implies that
$O R = \frac{4}{\sqrt{2}} (- ˆ i + ˆ j) = 2 \sqrt{2} (- ˆ i + ˆ j)$
(C) the position vector of Q is given by
$O P + P Q = O P + O R = \frac{1}{\sqrt{2}} (- ˆ i + 7 ˆ j)$

$∴ O M = \frac{(\frac{3}{\sqrt{2}}) (ˆ i + ˆ j) + (\frac{1}{\sqrt{2}}) (- ˆ i + 7 ˆ j)}{2}$
$= \frac{2 ˆ i + 10 ˆ j}{2 \sqrt{2}} = \frac{ˆ i + 5 ˆ j}{\sqrt{2}}$
(D) Now, $P T : R T = 1 : 2$
thereofore, $O T = \frac{1 (O R) + 2 (O P)}{3}$
$= \frac{(\frac{4}{\sqrt{2}}) (- ˆ i + ˆ j) + 2 [(\frac{3}{\sqrt{2}}) (ˆ i + ˆ j)]}{3}$
$= \frac{\sqrt{2}}{3} (ˆ i + 5 ˆ j)$

Updated on:21/07/2023

|

Class 12 MATHS VECTOR ALGEBRA

Similar Questions

Recommended Questions

Home
Ask Doubt
Profile