_S01_083_Q01.png)
Water (density ρ) is flowing through the uniform tube of cross-sectional area A with a constant speed v as shown in the figure. Find the magnitude of force exerted by the water on the curved corner of the tube is (neglect viscous forces)Solution in Gujrati
∣∣¯¯¯¯¯¯ΔPx∣∣=mvsin60∘=√32mv
∣∣¯¯¯¯¯¯ΔPy∣∣=mv2=32mv⇒∣¯¯¯¯¯¯ΔPnet=√ΔP2x+ΔP2y=√94+34mv
∣∣¯¯¯¯¯¯ΔPy∣∣=√3mv⇒∣∣¯¯¯¯¯¯ΔFnet∣∣=√3(dmdt)v=√3ρAv2
(Since, dm=A(vdt)ρ⇒dmdt=Aρv)
_S01_083_S01.png)
Topper's Solved these Questions
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 1 (Elasticity)
16 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 1 (Surface Tension)
21 Videos
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
- ERROR AND MEASUREMENTBOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
Similar Questions
Which one of the following substances possesses the highest elasticity-
View SolutionThe length of needle foating on the surface of water is 1.5 cm the force in addition to its weight required to lift the needle from water surface will be (surface tension of water =7.5N/cm)
View SolutionTwo particles of masses m1 and m2 in projectile motion have velocities →v1 and →v2 , respectively , at time t=0. They collide at time t0. Their velocities become →v'1 and →v'2 at time 2t0 while still moving in air. The value of ∣∣∣(m1→v'1+m2→v'2)−(m1→v1+m2→v2)∣∣∣
View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
View SolutionWater flows through the tube shown in figure. The areas of cross section of the wide and the narrow portions of the tube are 5cm2and2cm2 respectively. The rate of flow of water through the tube is 500cm3s−1 . Find the difference of mercury levels in the U-tube.

View SolutionA horizontal tube of uniform cross-sectional area A is bent in the form of U as shown in figure. If the liquid of density ρ enters and leaves the tube with velcity v, then the extermal force F requried to hold the bend stationary is

View SolutionA liquid of density rho is flowing with a speed v through a pipe of cross sectional area A. The pipe is bent in the shape of a right angles as shown. What force should be exerted on the pipe at the corner to keep it fixed?
View SolutionThe tube shown in figure is of unifrom corss-section. Liquid flows through it at a constant speed in the direction shown by arrows. Then the liquid exerts on the tube is:
View SolutionA liquid of density ρ comes out with a velocity v from a horizontal tube of area of cross-section A. The reaction force exerted by the liquid on the tube is F. Choose the incorrect option.
View Solution_S01_083_Q01.png)
Water (density ρ) is flowing through the uniform tube of cross-sectional area A with a constant speed v as shown in the figure. Find the magnitude of force exerted by the water on the curved corner of the tube is (neglect viscous forces)
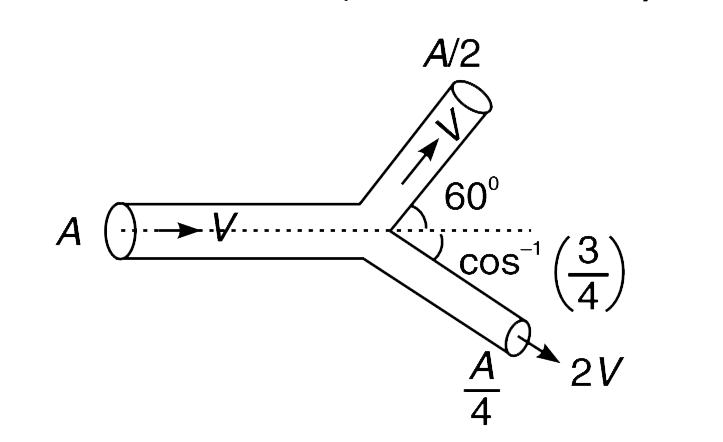
View SolutionWater flows through a tube assembly as shown in the fig. Speed of flow (marked as Vand2V), cross sectional area (A, A/2 and A/4) and the angles between segments has been shown in fig. Calculate the force applied by the water flow on the tube. Take density of water to be ρ.
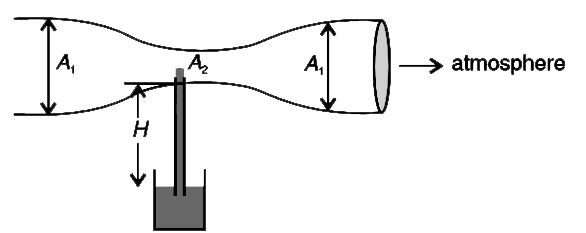
View Solution(i) Air (density=ρ) flows through a horizontal venturi tube that discharges to the atmosphere. The area of cross section of the tube is A1 and at the constriction it is A2. The constriction is connected to a water (density=ρ0) tank through a vertical pipe of lenght H. Find the volume flow rate (Q) of the air through tube that is needed to just draw the water into the tube.
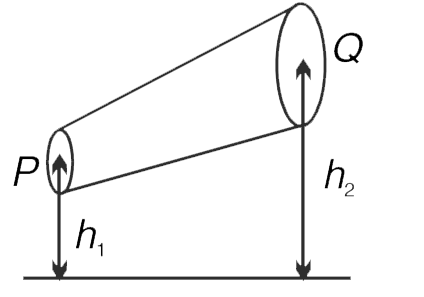
(ii) A non viscous liquid of constant density ρ flows in a stremline motion along a tube of variable cross section. The tube is kept inclined in the vertical plane as shown in the figure. The area of cross section of the tube at two points P and Q at heights of h1 and h2 are respectively A1 and A2. The velocity of the liquid at point P is v. Find the work done on a small volume ΔV of fluid by the neighbouring fluid as the small volume moves from P to Q.
View SolutionWater flows through a pipe bent at an angle alpha to the horizontal with velocity v . Calculate the force exerted by the water on the bend of the pipe of area of cross - section S .
View SolutionA liquid of density rho comes out with a velocity v from a horizontal tube of area of cross-section A. The reaction force exerted by the liquid on the tube is F :
View SolutionThe figure bwlow indicates flow of water through a tube of uniform cross-section with a constant speed in the direction as shown by the arrows. On the tube, water exerts
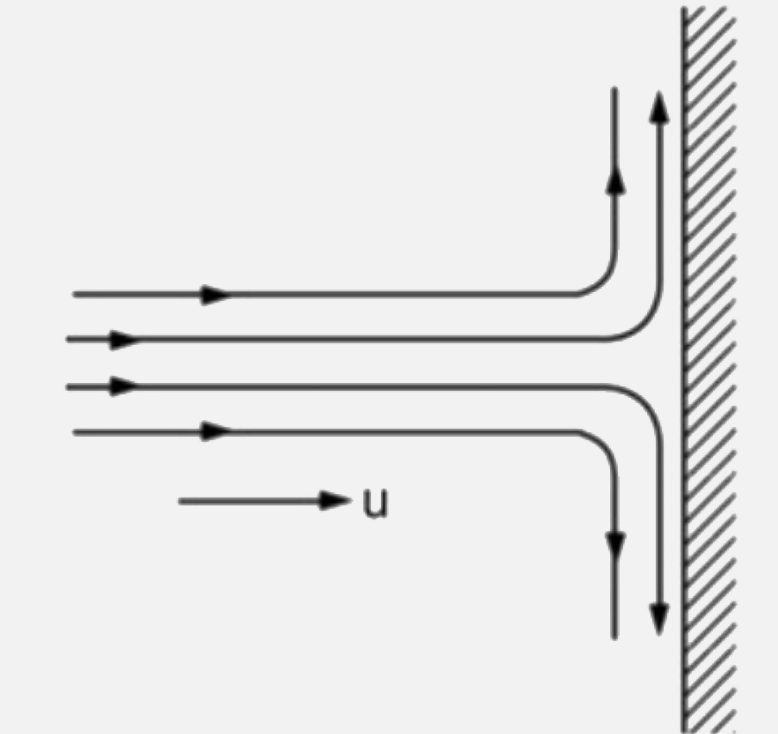
View SolutionA stream of water of density ρ, cross sectional area A, and speed u strikes a wall that is perpendicular to the direction of the stream, as shown in the figure below. The water then flows sideways across the wall. The force exerted by the stream on the wall is
View Solution
