A wooden cylinder of diameter 4 r, height H and density ρ/3 is kept on a hole of diameter 2 r of a tank, filled with water of density ρ as shown in the
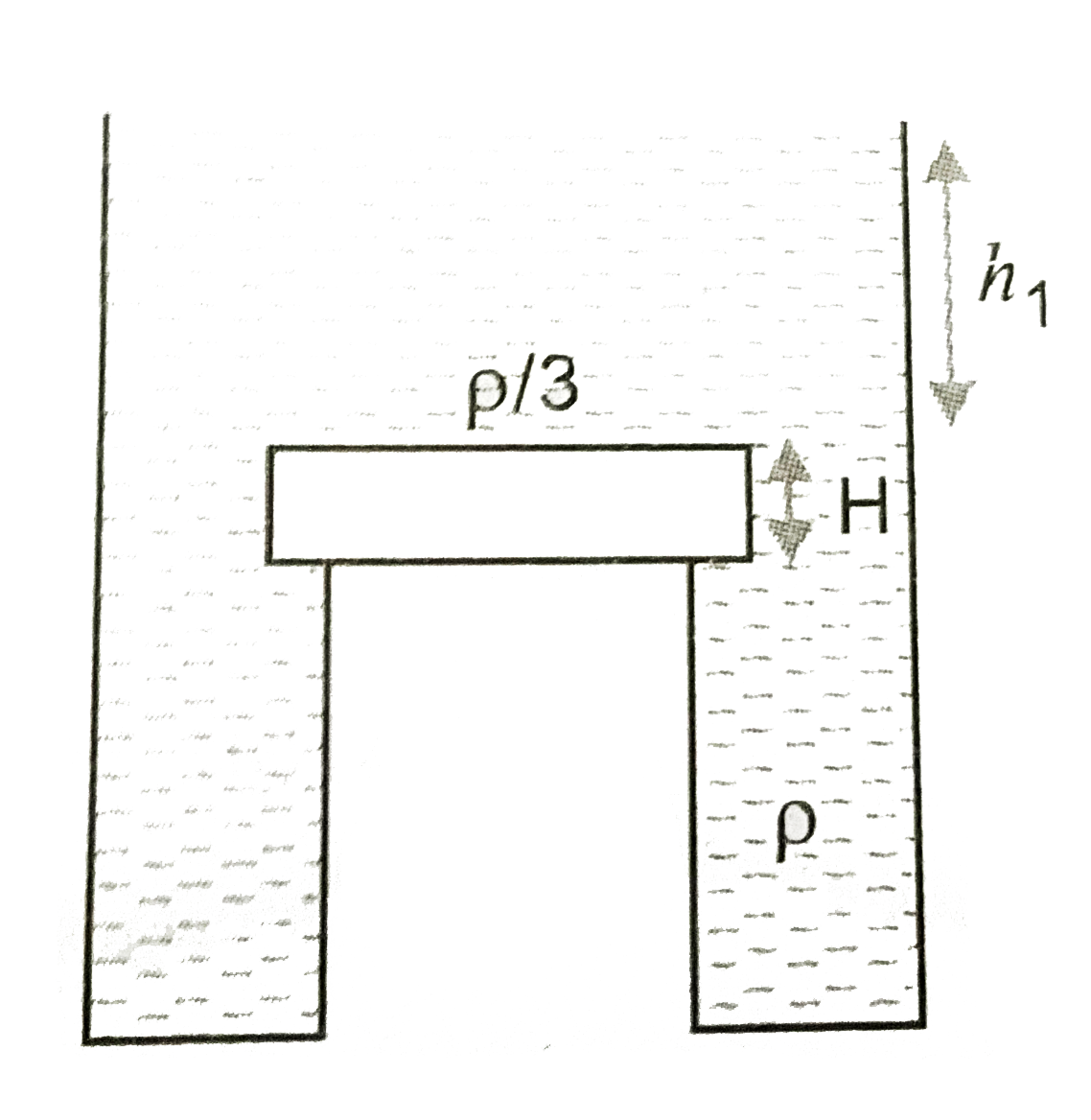
If level of liquid starts decreasing slowly, when the level of liquid is at a height h1 above the cylinder, the block just start moving up. Then the value of h1 is Solution in Gujrati
4h9
5h9
5h3
remains same
The correct Answer is:C
Step by step video & image solution for A wooden cylinder of diameter 4 r, height H and density rho//3 is kept on a hole of diameter 2 r of a tank, filled with water of density rho as shown in the If level of liquid starts decreasing slowly, when the level of liquid is at a height h_(1) above the cylinder, the block just start moving up. Then the value of h_(1) is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Assertion Reason)
1 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Subjective Type Questions)
7 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Multiple correct answers)
2 Videos
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
- ERROR AND MEASUREMENTBOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
Similar Questions
STATEMENT-1: The stream of water flowing at high speed from a garden hose pipe tends to spread like a fountain when held vertically up, but tends to narrow down when held vertically down.
STATEMENT-2: In any steady flow of an incompressible fluid, the volume flow rate of the fluid remains constant.
View SolutionA wooden stick of length L, radius R and density ρ has a small metal piece of mass m ( of negligible volume) attached to its one end. Find the minimum value for the mass m (in terms of given parameters) that would make the stick float vertically in equilibrium in a liquid of density σ(>ρ).
View SolutionA solid sphere of radius R and density ρ is attached to one end of a mass-less spring of force constant k. The other end of the spring is connected to another solid sphere of radius R and density 3ρ. The complete arrangement is placed in a liquid of density 2ρ and is allowed to reach equilibrium. The correct statements(s) is (are)
View SolutionTwo particles of masses m1 and m2 in projectile motion have velocities →v1 and →v2 , respectively , at time t=0. They collide at time t0. Their velocities become →v'1 and →v'2 at time 2t0 while still moving in air. The value of ∣∣∣(m1→v'1+m2→v'2)−(m1→v1+m2→v2)∣∣∣
View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
View SolutionA cylindrical tank has a hole of diameter 2r in its bottom. The hole is covered wooden cylindrical block of diameter 4r, height h and density ρ/3.

Situation I: Initially, the tank is filled with water of density ρ to a height such that the height of water above the top of the block is h1 (measured from the top of the block).
Situation II: The water is removed from the tank to a height h2 (measured from the bottom of the block), as shown in the figure. The height h2 is smaller than h (height of the block) and thus the block is exposed to the atmosphere.
Find the minimum value of height h1 (in situation 1), for which the block just starts to move up?
View SolutionA cylindrical tank has a hole of diameter 2r in its bottom. The hole is covered wooden cylindrical block of diameter 4r, height h and density ρ/3.

Situation I: Initially, the tank is filled with water of density ρ to a height such that the height of water above the top of the block is h1 (measured from the top of the block).
Situation II: The water is removed from the tank to a height h2 (measured from the bottom of the block), as shown in the figure. The height h2 is smaller than h (height of the block) and thus the block is exposed to the atmosphere.
Find the height of the water level h2 (in situation 2), for which the block remains in its origin) position whithout the application of any external force
View SolutionA cylindrical tank has a hole of diameter 2r in its bottom. The hole is covered wooden cylindrical block of diameter 4r, height h and density ρ/3.

Situation I: Initially, the tank is filled with water of density ρ to a height such that the height of water above the top of the block is h1 (measured from the top of the block).
Situation II: The water is removed from the tank to a height h2 (measured from the bottom of the block), as shown in the figure. The height h2 is smaller than h (height of the block) and thus the block is exposed to the atmosphere.
In situation 2, if h2 is further decreased, then
View SolutionA wooden cylinder of diameter 4 r, height H and density rho//3 is kept on a hole of diameter 2 r of a tank, filled with water of density rho as shown in the The block in the above question is maintained by external means and the level of liquid is lowered. The height h_(2) when this external force reduces to zero is
View SolutionA wooden cylinder of diameter 4 r, height H and density ρ/3 is kept on a hole of diameter 2 r of a tank, filled with water of density ρ as shown in the

If height h2 of water level is further decreased, then
View SolutionA cylindrical tank has a hole of diameter 2r in its bottom. The hole is covered wooden cylindrical block of diameter 4r, height h and density ρ/3.

Situation I: Initially, the tank is filled with water of density ρ to a height such that the height of water above the top of the block is h1 (measured from the top of the block).
Situation II: The water is removed from the tank to a height h2 (measured from the bottom of the block), as shown in the figure. The height h2 is smaller than h (height of the block) and thus the block is exposed to the atmosphere.
Find the height of the water level h2 (in situation 2), for which the block remains in its origin) position whithout the application of any external force
View Solutionएक लकड़ी का बेलन जिसका व्यास 4r, ऊंचाई h तथा घनत्व ρ/3 है। एक 2r व्यास की टंकी वाले छिद्र पर रखा है। टंकी ρ घनत्व वाले द्रव से भरी है।
द्रव का स्तर धीरे-धीरे नीचे गिरने लगता है जब बेलन के ऊपर द्रव स्तर की ऊँचाई h1 है, तब ब्लॉक ऊपर गति करना प्रारम्भ करता है। h1 के किस मान पर ब्लॉक उठेगा?
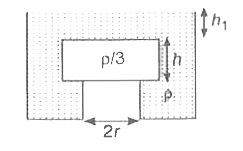
View Solutionएक लकड़ी का बेलन जिसका व्यास 4r, ऊंचाई h तथा घनत्व ρ/3 है। एक 2r व्यास की टंकी वाले छिद्र पर रखा है। टंकी ρ घनत्व वाले द्रव से भरी है।
उपरोक्त प्रश्न में ब्लॉक बाह्य बलों के द्वारा अपनी स्थिति में बना रहता है तथा द्रव का स्तर नीचे हो जाता है। जब यह बाह्य बल शून्य हो जाता है, तब ऊँचाई h2 का मान है
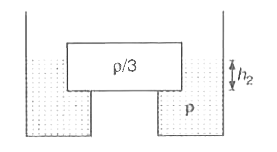
View SolutionA wooden cylindrical of diameter 4r, height h and density ρ/3 is kept on a hole of diameter 2r of a tank , filled with water of density ρ as shown in the figure . The height of the base of cylinder from the base of tank is H .
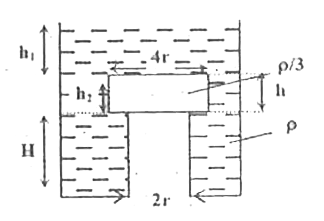
Let the cylinder is prevented from moving up , by applying a force and water level is further decreased . Then , height of water level (h2 in figure ) for which the cylinder remains in original position without application of force is
View SolutionA wooden ball of density sigma is released from the bottom of a tank which is filled with a liquid of density rho (rho>sigma) up to a height h_(1) . The ball rises in the liquid, emerges from its surface and attains a height h_(2) in air.If viscous effects are neglected,the ratio h_(2)/h_(1) is
View Solutionएक टंकी के तल में 2r व्यास का एक बेलनाकार छिद्र H है ( चित्रानुसार)। इस छिद्र से द्रव के बहाव रोकने के लिए इसके ऊपर 4r व्यास h ऊंचाई का एक बेलनाकार ब्लॉक B रखा जाता है। ब्लॉक की सतह से h1 ऊंचाई तक द्रव भरा हुआ है। द्रव का घनत्व ρ व् ब्लॉक का घनत्व ρ/3 है।
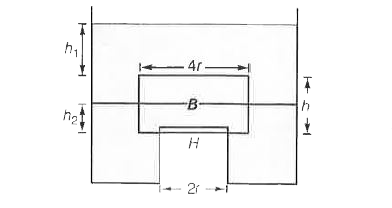
यदि द्रव की सतह को ओर कम किया जाता है। ब्लॉक की निचली सतह से द्रव के तल की दुरी h2 हो तो h2 के वह अधिकतम मान जिस पर ब्लॉक पाने स्थान से न हिले
View Solution
