A metallic rod of length 1m has one end free and other end rigidly clamped. Longitudinal stationary waves are set up in the rod in such a way that there are total six antinodes present along the rod. The amplitude of an antinode is 4×10−6m. young's modulus and density of the rod are 6.4×1010N/m2 and 4×103Kg/m3 respectively. Consider the free end to be at origin and at t=0 particles at free end are at positive extreme.
The magnitude of strain at midpoint of the rod at t=1sec isSolution in Gujrati
11√3π×10−6
11√2π×10−6
10√3π×10−6
10√2π×10−6
The correct Answer is:B
Speed of wave v=√yρ=3×103 _S01_024_S01.png) λ=5λ2+λ4⇒λ=4l11
λ=5λ2+λ4⇒λ=4l11
Frequency v=vλ=4×103411×1=11×103Hz, Wave Number K=2πλ=11π2
(i) Equation of standing wave in the rod S=Acoskxsin(ωt+ϕ) where A=4×10−6m
∵ at x=0,t=0⇒S=A⇒A=A⇒cosk(0)sinϕ=1⇒ϕ=π2
S=4×10−6cos(11π2x)cos(22π×103t)
(ii) Strain =dsdx=−22π×10−6sin(11π2x)cos(22π×103t)∵stress=Y×strain
⇒stress=140.8×104cos(22π×103t)sin(11π2x+π)
(iii) Strain at t=1s and x=l2=12m,∣∣∣dsdx∣∣∣t=1x=l2=22π×10−6×sin(11π4)=11√2π×10−6.
Topper's Solved these Questions
Similar Questions
The length , breadth , and thickness of a metal sheet are 4.234m,1.005m,and2.01cm, respectively. Give the area and volume of the sheet to the correct number of significant figures.
View SolutionThe energy of a photon of sodium light (λ=589nm)equal the band gap of a semiconducting material.(a)Find the minimum energy E requried to create a hole-electron pair.(b)Find the value of E/kTat a temperature of 300K.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionA steel rod 2.5 m long is rigidly clamped at its centre C and longitudinal waves are set up on both sides of C by rubbing along the rod . Young's modulus for steel = 2 xx 10^(11) N//m^(2) , density of steel = 8000 kg//m^(3) If two antinodes are observed on either side of C , the frequency of the node in which the rod is vibrating will be
View SolutionA steel rod 2.5m long is rigidly clamped at its centre C and longitudinal waves are set up on both sides of C by rubbing along the rod . Young's modulus for steel =2×1011N/m2 , density of steel =8000kg/m3

If the amplitude of the wave at the antinode , when it is vibrating in its fundamental mode is 2×10−6m , the maximum velocity of a steel particle in its vibration is
View SolutionA steel rod 2.5m long is rigidly clamped at its centre C and longitudinal waves are set up on both sides of C by rubbing along the rod . Young's modulus for steel =2×1011N/m2 , density of steel =8000kg/m3

If the clamp of the rod be shifted to its end A and totally four antinodes are observed in the rod when longitudinal waves are set up in it , the frequency of vibration of the rod in this mode is
View SolutionA metallic rod of length 1m has one end free and other end rigidly clamped. Longitudinal stationary waves are set up in the rod in such a way that there are total six antinodes present along the rod. The amplitude of an antinode is 4×10−6m. Young's modulus and density of the rod are 6.4×1010N/m2 and 4×103Kg/m3 respectively. Consider the free end to be at origin and at t=0 particles at free end are at positive extreme.
The equation describing displacements of particles about their mean positions is
View SolutionA metallic rod of length 1m has one end free and other end rigidly clamped. Longitudinal stationary waves are set up in the rod in such a way that there are total six antinodes present along the rod. The amplitude of an antinode is 4×10−6m. young's modulus and density of the rod are 6.4×1010N/m2 and 4×103Kg/m3 respectively. Consider the free end to be at origin and at t=0 particles at free end are at positive extreme.
The equation describing stress developed in the rod is
View SolutionA metallic rod of length 1m is rigidly clamped at its mid point. Longirudinal stationary wave are setup in the rod in such a way that there are two nodes on either side of the midpoint. The amplitude of an antinode is 2 xx 10^(-6) m . Write the equation of motion of a point 2 cm from the midpoint and those of the constituent waves in the rod, (Young,s modulus of the material of the rod = 2 xx 10^(11) Nm^(-2) , density = 8000 kg-m^(-3) ). Both ends are free.
View SolutionA metallic rod of length 1m is rigidly clamped at its end points. Longitudinal stationary waves are setup in the ord in such a way that there six antinodes of displacement wave observed along the rod. The amplitude of the anotinode is 2×10−6m. Write the equations of the stationary wave and the component waves at the point 0.1m from the one end of the rod.
[Young modulus =7.5×1010N/m2, density =2500kg/m3]
View SolutionA thin rod of length 3 m is being rotated with 10 rad/sec about its one end. The change in length of the rod due to rotation is (Given : Young's modulus of elasticity of the wire, Y=3×108SI unit, density of the rod is 104 Unit)
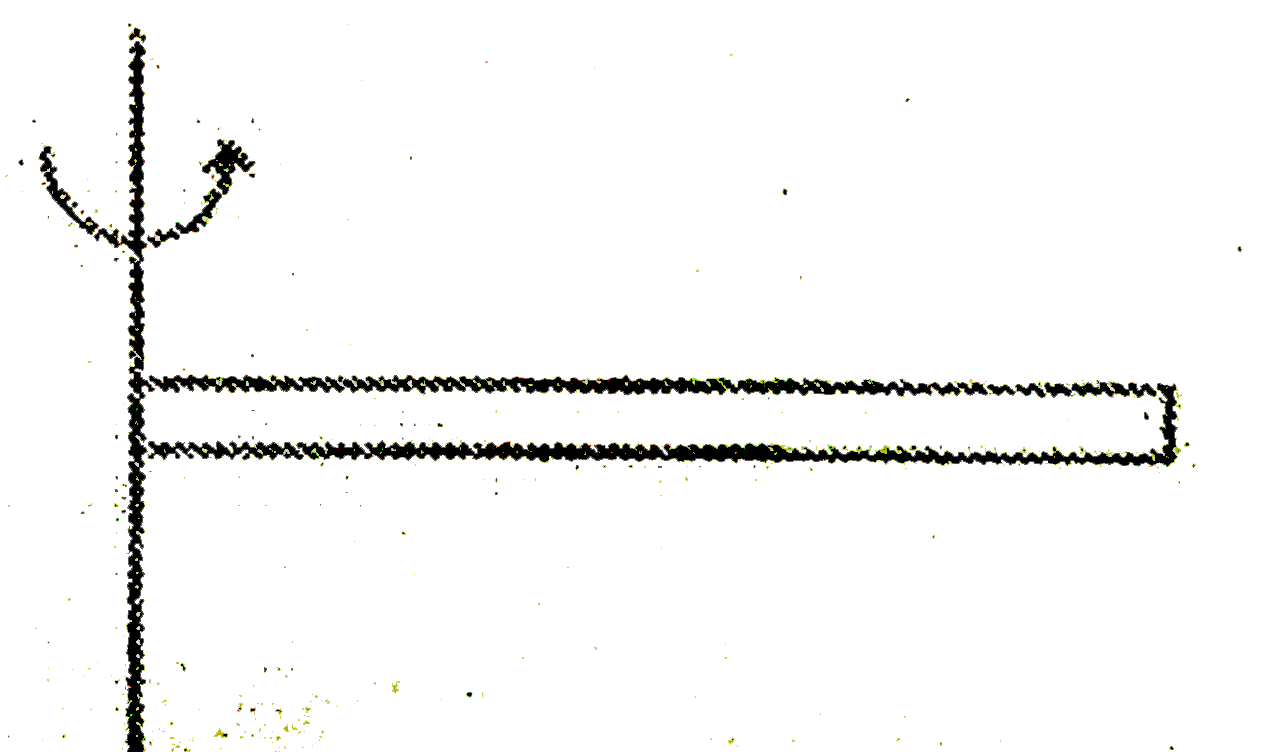
View SolutionA metallic rod of length 1m is rigidly clamped at its midpoint. Longitudinal stationary waves are set up in the rod in such a way that there are two nodes on either side of the mid - point and those of constituent waves in the rod . (Y=2×1011N/m2andρ=8×103kg/m3)
View SolutionIf the young's modulus of the material of the rod is 2 xx 10^(11) N//m^(2) and its density is 8000 kg//m^(3) then the time taken by a sound wave to traverse 1m of the rod will be
View SolutionA thin rod of length 3 m is being rotated with 10 rad/sec about its one end. The change in length of the rod due to rotation is (Given : Young's modulus of elasticity of the wire, Y=3×108SI unit, density of the rod is 104 Unit)

View SolutionLongitudinal waves of frequency 400Hz are produced in a rod of material of density 8000 kg//m^3 and Young modulus 7.2 xx 10^(10) N//m^2 . What is the wavelength of the wave?
View SolutionA steel rod 2.5m long is rigidly clamped at its centre C and longitudinal waves are set up on both sides of C by rubbing along the rod . Young's modulus for steel =2×1011N/m2 , density of steel =8000kg/m3

If the clamp of the rod be shifted to its end A and totally four antinodes are observed in the rod when longitudinal waves are set up in it , the frequency of vibration of the rod in this mode is
View Solution
