The transverse displacement y(x,t) of a wave on a string is given by y(x,t)=e−(ax2+bt2+2√(ab)xt). This represents a : Solution in Gujrati
Standing wave of frequency √b
standing wave of frequency 1√b
wave moving in +x direction with speed √ab
wave moving in -x direction with speed √ba
The correct Answer is:D
y(x1t)=e−[√ax+√bt]2
v=ω/K=√b√a in −ve x direction
Topper's Solved these Questions
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-05)[B]
42 Videos
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-04)[B]
13 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
A string of length 0.4m and mass 10−2kg is clamped at one end . The tension in the string is 1.6N. The identical wave pulses are generated at the free end after regular interval of time , Δt . The minimum value of Δt , so that a constructive interference takes place between successive pulses is
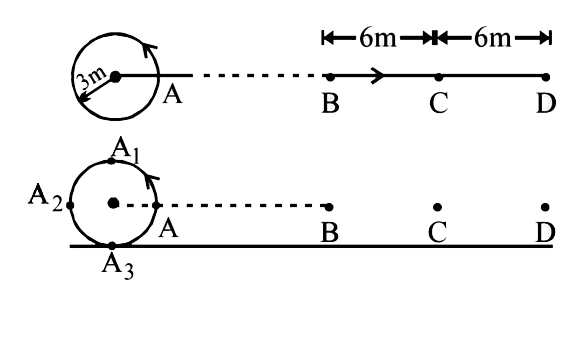
View SolutionA source of sound is moving along a circular orbit of radius 3meter with an angular velocity of 10rad/s. A sound detector located far away from the source is executing linear simple harmonic motion along the line BD with an amplitude BC=CD=6meters. The frequency of oscillation of the detector is 5π per second. The source is at the point A when the detector is at the point B. If the source emits a continuous sound wave of frequency 340Hz, Find the maximum and the minimum frequencies recorded by the detector.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionThe transverse displacement y(x,t) of a wave on a string is given by y(x,t)=e−(ax2+bt2+2√(ab)xt). This represents a :
View SolutionA transverse wave travelling on a taut string is represented by:
Y=0.01sin2π(10t−x)
Y and x are in meters and t in seconds. Then,
View Solutionएक डोरी पर तरंग का अनुप्रस्थ विस्थापन समीकरण yx,t=e−(ax2+bt2+2√abxt) है। यह प्रदर्शित करता है :
View SolutionThe transverse displacement of a string is given by y(x,t)=0.06sin(2π3)xcos(120πt) where 'x' & 'y' are 'm' and 't' is s. The length of the string is 1.5m and its mass is 3.0×10−2kg.
Does the function represent a travelling wave or stationary wave ?
View SolutionGiven below are some functions of x and t to represent the displacement (transversal or longitudinal) of an elastic wave. Identify the waves . y = 2 cos (3x) sin (10t)
View SolutionGiven below are some functions of x and t to represent the displacement (transversal or longitudinal) of an elastic wave. Identify the waves .
y=2√x−vt
View SolutionGiven below are some functions of x and t to represent the displacement (transversal or longitudinal) of an elastic wave. Identify the waves . y = cos x sin t + cos 2 x sin 2 t
View SolutionNo one is moving a diagonal wave along it. The displacement of the particles above the wire y(x, t) = e^-(ax^2 + bt^2 + 2sqrt(ab)*xt) Is indicated by the equation | Expresses the equation
View SolutionThe transverse displacement y(x,t) of a wave on a string is given by y (x,t) = e−(ax2+bt2+2√abxt) . This represents a :
View Solutionएक डोरी पर उत्पन्न एक तरंग का अनुप्रस्थ विस्थापन y(x, t ) निम्न है
y(x,t)=e−(ax2+bt2+2√abxt)
View SolutionThe transverse displamcement y(x,t) of a wave y(x,t ) on a string is given by yox, t) = . This represents a
View Solutionएक डोरी पर उत्पन्न एक तरंग का अनुप्रस्थ विस्थापन y(x, t ) निम्न है
y(x,t)=e−(ax2+bt2+2√abxt)
View Solution
