Heart leaks into a vessel, containing one mole of an ideal monoatomic gas at a constant rate of R4s−1 where R is universal gas constatant. It is observed that the gas expands at a constant rate dVdt=V0400(second) where V0 is intial volume. The inital temperature is given by T0=40K. What will be final temperature of gas at time t=400s.Solution in Gujrati
40K
80K
200K
260K
The correct Answer is:B
dVdt=V0400
V=V0(1+t400)
ΔRΔt=dUdt+pdVdt
R4=32RdTdt+RTV0(1+t400)V0400
14=32dTdt+T(t+400)
dTdt+23T(t+400)=16
T=t+40010+C(t+400)−2/3
at t=0T=40K⇒C=0
at t=400S
T=80K
Topper's Solved these Questions
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - part-2 physics
73 Videos
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - part-2 physic
48 Videos
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - PAPER 3
92 Videos
- TEST PAPER 4BOOK - ALLEN CHAPTER - TEST PAPER 4EXERCISE - PHYSICS
45 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLEN CHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
A bead is connected with a fixed disc of radius R by an inextensible massless string in a smooth horizontal plane. If the bead is pushed with a velocity v0 prependicular to the string, the bead moves in a curve and consequently collapses on the disc. Then

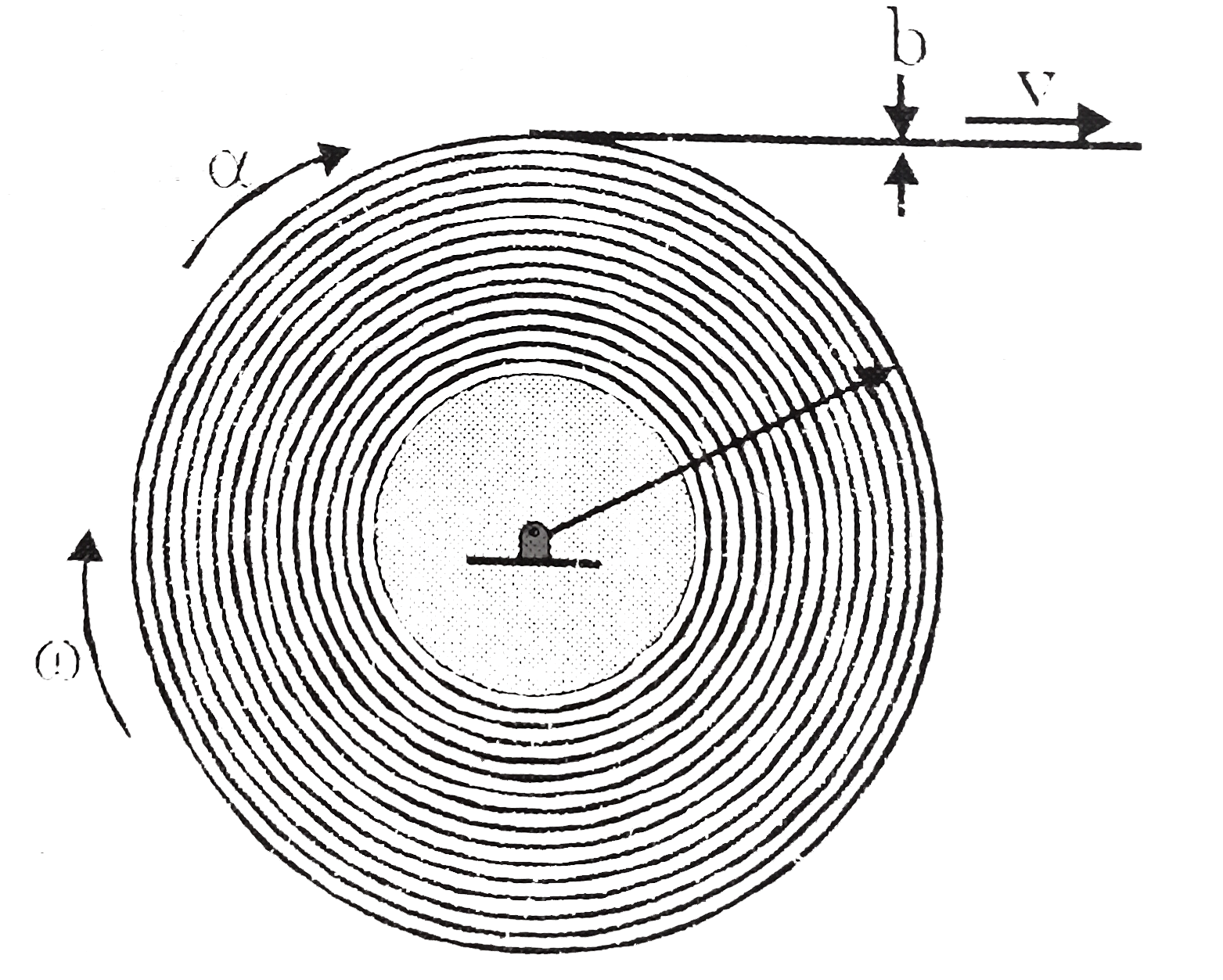
View SolutionIn a continuous printing process paper is drawn into the press at a constant speed v. Denoting by 'r' the radius of the paper roll at any given time and by 'b' the thickness of the paper. What is the angular acceleraion of the paper roll?
View SolutionA satellite S is moving in an elliptical orbit around the earth. The mass of the satellite is very small compared to the mass of the earth.
View SolutionTwo forces, each of magnitude F have a resultant of the same magnitude F. The angle between the two forces is
View SolutionMatch List I with List II and select the correct answer using the codes given below the lists :

View SolutionA vessel of volume V_(0) contains an ideal gas at pressure p_(0) and temperature T. Gas is continuously pumped out of this vessel at a constant volume-rate dV/dt=r keeping the temperature constant, The pressure inside the vessel. Find (a) the pressure of the gas as a function of time,(b) the time taken before half the original gas is pumped out.
View SolutionA gas is found to obey the law P2V=constant. The initial temperature and volume are T0andV0. If the gas expands to a volume 3V0, its final temperature becomes
View SolutionA gas is found to obey the law P^(2)V = constant. The initial temperature and volume are T_(0) and V_(0^(@)) If the gas expands to a volume 3V_(0) , then
View SolutionFor one mole of ideal gas if P=(P_(0))/(1+((V)/(V_(0)))) where P_(0) and V_(0) are constant, then temperature of gas when V=V_(0) is:
View SolutionA gas is found to obey the law P^(2) V= constant the innitial temperature and volume are T_(0) and V_(0) . If the gas expands to volume 2V_(0) , its final temperature becomes-
View SolutionOne mole of an ideal monoatomic gas at temperature T_(0) expands slowely according to the law P/V=constant. If the final temperature is 2T_(0) heat supplied to the gas is -
View SolutionOne mole of an ideal monoatomic gas undergoes the process T=T_(0)+4V , where T_(0) is initial temperature. Find (i) Heat capacity of gas as function of its volume. (ii) The amount of heat transferred to gas if its volume increases from V_(0) to 4V_(0) .
View SolutionA gas is found to obey the law p^(2) V = constant. The initial temperature and volume are T_(0) and V_(0) . If the gas expands to a volume 3V_(0) , its final temperature becomes
View SolutionIf 2 mol of an ideal monatomic gas at temperature T0 are mixed with 4 mol of another ideal monatoic gas at temperature 2T0 then the temperature of the mixture is
View SolutionIn a thermodynamic process two moles of a monatomic ideal gas obeys PV–2=constant. If temperature of the gas increases from 300 K to 400 K, then find work done by the gas (Where R = universal gas constant)
View SolutionA vessel of volume V_(0) contains an ideal gas at pressure p_(0) and temperature T. Gas is continuously pumped out of this vessel at a constant volume-rate dV/dt=r keeping the temperature constant, The pressure inside the vessel. Find (a) the pressure of the gas as a function of time,(b) the time taken before half the original gas is pumped out.
View Solution
