Given that hyperbola xy−2y+3x=k is tangent to the ellipse 3x2−12x+2y2+12y+6=0 at two points A and B On the bases of above answer the following
Product of ordinates of A and B for fixed 'k' is Solution in Gujrati
−6
−4
3
none of these
The correct Answer is:C
(x−2)(y+3)=k−6
(x−2)28+(y+3)212=1
x−2=X,y+3−Y
Xy=k−6 …..(1)
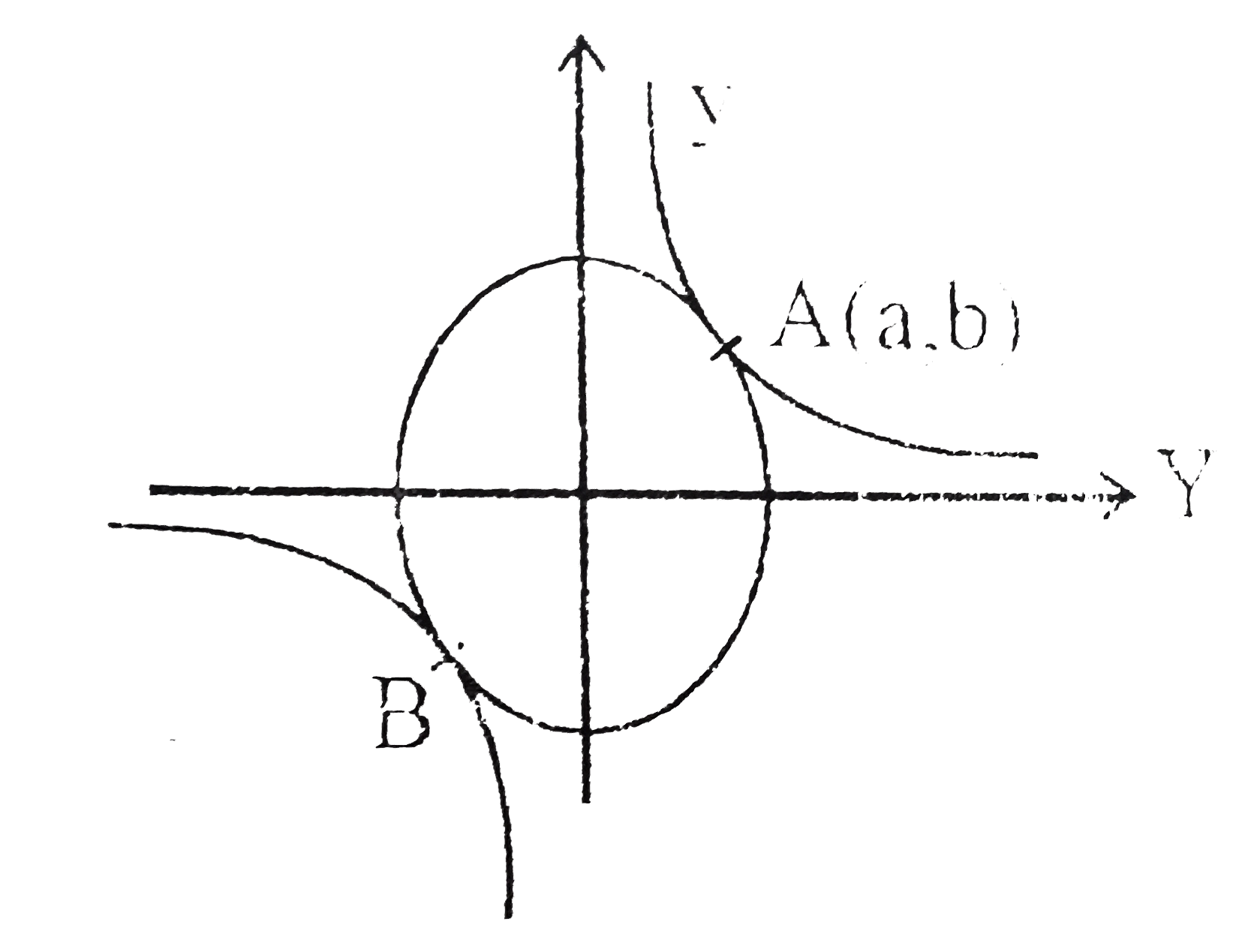
X28+Y212=1 ….(2)
At the point A and B, slopes of tangent to both curves must be equal
⇒y′=βα=−3α2β
2β2=3α2
since (α,β) satisfies equation (ii)
α28+β212=1
solving α=±2,β=±√6
α,β∈(2,√6)(−2,−√6)
α,β∈(2,−√6)(−2,√6)
k=6+2√6
k=6−2√6
coordinates of tangency
coordinates of tangency
(4,√6−3)(0,−√6−3)
(4,−√6−3)(0,√6−3)
Topper's Solved these Questions
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - part-2 Mathematics
48 Videos
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - PHYSICS
140 Videos
- TEST PAPERSBOOK - ALLEN CHAPTER - TEST PAPERSEXERCISE - part-2 Mathematics
15 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - CHEMISTRY SECTION-II
16 Videos
- VECTOR ALGEBRABOOK - ALLEN CHAPTER - VECTOR ALGEBRAEXERCISE - All Questions
1 Videos
Similar Questions
P1=x+y+z+1=0,P2=x−y+2z+1=0,P3=3x+y+4z+7=0 be three planes. Find the distance of line of intersection of planes P1=0 and P2=0 from the plane P3=0.
View SolutionA gas can be taken from state a to c by two different reversible processes,a⇒c or a⇒b=c. During the direct process a⇒c,20.0J of work is done by the system and 30.0J of heat is added to the system. During the process a⇒b⇒,25.0J of heat are added to the system. How much work is done by the system during a⇒b⇒c?

View SolutionNumber of necklaces can be formed with 4 identical beads and two distinct jewels is 'k', then k divides
View SolutionThe molar mass of a substance , each molecule of which contains 9 C atoms , 13 H-atoms and 3.32×10−23 g of other component is (NA=6.022×1023)
View SolutionFind the value of a, for which point P(a3,2) is the midpoint of the line segment joining the points Q(−5,4)andR(−1,0).
View SolutionTangents are drawn to the hyperbola 3x2−2y2=25 from the point (0,52). Find their equations.
View SolutionTangents are drawn to 3x^(2)-2y^(2)=6 from a point P If the product of the slopes of the tangents is 2, then the locus of P is
View SolutionThe equation of a hyperbola,conjugate to the hyperbola 2x^(2)+3xy-2y^(2)+3x+y+2=0 is 2x^(2)+3xy-2y^(2)+3x+y+k=0 then k=
View SolutionGiven that hyperbola xy−2y+3x=k is tangent to the ellipse 3x2−12x+2y2+12y+6=0 at two points A and B On the bases of above answer the following
Product of possible values of k is
View SolutionGiven that hyperbola xy−2y+3x=k is tangent to the ellipse 3x2−12x+2y2+12y+6=0 at two points A and B On the bases of above answer the following
Product of ordinates of A and B for fixed 'k' is
View SolutionGiven that hyperbola xy−2y+3x=k is tangent to the ellipse 3x2−12x+2y2+12y+6=0 at two points A and B On the bases of above answer the following
Length of chord AB of ellipse is
View SolutionFind the equation of tangent to the ellipse 3x2+y2+2y=0 which are perpendiculor to the line 4x−2y=1.
View SolutionThe equation of the tangent to the ellipse 3x2+2y2=30 at (-2, 3) is
View SolutionTangents are drawn from (-2,1) to the hyperola 2x2−3y2=6. Find their equations.
View SolutionThe asymptotes of the hyperbola xy–3x–2y=0 are
View SolutionIf 3x+2y−1=0 is a tangent to a hyperbola x216−y29=1, then the point of contact is
View Solution
