The line of action of a force →F=(−3ˆi+ˆj+5ˆk)N passes through a point (7,3,1). The moment of force (→τ=→r×→F) about origin is given bySolution in Gujrati
14ˆi+38ˆj+16ˆk
14ˆi+38ˆj−16ˆk
14ˆi−38ˆj+16ˆk
14ˆi−38ˆj−16ˆk
The correct Answer is:C
→τ=→r×→F=∣∣ ∣ ∣∣ˆiˆjˆk731−315∣∣ ∣ ∣∣=14ˆi−38ˆj+16ˆk
Topper's Solved these Questions
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Exercise-03
1 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Exercise-04
1 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Exersice -05(B)
20 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-IV
7 Videos
Similar Questions
Two parallel metallic plates have surface charge densities σ1 and σ2 as shown in figure.
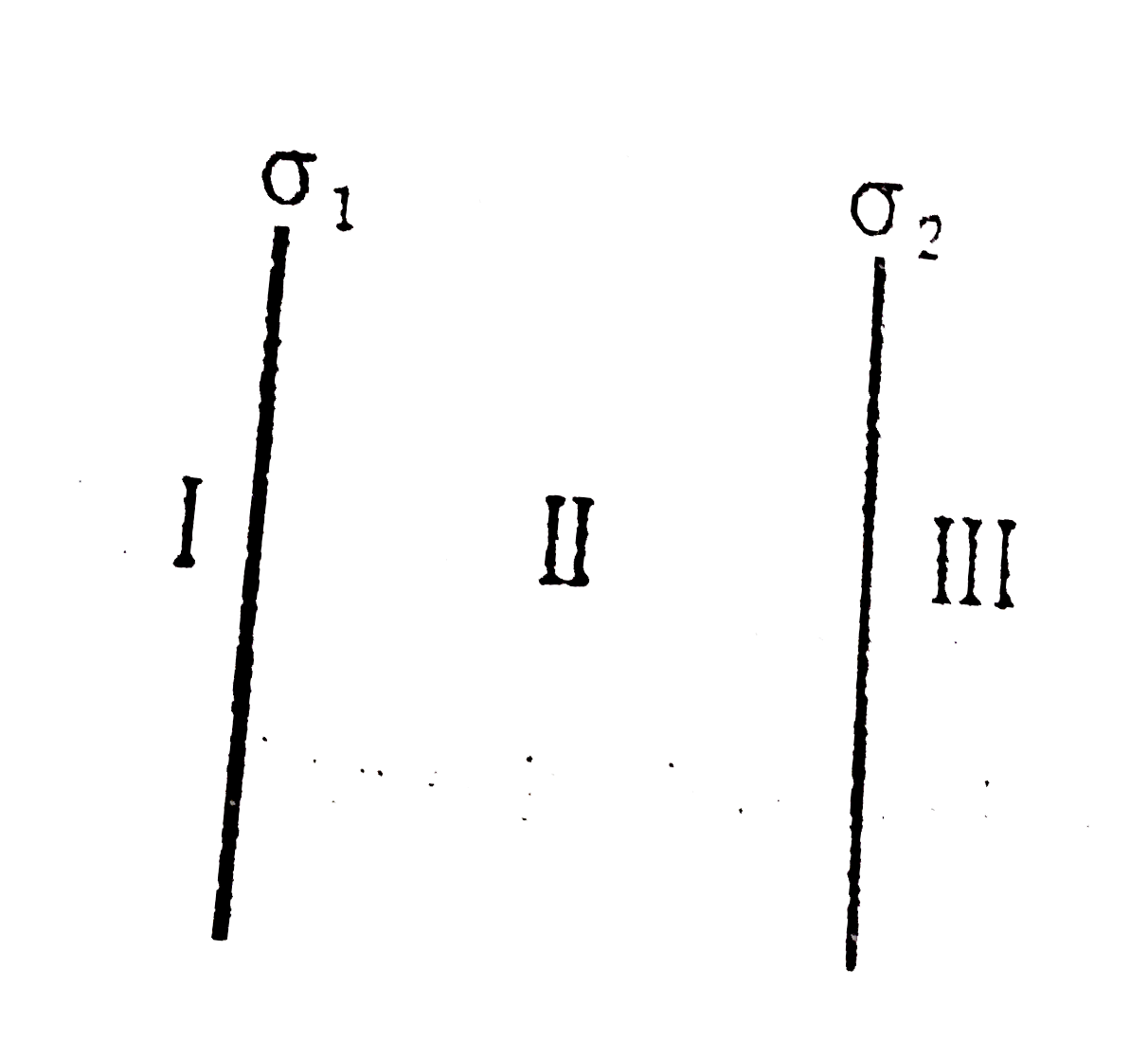
Column-IColumn-II(A)If σ1+σ2=0(p)Electric field in region III is towards right(B)Ifσ1+σ2>0(q)Electric field in region I is zero(C)If σ1+σ2<0(r)Electric field in region I is towards roght(s)Nothing can be said
View SolutionIn each situation of Column-I two elelctric dipoles having moments →p1 and →p1 of same magnitude (that is ,p1=p2) are placed on x-axis symmetrically about origin in different orientations as shown. In column-II certain inferences are drawn for these two dipoles. Then match the different orientations of dipole in column-I with the corresponding result in column-II.

View SolutionPlanck's constant has dimensions …………….
View SolutionA football player kicks a ball at an angle of 30∘ with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, (g=10m/s2)
View SolutionAssertion : Gravitational forces do not depend on the medium between the masses.
Reason : Mediating particle responsible for gravitational force is graviton.
View SolutionFind ∣∣→a×→b∣∣,if →a=2ˆi+ˆj+3ˆk and →b=3ˆi+5ˆj−2ˆk.
View SolutionLet vec a=hat i+hat j and vec b=2hat i-hat k. The point of intersection of the lines vec r xxvec a=vec b xxvec a and vec r xxvec b=vec a xxvec b is
View SolutionLet vec a=hat i+hat j and vec b=2hat i-hat k. The point of intersection of the lines vec r xxvec a=vec b xxvec a and vec r xxvec b=vec a xxvec b is
View SolutionIf →a=ˆi−2ˆj+3ˆk, and →b=2ˆi+3ˆj−5ˆk, then find →a×→b. verify that →a and →a×→b are perpendicular to each other.
View SolutionThe line of action of a force vec(F)=(-3hat(i)+hat(j)+5hat(k)) N passes through a point (7, 3, 1) . The moment of force (vec(tau)=vec(r)xxvec(F)) about origin is given by
View SolutionThe torque of force →F=−3ˆi+ˆj+5ˆk acting at the point →r=7ˆi+3ˆj+ˆk is ______________?
View Solutionयदि →A=3ˆi+2ˆj+ˆk तथा →B=5ˆi−5ˆj+ˆk, हो तो →A⋅→B तथा →A×→B का मान ज्ञात कीजिये ।
View SolutionIf →a=2ˆi+3ˆj−ˆk,→b=3ˆi+5ˆj+2ˆk,→c=−ˆi−2ˆj+3ˆk,
(→a×→b)×→c=(→a⋅→c)→b−(→b⋅→c)→a
View SolutionIf →a=2ˆi+3ˆj−ˆk,→b=3ˆi+5ˆj+2ˆk,→c=−ˆi−2ˆj+3ˆk,
→a(→a×→b)=(→a⋅→c)→b−(→a⋅→b)→c
View Solutionबल →F=3ˆi+4ˆj−5ˆk तथा विस्थापन →d=5ˆi+4ˆj+3ˆk के बीच का कोण ज्ञात कीजिए | →F का पर प्रक्षेप भी ज्ञात कीजिए |
View SolutionThe torque of a force vec(F)=-2hat(i)+2hat(j)+3hat(k) acting on a point vec(r )=hat(i)-2hat(j)+hat(k) about origin will be
View Solution
