Statement-1 : If two concentric conducing sphere which are connected by a conducting wire. No charge can exist on inner sphere.
Statement-2: When charge on outer sphere will exist then potential of inner shell and outer shell will be same.Solution in Gujrati
Statement-1 is True, Statement-2 is True, Statement-2 is a cor.
Statement-1 is True, Statement-2 is True, Statement-2 is not a
Statement-1 is False, Statement-2 is True
Statement-1 is True, Statement-2 is False.
The correct Answer is:D
Step by step video & image solution for Statement-1 : If two concentric conducing sphere which are connected by a conducting wire. No charge can exist on inner sphere. Statement-2: When charge on outer sphere will exist then potential of inner shell and outer shell will be same. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Comprehension 1
5 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Comprehension 2
8 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Exersice-03
7 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-IV
7 Videos
Similar Questions
The van der waal's gas equation is (P+aV2)(V−b)=RT, where P is pressure, V is molar volume and T is the temperature of the given sample of gas. R is called molar gas constant, a and b are called van der waal's constants.
The dimensional formula for b is same as that for
View SolutionTwo ships, V and W, move with constant velocities 2ms−1 and 4ms−1 along two mutually perpendicular straight tracks toward the intersection point O. At the moment t=0, the ships V & W were located at distances 100m and 200m respectively from the point O.
The distance between them at time t is :-
View SolutionColumn IColumn II(A)1fermi(p)10−13m(B)1X-ray unit(q)10−15m(C)1angstrom(r)10−10m(D)1Astronomical unit(s)9.46×1015m(E)1Light year(t)3.26Light year(F)1Parse(u)3.08×1016m(v)1.49×1011m
View SolutionA football player kicks a ball at an angle of 30∘ with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, (g=10m/s2)
View SolutionAssertion : Gravitational forces do not depend on the medium between the masses.
Reason : Mediating particle responsible for gravitational force is graviton.
View SolutionConsider two concentric conducting spheres. The outer sphere is hollow and initially has a charge -7Q on it. The inner sphere is solid and has a charge +2Q on it. a. How much charge is on the outer surface and inner surface of the outer sphere. b. If a wire is connected between the inner and outer sphere, after electrostatic equilibrium is established how much total charge is on the outer sphere? How much charge is on the outer surface and inner surface of the outer sphere? Does the electric field at the surface of the inside sphere change when the wire is connected? c. We return to original condition in (a). We now connect the outer sphere to ground with a wire and then disconnect it. How much total charge will be on the outer sphere? How much charge will be on the inner surface and outer surface of the outer sphere?
View SolutionA conducting sphere of radius R and a concentric thick spherical shell of inner radius 2R and outer radius 3R is shown in figure. A charge +10Q is given to the shell and inner sphere is earthed. Then charge on inner sphere is
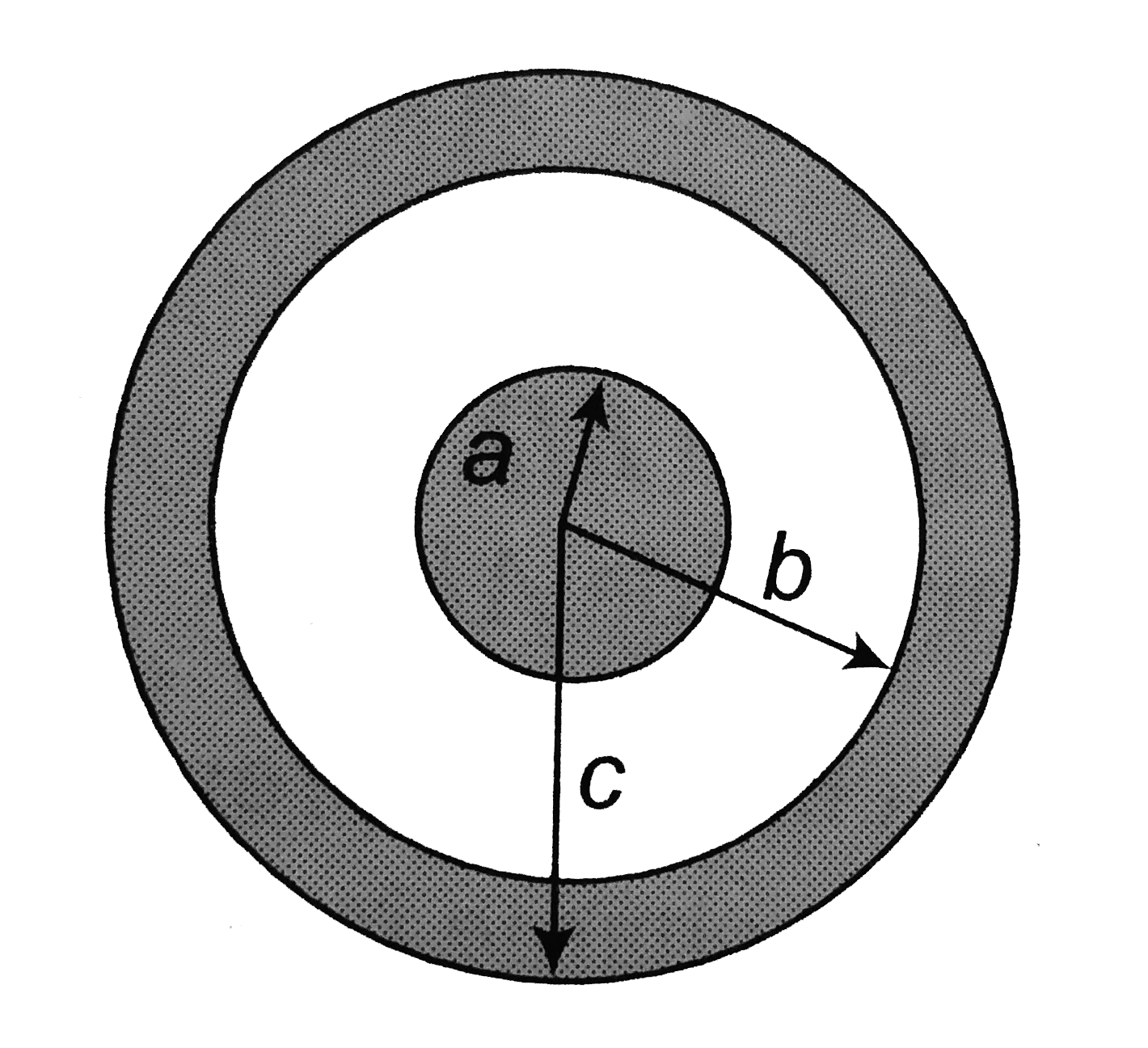
View SolutionA solid conducting sphere of radius a has a net positive charge 2Q. A conducting spherical shell of inner radius b and outer radius c is concentric with the solid sphere and has a net charge −Q. The surface charge density on the inner and outer surfaces of the spherical shell will be
View SolutionFigure shows three concentric conducting spherical shells with inner and outer shells earthed and the middle shell is given a charge q. Find the charge distribution of sphere

View SolutionA conducting sphere of radius R carries a charge Q. It is enclosed by another concentric spherical shell of radius 2R. Charge from the inner sphere is transferred in infinitesimally small installments to the outer sphere. Calculate the work done in transferring the entire charge from the inner sphere to the outer one.
View SolutionAssertion: Two concentric charged spherical shells are given. The potential difference between the shells depends on charge of inner shell.
Reason: Potential due to charge of outer shell remains same at every point inside the sphere.
View SolutionConsider two concentric conducting spheres.The " outer sphere is hollow and initially has a charge −7Q on it.The inner sphere is solid and has a change +2Q on it.How much charge is on the outer surface and inner surface of the outer sphere??
View SolutionTwo concentric conducting spheres of radii R and 3R carrying charges Q and 2Q respectively.If the charge on inner sphere is doubled.The potential difference between inner and outer spheres will..?
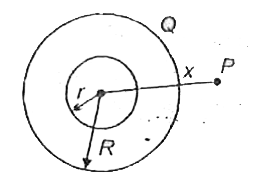
View SolutionTwo concentric hollow conducting spheres of radius r and R are shown. The charge on outer shell is Q. what charge should be given to inner sphere so that the potential at any point P outside the outer sphere is zero ?
View SolutionTwo concentric metallic shells are of radii r1andr2(r2>r1). If charge given to outer sphere is q. and the inner sphere is ground. Then the charge on the inner sphere q1 is

View SolutionConsider two concentric conducting spheres. The outer sphere is hollow and the inner sphere is solid. Initially they are given a charge - 7Q and a charge + 2Q respectively then the charges on the outer surface and inner surface of the outer spher is
View Solution
