A particle is moving with a velocity of →v=(3ˆi+4tˆj)m/s. Find the ratio of tangential acceleration to that of total acceleration at t=1sec Solution in Gujrati
(4)/(5)
(3)/(5)
(5)/(4)
(3)/(4)
The correct Answer is:A
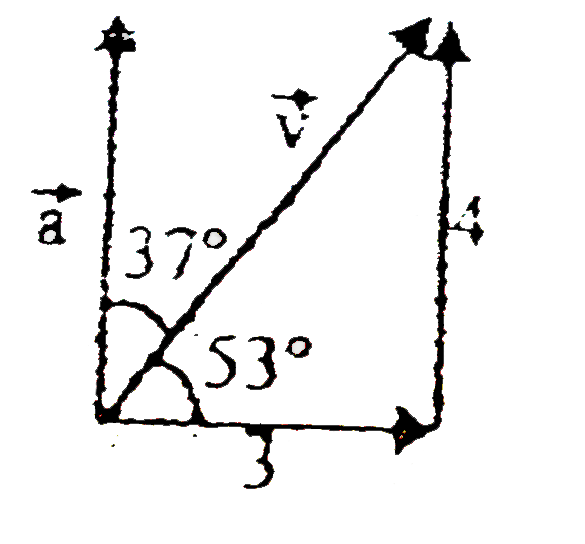
At t=1s,v=√32+42=5,→a=→dvdt=4ˆj
at=4cos372=165⇒ata=16/54=45
Topper's Solved these Questions
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- TEST PAPER 1BOOK - ALLEN CHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
Choose the correct statement:
View SolutionChoose the correct statement:
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionWhy are electric field lines perpendicular at a point on an equipotential surface of a conductor ?
View SolutionA particle moves in the x−y plane under acceleration →a=3ˆi+4ˆjm/s2. If the initial velocities is →u=6ˆi+8ˆjm/s. Find the velocity and the position vector of a particle after 2s.
View SolutionVelocity and acceleration vectors of a charged particle moving in a magnetic field at some instant are vec(v)=3hat(i)+4hat(j) and vec(a)=2hat(i)+xhat(j) . Selcet the wrong alternative.
View SolutionA particle travels so that its acceleration is given by →a=5costˆi−3sintˆj. If the particle is located at (−3,2) at time t=0 and is moving with a velocity given by (−3ˆi+2ˆj). Find
(i) The velocity [→v=∫→a.dt] at time t and
(ii) The position vector [→r=∫→v.dt] of the particle at time t(t>0).
View SolutionA particle moves with a tangential acceleration at=→a.ˆv where →a=(5ˆi)m/s2. If the speed of the particle is zero at x=0, then find v (in m/s) at x=4.9m.
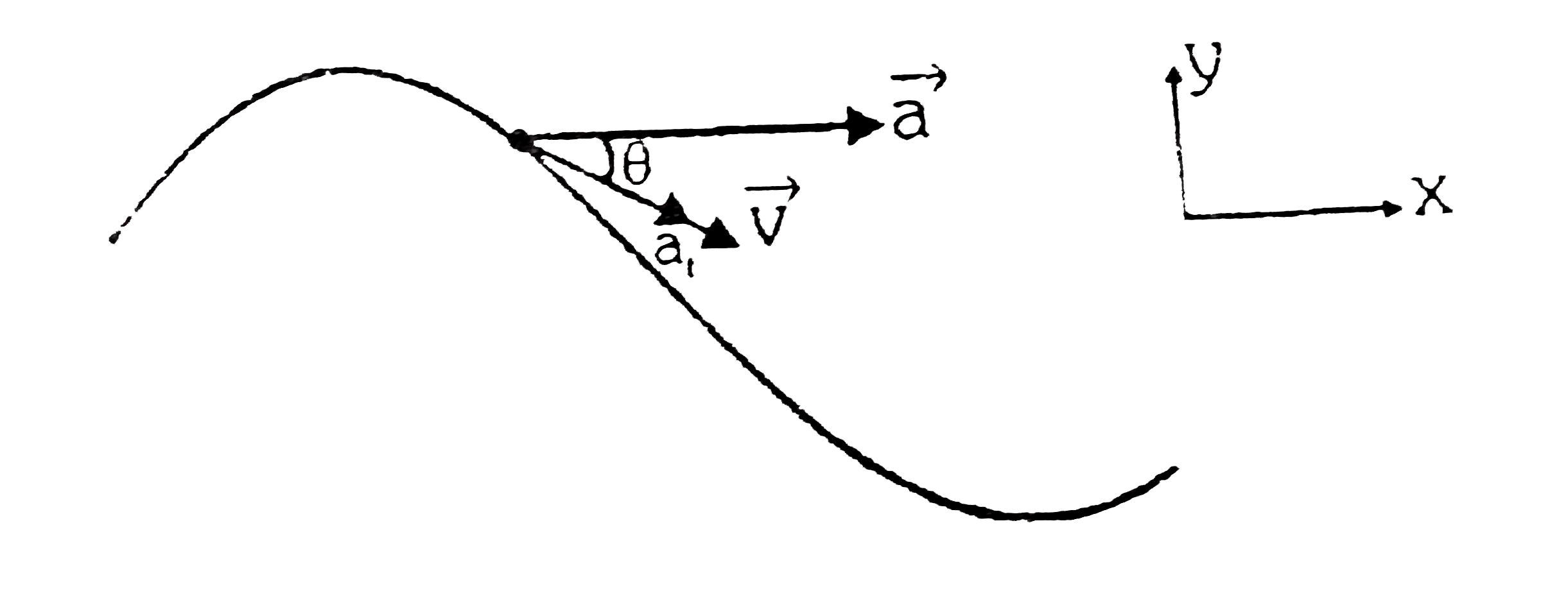
View SolutionFigure shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5 m at a given instant of time. At this instant, Find: (i) the radial acceleration, (ii) the speed of the particle and (iii) its tangential acceleration.
View SolutionVelocity and acceleration of a particle at time t = 0 are u=(2ˆi+3ˆj)m/s and a=(4ˆi+2ˆj)m/s2 respectively. Find the velocity and displacement of particle at t = 2 s.
View SolutionA particle moves in the X-Y plane with velocity →v=αˆi+βtˆj. At t=α√3β the magnitude of tangential, normal and total accelerations respectively are
View SolutionIf the velocity of a paraticle moving along x-axis is given as v=(4t2+3t=1)m/s then acceleration of the particle at t=1sec is :
View SolutionA particle of mass 2 kg moving with a velocity v1=(2ˆi−3ˆj)m/s experience a perfectly inelastic collision with another particle of mass 2 kg having velocity →v2=(3ˆj+6ˆk)m/s. Find the velocity and speed of the particle formed.
View SolutionA particle moves in the x-y plane with the velocity →v=aˆi−btˆj. At the instant t=a√3/b the magnitude of tangential, normal and total acceleration are ….. &…….
View Solutionx-y तल में गतिशील एक कण का वेग →v=aˆi+btˆj है । t=a√3/b समय पर, स्पर्शज्या, अभिलम्ब तथा कुल त्वरण ________, ______, तथा _____, है ।
View Solutionकण का वेग v(t)=t3+2t+1 द्वारा दिया जाता है
t=1sec समय पर कण का त्वरण ज्ञात करो।
View Solution
