A particle is projected with velocity 2(√gh), so that it just clears two walls of equal height h which are at a distance of 2h form each other. Show that the time of passing between the walls is 2(√hg). [Hint : First find velocity at height h. Treat it as initial velocity and 2h as the range. ]Solution in Gujrati
s=ut+12at2⇒a=(usinθ)t−12gt2
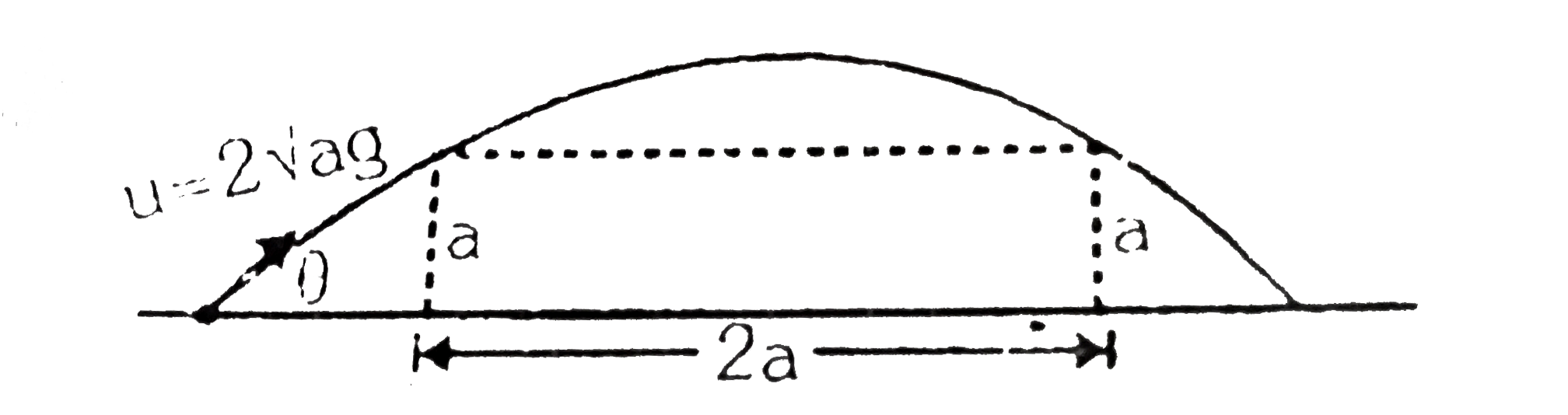
⇒t=usinθ±√u2sin2θ−2agg
Δt=2√u2sin2θ−2agg
For horizontal motion : 2a=ucosθ×Δt
⇒2a=ucosθ×2√u2sin2θ−2agg⇒θ=60∘
∴Δt=2aucosθ=2a2√ag×12=2√ag
Topper's Solved these Questions
- KINEMATICSBOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - Exercise-05 [A]
11 Videos
- KINEMATICSBOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - Exercise-05[B]
5 Videos
- KINEMATICSBOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - EXERCISE-04[A]
35 Videos
- ERROR AND MEASUREMENTBOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
A ball whose kinetic energy is E , is projected at an angle of 45∘ to the horizontal . The kinetic energy of the ball at the highest point of its flight will be
View SolutionA particle goes from point A and B, moving in a semcircle of raidus 1.0m, in 1.0 s, as shown in the figure. The magnitude of the average velocity of the particle is
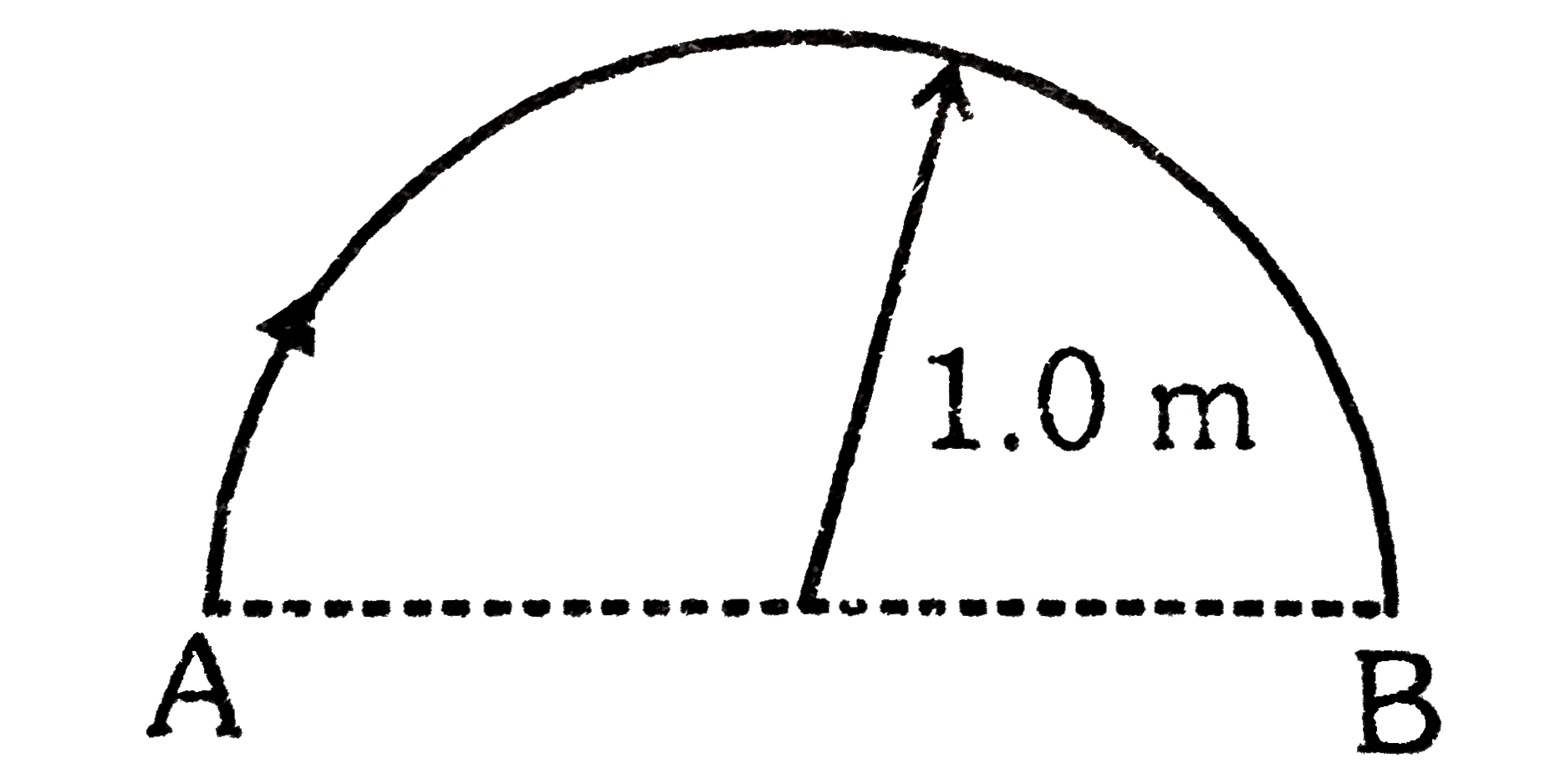
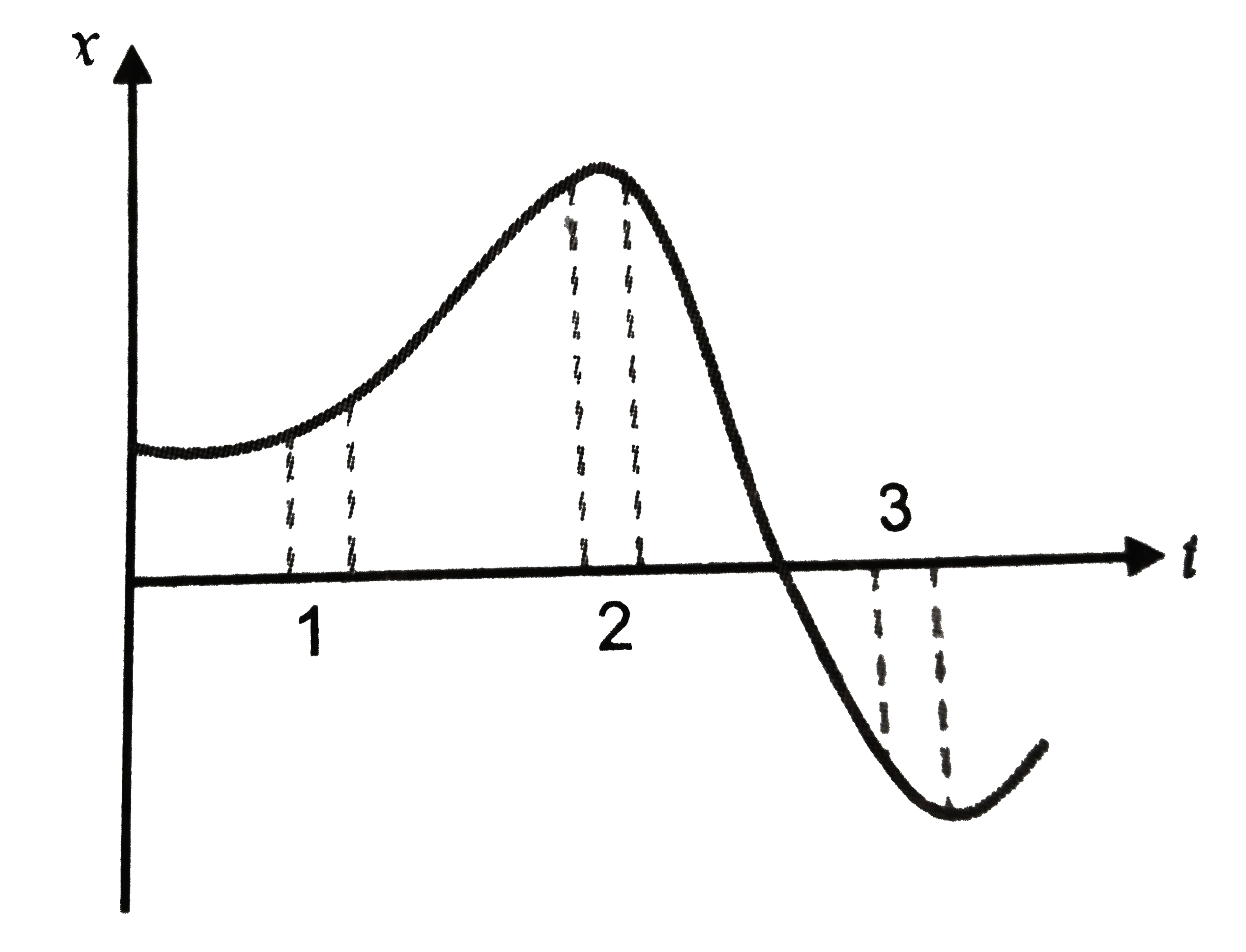
View SolutionFig. 2 (NCT).9 show the x−t plot of a particle in one dimensional motion. Three different equal intervals of time are shown. In which interval the average speed is greatest and in which it is the least ? Give th sign of average speed for each interval.
 .
.
View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
View SolutionA football player kicks a ball at an angle of 30∘ with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, (g=10m/s2)
View SolutionA particle is projected from the ground at t=0 so that on its way it just clears two vertical walls of equal height on the ground. The particle was projected with initial velocity u and at angle θ with the horiozontal. If the particle passes just grazing top of the wall at time t=t1andt=t2, then calculate.
(a) the height of the wall.
(b) the time t1andt2 in terms of height of the wall.
Write the expression for calculating the range of this projectile and separation between the walls.
View SolutionA particle is projected with velocity 2sqrt(ag) so that it just clears two walls, of equal height a, at a distance 2a from each other . Show that the velocity of projection is 60^@ and that the latus rectum of the path is 2a.
View SolutionA particle is projected with a velocity u from a point at ground level. Show that it cannot clear a wall of height h at a distance x from the point of projection if u2<g(h+√h2+x2).
View SolutionA particle is projected with velocity 2√gh so that it just clears two walls of equal height h which are at a distance 2h from each other. Show that the time of passing between the walls is 2√h/g.
View SolutionAn object is projected so that it just clears two walls of height 7.5 m and with separation 50m from each other. If the time of passing between the walls is 2.5s, the range of the projectile will be (g= 10m//s^(2) )
View SolutionA particle is projected with velocity 2 sqrt(gh) and at an agnle 60^(@) to the horizontal so that it just clears two walls of equal height h which are at a distance 2h from each other. The time taken by the particle to travel between these two wall is
View SolutionA particle is projected with velocity 2√gh so that it just clears two walls of equal height h which are at a distance 2h from each other. Show that the time of passing between the walls is 2√h/g.
View Solutionएक कण 2√gh के वेग से प्रक्षिप्त किया जाता है जिससे कि वह h ऊँचाई की दो दीवारों को पार करे और दीवारें एक -दूसरे से h दूरी पर हैं। तब प्रक्षिप्त कोण है
View SolutionA particle 2sqrt(gh) Is projected in such a way that it crosses two walls of height h, the distance between the two is 2h. The time taken to cross both walls is
View SolutionA particle is projected with velocity 2√gh so that it just clears two walls of equal height h which are a distance 2 h from each other. The time interval for which the particle travels between this two walls is (θ=60∘)
View Solution
