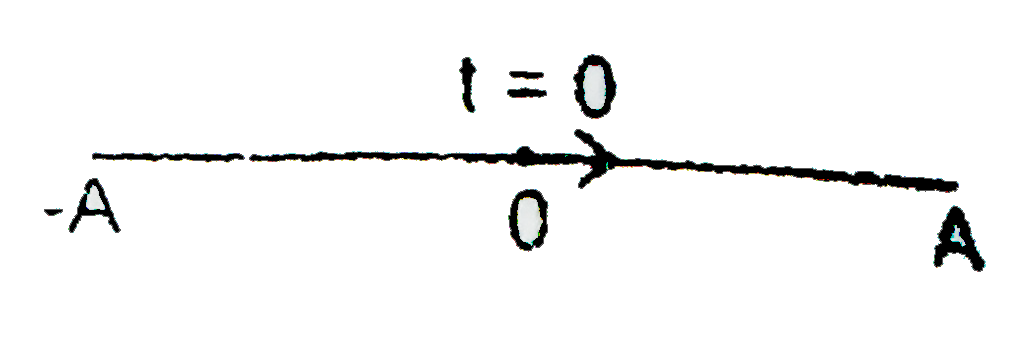
A particle starts from mean position and moves towards positive extreme as show below. Find the equation of the SHM, Amplitude of SHM is A.
_S01_003_Q01.png) Solution in Gujrati
Solution in Gujrati
General equation of SHM can be written as x=Asin(ωt+ϕ)
At t=0,x=0,∴0=Asinϕ,∴ϕ=0,π,ϕ∈[0,2π]
Also, at t=0,v=+ve,∴Aωcosϕ=maximum+ve or ϕ=0
Hence, if the particle is at mean position at t=0 and is moving towards +ve extreme, then the equation of SHM is given by x=Asinωt
_S01_003_S01.png)
Similarly, for particle moving towards −ve extreme then ϕ=π
∴ equation of SHM is x=Asin(ωt+π) or, x=−Asinωt
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES
29 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-01
117 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
25 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
A simple pendulum has time period 2s. The point of suspension is now moved upward accoding to relation y=(6t−3.75t2)m where t is in second and y is the vertical displacement in upward direction. The new time period of simple pendulum will be
View SolutionThe equation of motion of a particle of mass 1g is d2xdt2+π2x=0, where x is displacement (in m) from mean position. The frequency of oscillation is (in Hz)
View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
View SolutionChoose the correct statement:
View SolutionA particle starts from mean position and moves towards positive extreme as show below. Find the equation of the SHM , Amplitude of SHM is A .
View SolutionA particle starts from point x = (-sqrt(3))/(2)A and move towards negative extreme as shown, (a) Find the equation of the SHM (b) Find the time taken by the particle to go directly from its initial position to negative extreme. (c) Find the time taken by the particle to reach at mean position.
View SolutionA particle start form mean position and moves towards positive extreme as shown. Find the equation of the SHM. Amplitude of SHM is A.
View SolutionWhat is the initial phase of a particle, in SHM, starting from an extreme position?
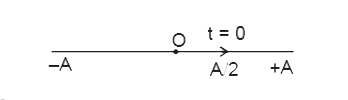
View SolutionA particle starts from A/2 and moves towards positive extreme as shown below. Find the equation of the SHM. Given amplitude of SHM is A.
View SolutionA particle starts from point x = (-sqrt(3))/(2) A and move towards negative extreme as shown (a) Find the equation of the SHM. (b) Find the time taken by the particle to go directly from its initial position to negative extreme. (c) Find the time taken by the particle to reach at mean position.
View SolutionA particle starts from rest at the extreme position and makes SHM with an amplitude A and angular frequency omega . Its displacement at time 't' is
View SolutionBasics: Oscillatory and periodic motion | Condition for SHM | Kinematics of SHM: Equation of SHM | Mean Position | Extreme position| Amplitude | Phase | Time period | Frequency
View Solution(A) : In SHM, acceleration is always directed towards the mean position. (R) : In SHM, the body has to stop momentarily at the extreme position and move back to mean position.
View SolutionA particle performing SHM with a frequency of 5 Hz and amplitude 2cm is initially in the positive extreme position. What is the equation for its displacement?
View SolutionA particle performing SHM with a frequency of 5 Hz and amplitude 2cm is initially in the positive extreme position. What is the equation for its displacement?
View SolutionHow will the velocity of a particle in SHM vary as it moves from the mean position to an extreme position?
View Solution
