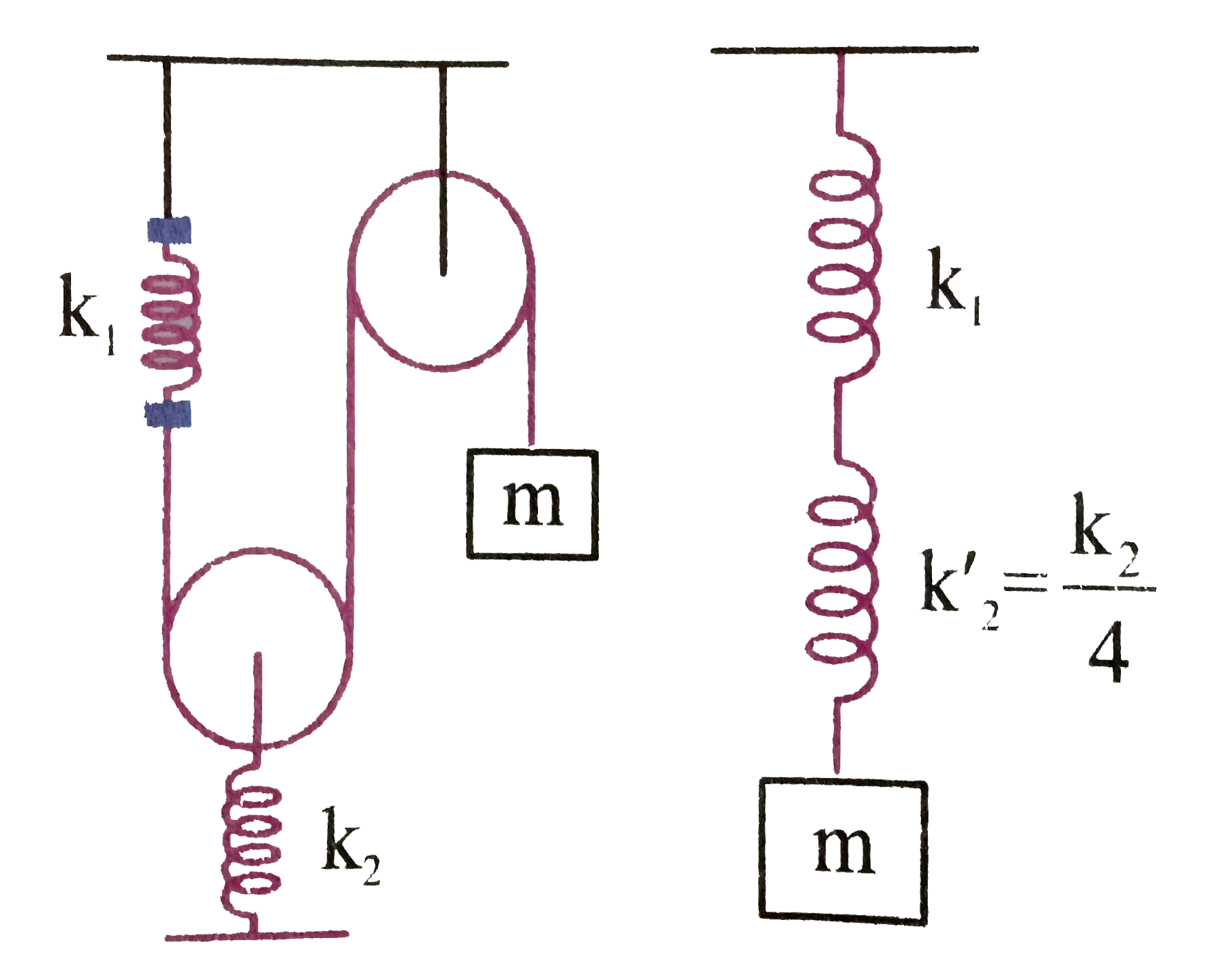
Figure shows a system consisting of a massless pulley, a spring of force constant k and a block of mass m. If the block is slightly displaced vertically down from is equilibrium position and then released, the period of its vertical oscillation is
 Solution in Gujrati
Solution in Gujrati
Case (A)
As the pully is fixed and string in inexrensible, if mass m is displaced by y the spring will stretch by y, and as there is no mass between string and spring (as pully is massless) F=T=ky i.e., restoring force is linear and so motion of mass m will be linear simple harmonic with frequnecy
nA=12π√km=12π√40001≈10Hz
_S01_020_S01.png)
Case (B) :
The pulley is mobale and string inetensible, so if mass m moves down a distance y, the pulley will move down by (y/2).
Now as pulley is massless F=2T,⇒T=f/2=(k/4y).
So the force in the spring F=k(y/2)
So, nB=12π√km=12π√k4m=nA2=5Hz
_S01_020_S02.png)
Case (C) :
In this sitution if the mass m moves by y the pulley will also move by y and so the string will stretch by 2y (as string is inextensible) and so T'=F=2ky.Now as pulley is massless so T=F+T'=4ky, i.e., the restoring force on the mass m
T=4ky=k'=4k
so nC=12π√k'm=12π√4km=2nA=20Hz
_S01_020_S03.png)
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES
29 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-01
117 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
25 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
A simple pendulum has time period 2s. The point of suspension is now moved upward accoding to relation y=(6t−3.75t2)m where t is in second and y is the vertical displacement in upward direction. The new time period of simple pendulum will be
View SolutionThe equation of motion of a particle of mass 1g is d2xdt2+π2x=0, where x is displacement (in m) from mean position. The frequency of oscillation is (in Hz)
View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
View SolutionChoose the correct statement:
View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View SolutionFigure shows a system consisting of a massles pulley, a spring of force constant k and a block of mass m . If the block is sligthtly displaced vertically down from its equilibrium and released, find the period of its vertical oscillation in cases (a) and (b).
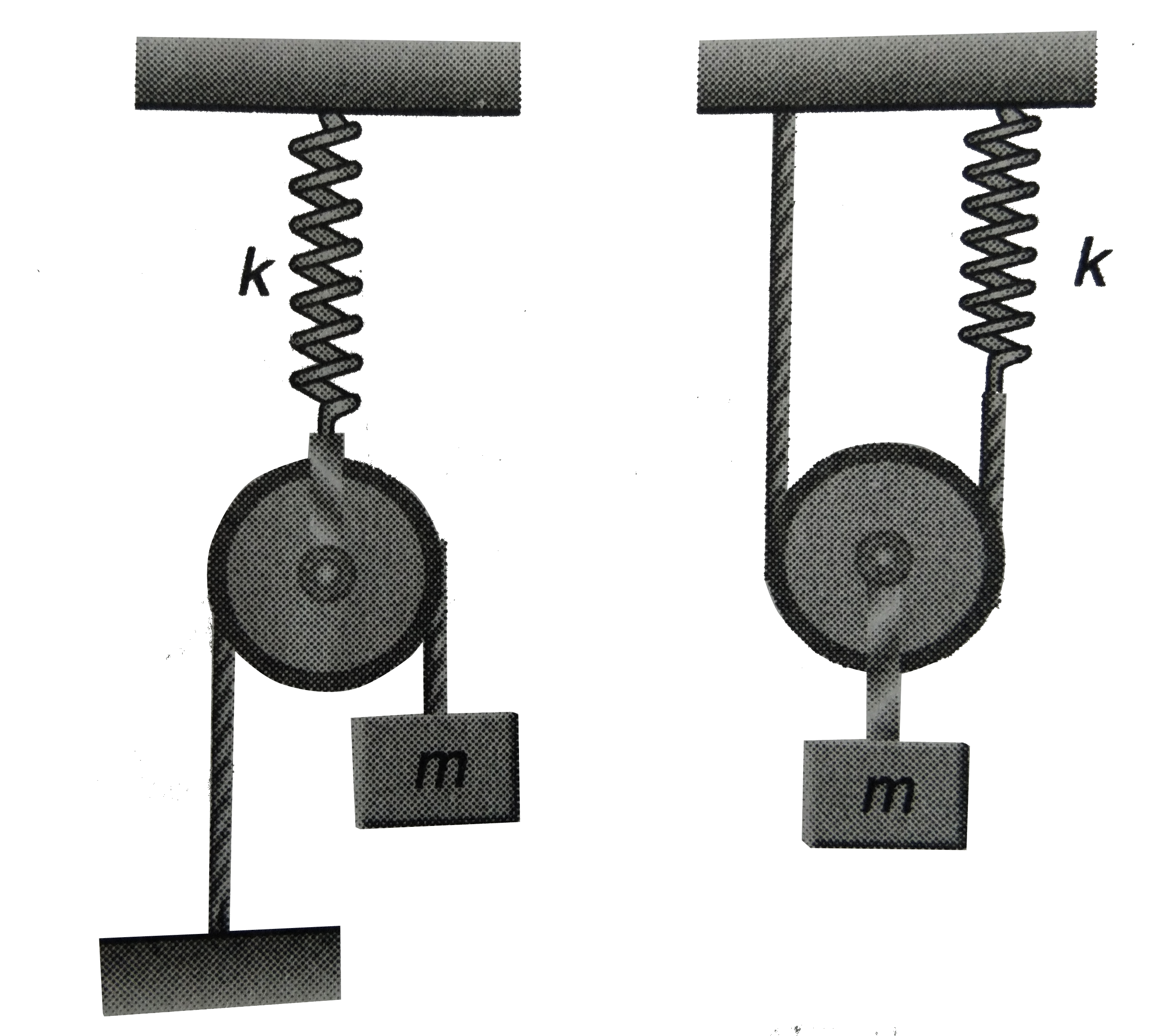
View SolutionIn the following arrangements, bock is slightly displaced vertically down from its equilibrium position and released. Find time period of vertical oscillations. The pulley is light.
View SolutionIn the device shown in Fig, the block m is displaced down slightly and released. It starts oscillating. Pulleys are smooth and massless string and springs are also massless. Find time period of oscillations.
View SolutionA block of mass m is connected with two ideal pullies and a massless spring of spring constant K as shown in figure. The block is slightly displaced from its equilibrium position. If the time period of oscillation is μπ√mK. Then find the value of μ.

View SolutionA system consists of two spring and a mass m = 1 kg as shown in figure . If mass m is dispalced slightly along vertical and vertical and released. The system oscillates with a period of 2 s .Then , the spring canstant K is

View SolutionFigure shows a system consisting of pulley having radius R, a spring of force constant k and a block of mass m. Find the period of its vertical oscillation.
View SolutionAs shown in the figure a block of mass m is hung from the ceiling by the system of springs consisting of two layers. The force constant of each of the springs is k. The frequency of the vertical oscillations of the block is
View SolutionA system shown in the figure consists of a massless pulley, a spring of force constant k displaced vertically downwards from its equilibrium position and released, then the Period of vertical oscillations is
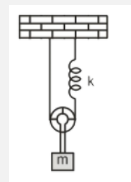
View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View Solutionएक m द्रव्यमान का गुटका स्प्रिंग नियतांक k की स्प्रिंग से ऊर्ध्वाधर रूप से लटक रहा है। यदि द्रव्यमान को ऊर्ध्वाधर रूप से दोलन कराया जाता है, तो इसकी कुल ऊर्जा होती है
View SolutionFigure shows a system consisting of a massles pulley, a spring of force constant k and a block of mass m. If the block is sligthtly displaced vertically down from its equilibrium and released, find the period of its vertical oscillation in cases (a) and (b).
View Solution