Let Rt represents activity of a sample at an insant and Nt represent number of active nuclei in the sample at the instant. T1/2 represents the half life.
Column IColumn II(A)t=T1/2(p)Rt=R02(B)t=T1/2ln2(q)N0−Nt=N02(C)t=32T1/2(r)Rt−R0R0=1−ee(s)Nt=N02√2Solution in Gujrati
The correct Answer is:(A) p,r (B) r (C) s
(A) In half life active sample reduce =R02
∴ Decay number of nuclei is =R02
(B) N=N0e−λt
where λ= decay constant, λ=ln(2)t1/2
N=N0e−ln2t1/2t1/2ln2⇒N=N0e
N0−N=N0[e−1e]⇒N−N0N0=1−ee
(C) N=N0(2)t/T1/2⇒N=N023/2⇒N02√2
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise - 07
2 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise - 08
2 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise - 05
2 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
25 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
The stopping potential for the photo-electrons emitted from a metal surface of work-function 1.7 eV is 10.4 eV. Find the wavelength of the radiation used. Also identify the energy-levels in hydrogen atom which will emit this wavelength.
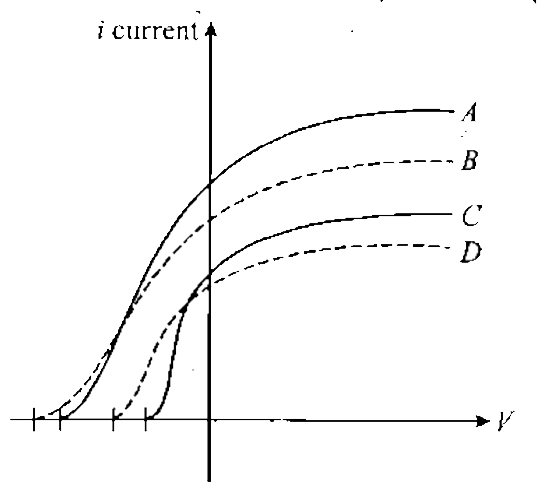
View SolutionThe figure shows the results of an experiment involving photoelectric effect. The graphs A, B,C and D relate to a light beam having different wavelengths. Select the correct alternative.
View SolutionWhen a hydrogen atom is excited from ground state to first excited state then
View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
View SolutionChoose the correct statement:
View SolutionThe radioactivity of a sample is R1 at a time T1 and R2 at time T2. If the half-life of the specimen is T, the number of atoms that have disintegrated in the time (T2−T1) is proporational to
View SolutionThe activity of a radioactive substance is R0 at t=0, R1 at t=t and R2 at t=2t. The decay constant of this species is/are given by:
View SolutionRadioactivity of a sample at T1 time is R1 and at time T2 is R2. If half-life of sample is T, then in time (T2−T1), the number of decayed atoms is proportional to
View SolutionIf Nt=Noeλt then number of disintefrated atoms between t1 to t2(t2>t1) will ve :-
View SolutionLet R_(t) represents activity of a sample at an insant and N_(t) represent number of active nuclei in the sample at the instant. T_(1//2) represents the half life. {:(,"Column I",,"Column II"),((A),t=T_(1//2),(p),R_(t)=(R_(0))/(2)),((B),t=(T_(1//2))/(ln2),(q),N_(0)-N_(t)=(N_(0))/(2)),((C),t=(3)/(2)T_(1//2),(r),(R_(t)-R_(0))/(R_(0)) = (1-e)/(e)),(,,(s),N_(t)=(N_(0))/(2sqrt(2))):}
View SolutionThe radioactivity of sample is R_(1) at a time T_(1) and R_(2) at a time T_(2) . If the half -life of thespeciman is T, the number of atoms that have disintegrated in the time (T_(2)-T_(1)) is equal to :
View Solutionएक प्रतिदर्श की रेडियो सक्रियता समय t1 पर R1 तथा समय t2 पर R2 है | यदि प्रतिदर्श की अर्द्धआयु T है तो समय (t2−t1) में क्षय होने वाले नाभिकों की संख्या अनुक्रमानुपाती होगी :
View SolutionActivity of a radioactive substance is Rt at time t1 and R2 at time t2(t2>t1). Then the ratio R2R1 is
View SolutionRadioactivity of a sample at T1 time is R1 and at time T2 is R2. If half-life of sample is T, then in time (T2−T1), the number of decayed atoms is proportional to
View SolutionLet R_(t) represents activity of a sample at an insant and N_(t) represent number of active nuclei in the sample at the instant. T_(1//2) represents the half life. {:(,"Column I",,"Column II"),((A),t=T_(1//2),(p),R_(t)=(R_(0))/(2)),((B),t=(T_(1//2))/(ln2),(q),N_(0)-N_(t)=(N_(0))/(2)),((C),t=(3)/(2)T_(1//2),(r),(R_(t)-R_(0))/(R_(0)) = (1-e)/(e)),(,,(s),N_(t)=(N_(0))/(2sqrt(2))):}
View Solution
