If Nt=Noeλt then number of disintegrated atoms between t1 to t2(t2>t1) will ve :-
No[eλt2−eλt3]
No[−eλt2−e−λt1]
No[e−λt1−e−λt2]
None of these
The correct Answer is:C
To find the number of disintegrated atoms between time t1 and t2, we start with the given equation for the number of active radioactive atoms at time t:
N(t)=N0e−λt
where:
- N(t) is the number of active atoms at time t,
- N0 is the initial number of atoms,
- λ is the decay constant.
Step 1: Calculate the number of active atoms at t2
Using the formula, the number of active atoms at time t2 is:
N(t2)=N0e−λt2
Step 2: Calculate the number of active atoms at t1
Similarly, the number of active atoms at time t1 is:
N(t1)=N0e−λt1
Step 3: Calculate the number of disintegrated atoms at t2
The number of disintegrated atoms at time t2 is the initial number of atoms minus the number of active atoms at t2:
Disintegrated atoms at t2=N0−N(t2)=N0−N0e−λt2
This simplifies to:
Disintegrated atoms at t2=N0(1−e−λt2)
Step 4: Calculate the number of disintegrated atoms at t1
Similarly, the number of disintegrated atoms at time t1 is:
Disintegrated atoms at t1=N0−N(t1)=N0−N0e−λt1
This simplifies to:
Disintegrated atoms at t1=N0(1−e−λt1)
Step 5: Calculate the number of disintegrated atoms between t1 and t2
To find the number of disintegrated atoms between t1 and t2, we subtract the number of disintegrated atoms at t1 from the number at t2:
Disintegrated atoms between t1 and t2=Disintegrated atoms at t2−Disintegrated atoms at t1
Substituting the expressions we found:
=N0(1−e−λt2)−N0(1−e−λt1)
This simplifies to:
=N0e−λt1−N0e−λt2
Factoring out N0:
=N0(e−λt1−e−λt2)
Final Answer
Thus, the number of disintegrated atoms between t1 and t2 is:
N0(e−λt1−e−λt2)
- N(t) is the number of active atoms at time t,
- N0 is the initial number of atoms,
- λ is the decay constant.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-02
19 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column
1 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES
29 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
The half-life of a radioactive sample is T. If the activities of the sample at time t1 and t2 (t1<t2) and R1 and R2 respectively, then the number of atoms disintergrated in time t2−t1 is proportional to
View Solution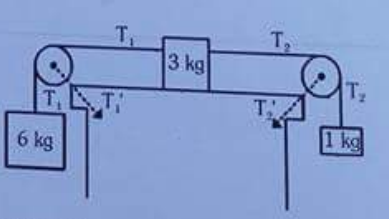
Calculate a, T1,T2,T1'&T2'.
View SolutionThe radioactivity of a sample is R1 at a time T1 and R2 at time T2. If the half-life of the specimen is T, the number of atoms that have disintegrated in the time (T2−T1) is proporational to
View SolutionThe radioactive of a sample is R1 at a time T1 and R2 at a time T2. If the half-life of the specimen is T, the number of atoms that have disintegrated in the time (T2−T1) is equal ton(R1−R2)Tln4. Here n is some integral number. What is the value of n?
View SolutionShown below are the black body radiation curves at temperature T1 and T2(T2>T1). Which of the following plots is correct?
View SolutionThe activity of a radioactive susbtance is R1 at time t1 and R2 at time t2(>t1). its decay constant is λ. Then, number of atoms decayed between time interval t1 and t2 are
View SolutionFind the value of ln(∫10et2+t(2t2+t+1)dt)
View SolutionUsing the equation N=N0e−λt, obtain the relation between half-life (T) and decay constant (λ) of a radioactive substance.
View SolutionThe numbers 1,3,6,10,15,21,28... are called triangular numbers. Let tn denote the nth triangular number such that tn=tn−1+n,∀n≥2.
The number of positive integers lying between t100 and t101 are:
View SolutionActivity of a radioactive substance is A1 at time t1 and A2 at time t2(t2>t1) , then the ratio of fA2A1 is:
View Solution
