If a,b,c,d are positive real numbers such that a3=a+b4=a+b+c5=a+b+c+d6, then ab+2c+3d is:-Solution in Gujrati
1/2
1
2
Not determinable
The correct Answer is:A
Step by step video & image solution for If a,b,c,d are positive real numbers such that (a)/(3) = (a+b)/(4)= (a+b+c)/(5) = (a+b+c+d)/(6), then (a)/(b+2c+3d) is:- by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - RACE 5
10 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - RACE 6
7 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - RACE 3
10 Videos
- LOGARITHMSBOOK - ALLEN CHAPTER - LOGARITHMSEXERCISE - All Questions
103 Videos
- SEQUENCE AND PROGRESSIONBOOK - ALLEN CHAPTER - SEQUENCE AND PROGRESSIONEXERCISE - Exercise (JA)
10 Videos
Similar Questions
If BC passes through centre of the circle, then the area of the shaded region in the given figure is:
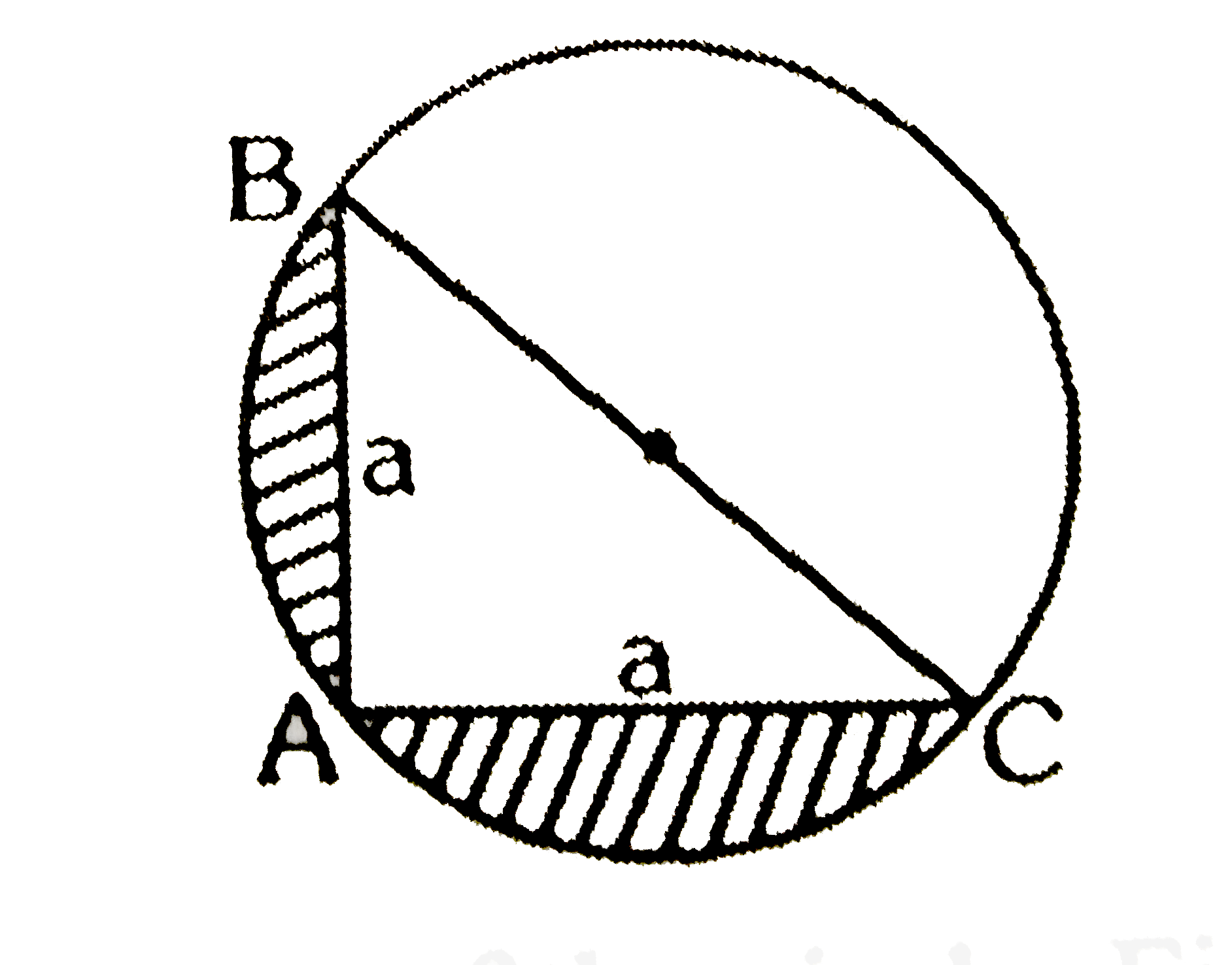
View SolutionIf M is even prime number,N is least prime number of two digits & P is greatest prime number of two digits, then P+NM is dividible by-
View SolutionValue of (x4+x2+1)(x2−x+1)−(x+1)2 is
View SolutionThe value of N satisfying (log)a[1+(log)b {1+(log)c(1+(log)pN)}]=0 is-
a.4 b. 3 c.2 d. 1
View SolutionThe minimum value of the sum of real numbers a−5,a−4,3a−3,1,a8 and a10 with a>0 is
View SolutionMinimum value of (b+c)/a+(c+a)/b+(a+b)/c (for real positive numbers a,b,c) is (a)1 (b)2 (c)4 (d)6
View SolutionIf a,b,c,d are positive real number with a+b+c+d=2, then M=(a+b)(c+d) satisfies the inequality
View SolutionIf a, b, c are real and x^3-3b^2x+2c^3 is divisible by x -a and x - b, then (a) a =-b=-c (c) a = b = c or a =-2b-_ 2c (b) a = 2b = 2c (d) none of these
View SolutionIf a,b,c,d are four positive numbers then (A) ((a)/(b)+(b)/(c))((c)/(d)+(d)/(c)) =4.sqrt((a)/(c))(C)(a)/(b)+(b)/(c)+(c)/(d)+(d)/(e)+(e)/(a) =(1)/(5)
View SolutionIf a,b,c,d are four positive numbers that (i)((a)/(b)+(b)/(c))((c)/(d)+(d)/(e)) =4.sqrt((a)/(c))(iii)(a)/(b)+(b)/(c)+(c)/(d)+(d)/(e)+(e)/(a) =(1)/(5)
View SolutionIf (2a+3b)(2c−3d)=(2a−3b)(2c+3d) , then ab=cd (b) ad=cb (c) ab=dc (d) ba=cd
View SolutionIf (a+b+2c+3d)(a−b−2c+3d)=(a−b+2c−3d)(a+b−2c−3d) , then 2bc is equal to 32 (b) 3a2d (c) 3ad (d) a2d2
View SolutionIf a, b, c are distinct positive real numbers such that a+1b=4,b+1c=1,c+1d=4 and d+1a=1, then
View SolutionIf a,b,c,d are positive real numbers such that a+b+c+d=12 , then M=(a+b)(c+d) satisfies the relation :
View SolutionMinimum value of (b+c)/a+(c+a)/b+(a+b)/c (for real positive numbers a,b,c) is (a)1 (b)2 (c)4 (d)6
View SolutionIf a, b, c, d are are positive real numbers such that a+b+c+d=2, then M=(a+b)(c+d) satisfies the relation is:
View Solution
