If velocity of a particle is given by v=(2t+3)m/s. Then average velocity in interval 0≤t≤1 s is : Solution in Gujrati
7/2 m/s
9/2 m/s
4 m/s
5 m/s
The correct Answer is:D
lmin=√(100−2×50)2+(200−4×50)2=0
Topper's Solved these Questions
- BASIC MATHSBOOK - ALLEN CHAPTER - BASIC MATHSEXERCISE - Comprehension 3
3 Videos
- BASIC MATHSBOOK - ALLEN CHAPTER - BASIC MATHSEXERCISE - Comprehension 4
3 Videos
- BASIC MATHSBOOK - ALLEN CHAPTER - BASIC MATHSEXERCISE - Comprehension 1
5 Videos
- AIIMS 2019BOOK - ALLEN CHAPTER - AIIMS 2019EXERCISE - PHYSICS
39 Videos
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
Similar Questions
Magnitude of slope of the shown graph.
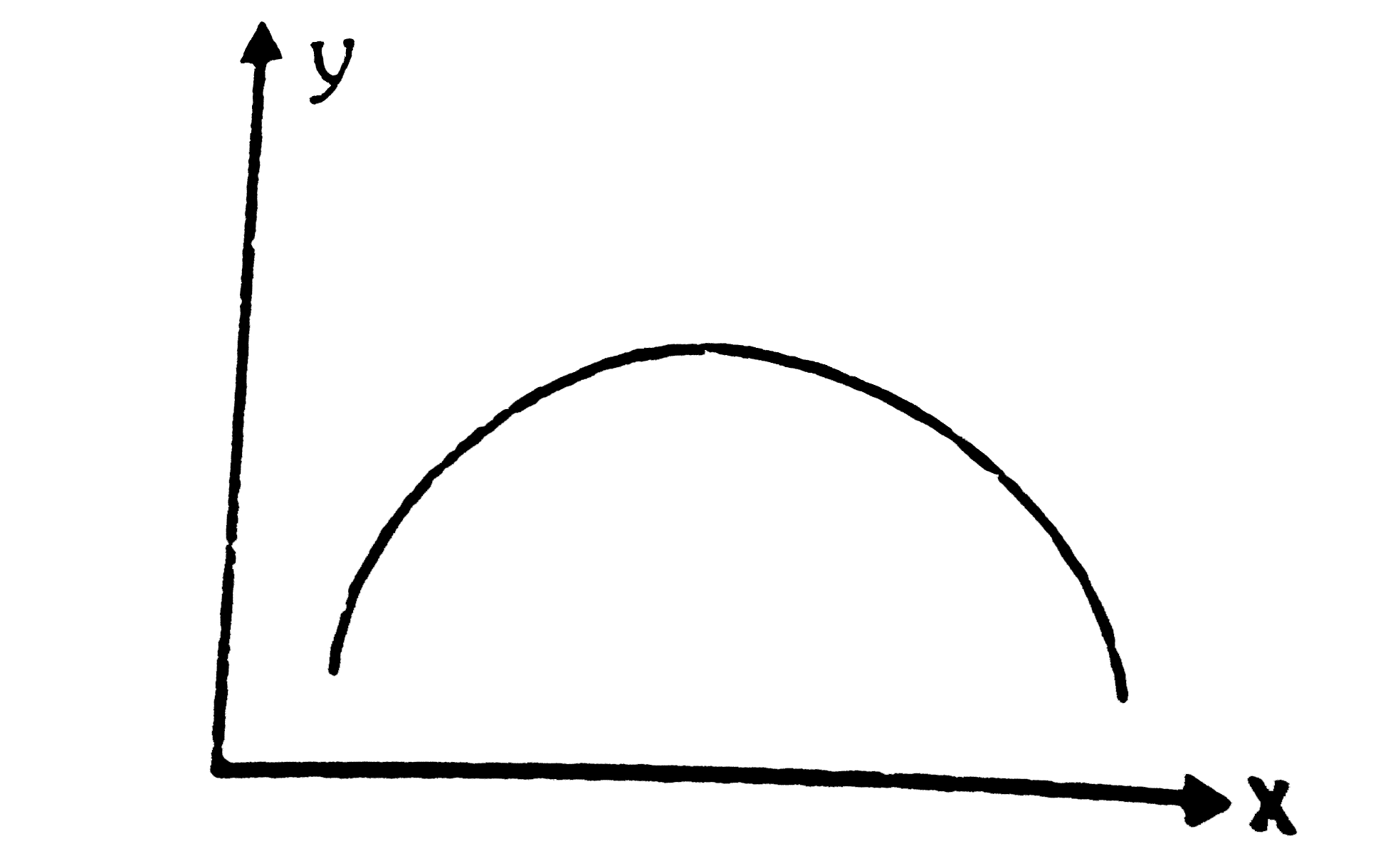
View SolutionWhich one of the following statement is false?
View SolutionThe greater value of the function −5sinθ+12cosθ is
View SolutionA simple pendulum executing SHM in a straight line has zero velocity at 'A' and 'B' whose distances form 'O' in the same line OAB are 'a' and 'b'. If the velocity half wat between them is 'v' then its time period is
View SolutionA ball of mass (m)0.5kg is attached to the end of a string having length (L)0.5m. The ball is rotated on a horizontal circular path about vertical axis. The maximum tension that the string can bear is 324N. The maximum possible value of angular velocity of ball (radian/s) is -
 .
.
View SolutionThe position of a particle moving on the x-axis is given by x=t^(3)+4t^(2)-2t+5 where x is in meter and t is in seconds (i) the velocity of the particle at t=4 s is 78 m//s (ii) the acceleration of the particle at t=4 s is 32 m//s^(2) (iii) the average velocity during the interval t=0 to t=4 s is 30 m//s (iv) the average acceleration during the interval t=0 to t=4 s is 20 m//s^(2)
View SolutionThe velocity of a particle is given by v=(2t2−4t+3)m/s where t is time in seconds. Find its acceleration at t=2 second.
View SolutionIf velocity of a particle is given by v=2t2−2 then find the acceleration of particle at t = 2 s.
View SolutionThe velocity time relation of a particle is given by v = (3t^(2) -2t-1) m//s Calculate the position and acceleration of the particle when velocity of the particle is zero . Given the initial position of the particle is 5m .
View SolutionVelocity of a particle is given as v=(2t2−3)m/s. The acceleration of particle at t = 3s will be :
View SolutionA particle travels A to M along a straight line with a velocity of 8 m/s and M to A with a velocity of 2 m/s,
then the average velocity for the whole journey is –
View SolutionThe velocity of a particle is given by the equation, v=2t2+5cms−1 . Find
the average acceleration during the time interval betweent1=2sandt2=4s .
View Solutionकिसी कण का त्वरण a=3t2+2t+2m/s2 द्वारा दिया जाता है, यहाँ t समय है। यदि कण t=0 पर v= 2 मी/सै से चलना प्रारम्भ करता है, तब 2 सैकण्ड पश्चात् कण का वेग होगा
View SolutionVelocity of a particle is given byv=(3t2+2t)ms. Find its average velocity between t = 0 to t=3s and also find its acceleration at t = 3s. Motion of the particle is in one dimension.
View Solutionयदि एक कण का वेग सकीकरण v=(2t+3)m/s से दिया जाता है तो अंतराल 0≤t≤1s से औसत वेग है
View Solutionएक कण 20 सेकण्ड तक 3 m/s वेग से तथा फिर 20 सेकण्ड तक 4m/s वेग से और अगले 20 सेकण्ड तक 5 m/s के वेग से गति करता है। कण का औसत वेग क्या होगा ?
View Solution
