A ball is projected vertically up wards with a velocity of 100 m/s. Find the speed of the ball at half the maximum height. $(g = 10 m / s^{2})$ Solution in Gujrati

Video Solution

Text Solution

Verified by Experts

Ships a and b are moving with same speed $20 k m / h$ in the direction shown in figure. It is a two dimensional, two body problem with zero acceleration. Let us find ${\to v}_{B A}$

${\to v}_{B A} = {\to v}_{B} - {\to v}_{A}$
Here, $| {\to v}_{B A} | = \sqrt{{(20)}^{2} + {(20)}^{2}} = 20 \sqrt{2} k m / h$
i.e., ${\to v}_{B A}$ is $20 \sqrt{2} k m / h$ at an angle of $45^{\circ}$ from east
towards north. Thus, the given problem can be simplified as :

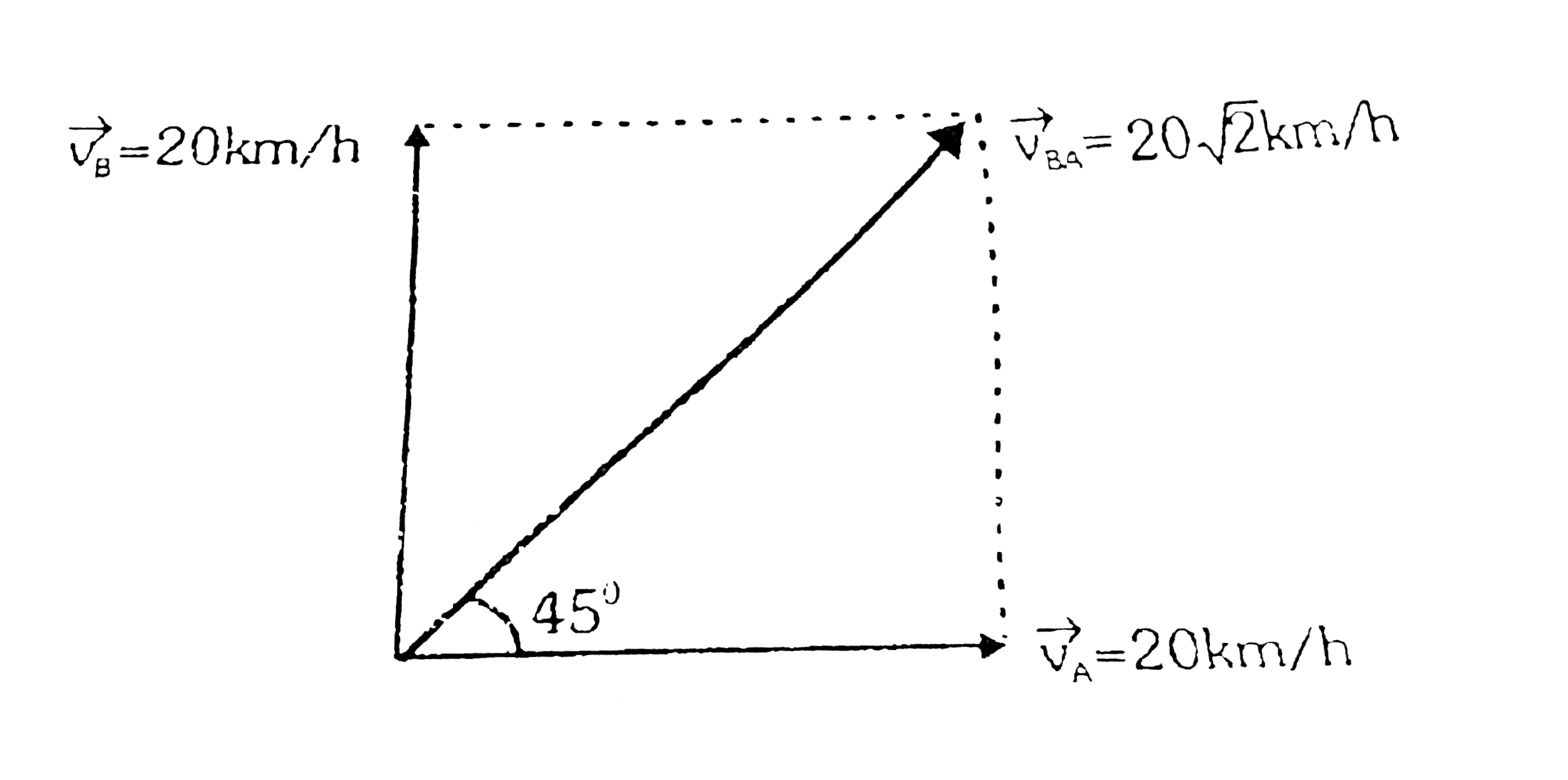
A is at rest and B is moving with ${\to v}_{B A}$ in the direction shown in figure.
Therefore, the minimum distance between the two is ltbtgt $s_{min} = A C = A B {sin 45}^{\circ} = 10 (\frac{1}{\sqrt{2}}) k m = 5 \sqrt{2} k m$
and the desired time is $t = \frac{B C}{| {\to v}_{B A} |} = \frac{5 \sqrt{2}}{20 \sqrt{2}} = \frac{1}{4} h = 15$ min

|

Updated on:21/07/2023

Class 12 PHYSICS MOTION IN A PALNE

Topper's Solved these Questions

Similar Questions

ALLEN -MOTION IN A PALNE-SOLVED EXAMPLE

Home
Ask Doubt
Profile