If the area of a triangle of sides is equal to , then find the acute angle between .
Text Solution
The correct Answer is:
If vector are sides of a triangle then its area
|
Topper's Solved these Questions
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLEN CHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - TRIGONOMETRY
7 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLEN CHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - GEOMETRY
7 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLEN CHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - BEGINNER S BOX 11
4 Videos
- CENTRE OF MASSBOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B
19 Videos
Similar Questions
Knowledge Check
If →a.→b =ab then the angle between →a and →b is
Aπ/2BπCzeroDany angle between zero and πTwo vectors →A and →B have equal magnitudes. If magnitude of →A+→B is equal to n times the magnitude of →A−→B, then the angle between →A and →B is :-
Acos−1(n−1n+1)Bcos−1(n2−1n2+1)Csin−1(n−1n+1)Dsin−1(n2−1n3+1)If ∣∣→A×→B∣∣=∣∣→A⋅→B∣∣, then the angle between →Aand→B will be :
A30∘B45∘C60∘D75∘
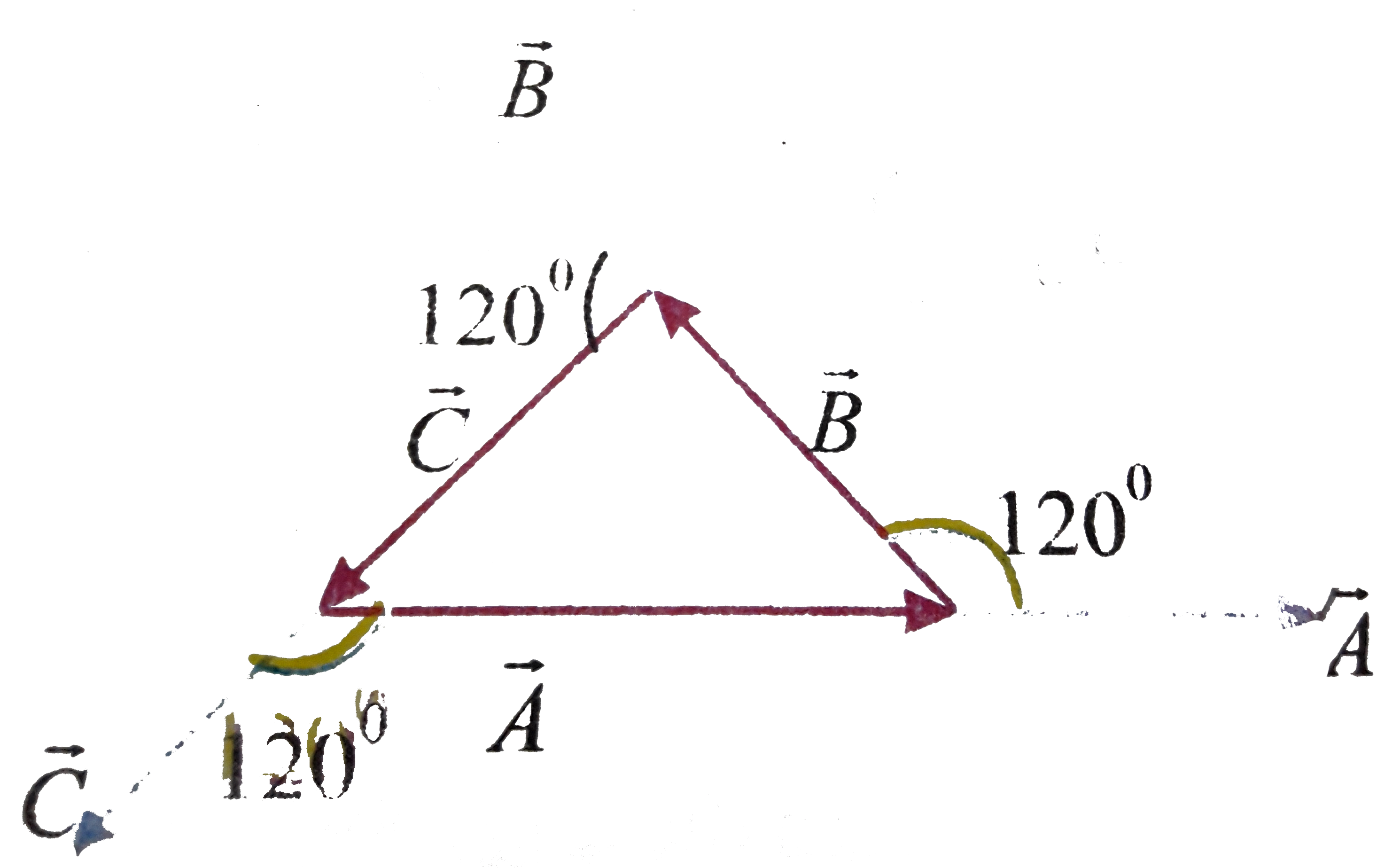
If →A,→B,→C represents the three sides of an equilateral triangle taken in the same order then find the angle between
(i) →A and →B (ii) →B and →C (iii) →A and →C.
View SolutionTwo vectors →Aand→B have equal magnitudes.If magnitude of (→A+→B) is equal to n times of the magnitude of (→A−→B) then the angle between →Aand→B is :-
View SolutionIf ∣∣→A+→B∣∣=∣∣→A−→B∣∣, then the angle between →Aand→B will be
View SolutionIf ∣∣→A+→B∣∣=∣∣→A−→B∣∣, then the angle between →Aand→B will be
View SolutionIf ∣∣→A×→B∣∣=→A.→B then the angle between →Aand→B is :
View Solution
