Column IColumn II(A)Dimensional variable(p)π(B)Dimensionless variable(q)Force(C)Dimensional constant(r)Angle(D)Dimensionless constant(s)Gravitational constantSolution in Gujrati
The correct Answer is:(i) (b), (ii) (c ), (iii) (d), (iv)(a)
Step by step video & image solution for {:(,"Column I",,"Column II"),((A),"Dimensional variable",(p),pi),((B),"Dimensionless variable",(q),"Force"),((C),"Dimensional constant",(r),"Angle"),((D),"Dimensionless constant",(s),"Gravitational constant"):} by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - BEGINNER S BOX-3
3 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - BEGINNER S BOX-4
4 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - BEGINNER S BOX-1
2 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Question
1 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
Write the following in scientific notation :
(a) 3256 g
(b) 0.0010 g
(c ) 50000 g
(d) 0.3204
View SolutionRound off the following numbers as indicated:
(a) 25.653 to 3 digits (b) 4.996×105 to 3 digits (c) 0.6995 to 1 digit
(d) 3.350 to 2 digits (e) 0.03927 kg to 3 digits (f) 4.085×108s to 3 digits
View SolutionThe value of Gravitational constant G in MKS system is 6.67×10−11N−m2/kg2
What will be its value in CGS system ?
View SolutionA simple pendulum of length l and bob mass m is hanging in front of a large nonconducting sheet having surface charge density σ. If suddenly a charge +q is given to the bob & it is released from the position shown in figure. Find the maximum angle through which the string is deflected from vertical .
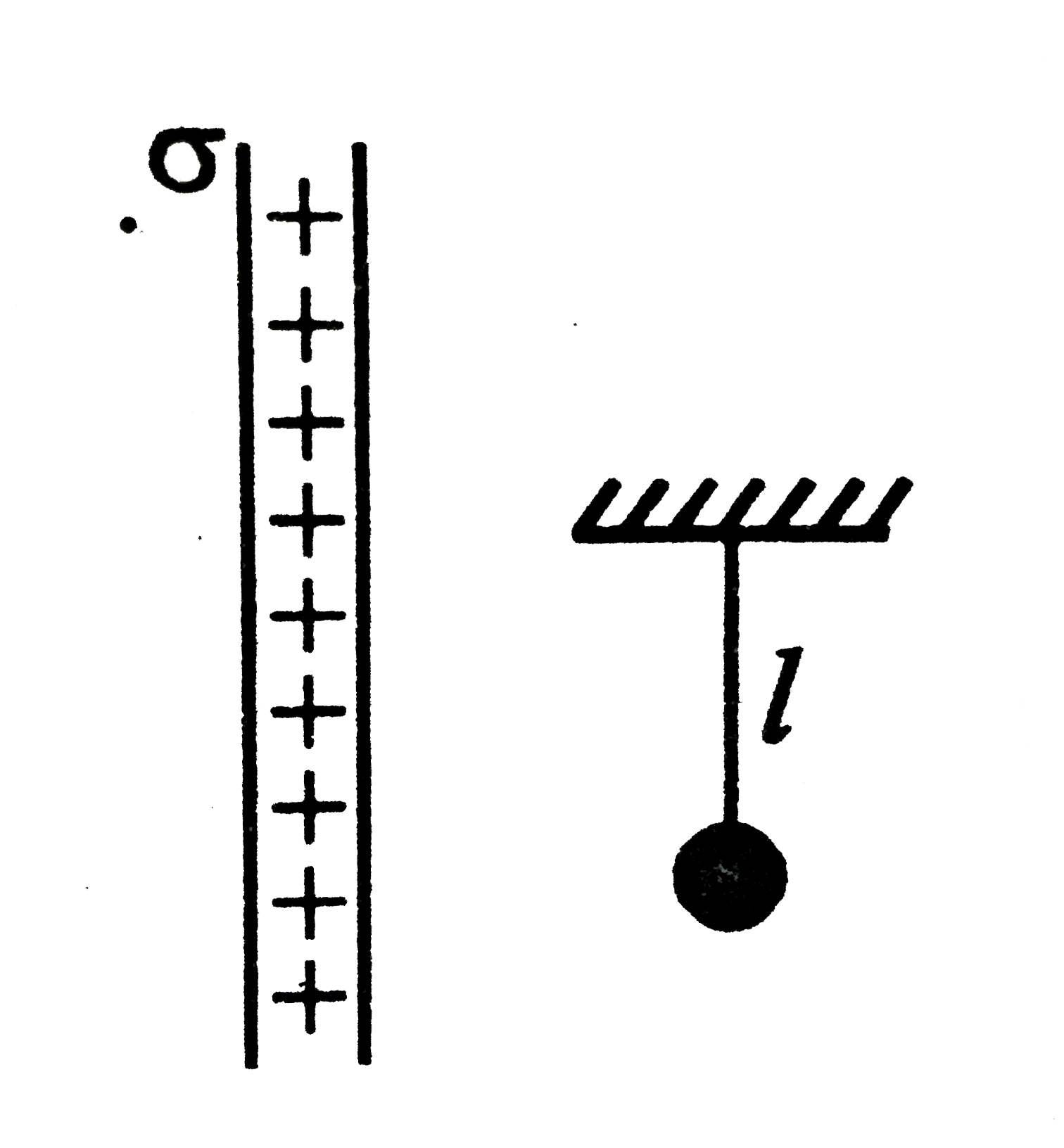 .
.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionAssertion: Dimensional constant are the quantites whose value are constant. Reason: Dimensional constant are Dimensionless.
View SolutionGive two examples each of dimensionless constants and dimensional variavles.
View Solution{:(,"Column I",,"Column II"),((A),"Dimensional variable",(p),pi),((B),"Dimensionless variable",(q),"Force"),((C),"Dimensional constant",(r),"Angle"),((D),"Dimensionless constant",(s),"Gravitational constant"):}
View SolutionSTATEMENT-1 Dimensional constants are the quantities whose values are constant STATEMENT 2 Quantities which are dimensionless are known as dimensional constants
View SolutionSTATEMENT-1: Dimensional constants are the quantities whose values are constant STATEMENT-2: Dimensional constants are dimensionless STATEMENT-3 Universal gravitational constant (G) is a dimensional constant
View SolutionIf velocity, force and time are taken as the fundamental quantities, them using dimensional analysis choose the correct dimensional formula for mass among the following. [K is a dimensionless constant]
View SolutionGive examples of dimensional constants and dimensionless constants.
View SolutionGive examples of dimensional constants and dimensionless constants.
View Solution.(A): The value of dimensionless constants or proportionality constants cannot be found by dimensional methods.
(b) : The equations containing trigonometrical, exponential and logarithmic functions cannot be analysed by dimensional methods.
View Solution(A) : The value of dimensionless constants or proportionality constants cannot be found by dimensional methods.
(B) : The equations containing trigonometrical, exponential and logarithmic functions cannot be analysed by dimensional methods.
View SolutionWhat is the difference between a dimensional constant and a dimensionless constant?
View Solution
