Depending on in which quadrant an angle θ lies, functions cosθ and sinθ may be positive or negative. In the second column of the given lable are specified whether these functions are positive or negative and in the first column are specified quadrants.
Column-IColumn-II(A) First(P)sinθ is positive(B) Second(Q)sinθ is negative(C) Third(R)cosθ is negative(D) Fourth(S)tanθ and sinθboth are negative(T)secθ is negative and sinθ is positiveSolution in Gujrati
The correct Answer is:(A)→(P);(B)→(P,R,T);(C)→(Q,R);(D)→(Q,S)
Step by step video & image solution for Depending on in which quadrant an angle theta lies, functions costheta and sintheta may be positive or negative. In the second column of the given lable are specified whether these functions are positive or negative and in the first column are specified quadrants. {:("Column-I","Column-II"),((A)" First",(P)sintheta" is positive"),((B)" Second",(Q)sintheta" is negative"),((C)" Third",(R)costheta" is negative"),((D)" Fourth",(S)tantheta" and "sintheta"both are negative"),(,(T)sectheta" is negative and "sintheta" is positive"):} by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Differentiation)
40 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Integration)
12 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - PHYSICS
12 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLEN CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
For a straight line y=43x−4. Choose correct alternate(s)
View SolutionFind the value of x1, so that ∫x10ydx=5
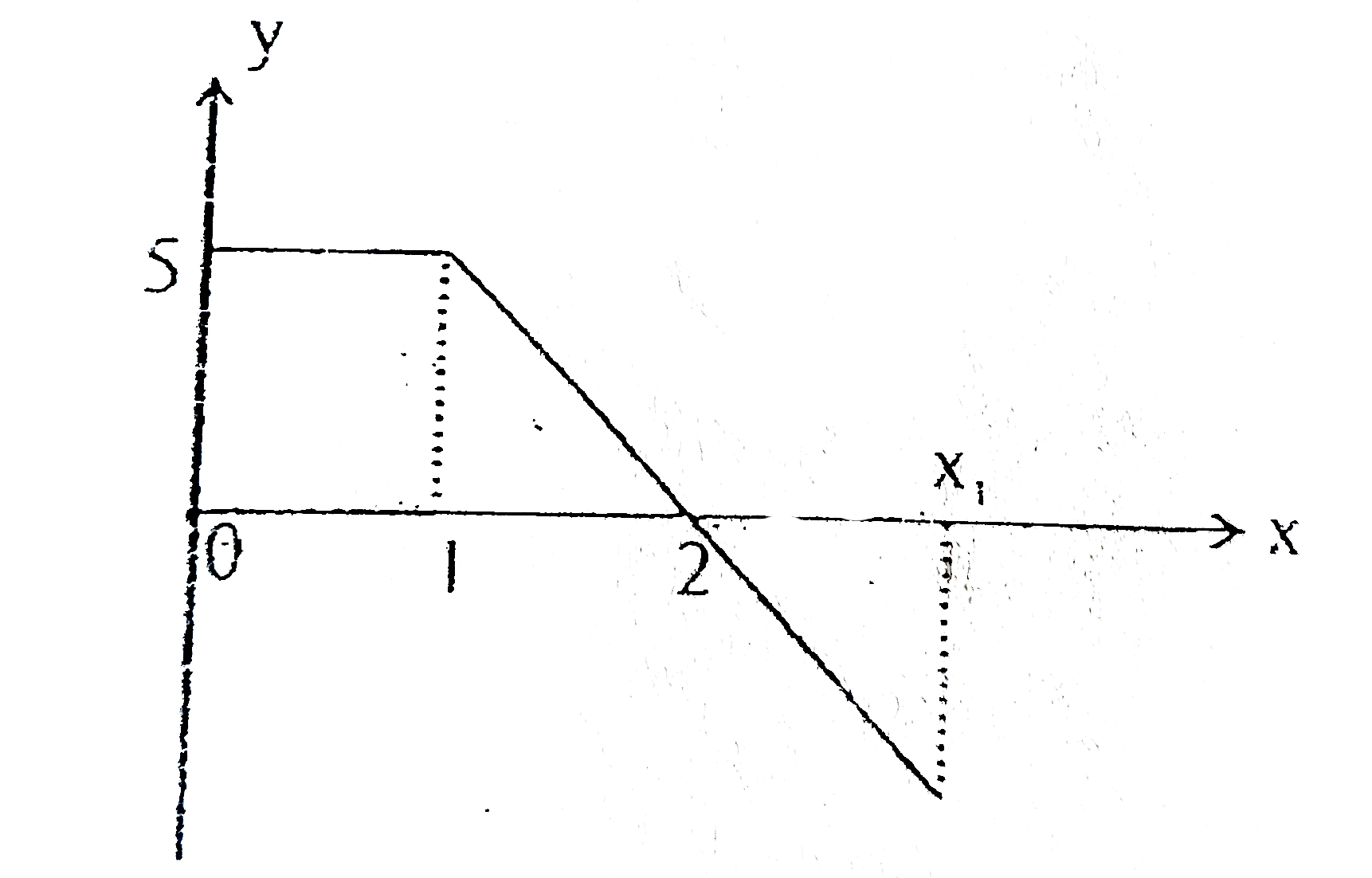
View SolutionThe graph shown the extension of is wire of length 1 m suspended from the top of a roof at one end and with a load W connected to the other end. If the cross sectional area of the wire is 1mm2, then the Young's modulus of the material of the wire.

View SolutionAssertion: The driver in a vechicle moving with a constant speed on a straight road is in a non-inertial frame of referance.
Reason: A reference frame in which Newton's laws of motion are applicable is non-inertial.
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionIf theta lies in the second quadrant, then the value sqrt(((1-sintheta)/(1+sintheta)))+sqrt(((1+sintheta)/(1-sintheta)))
View SolutionIf tantheta=(24)/(7) and sintheta is negative then value of costheta will be
View SolutionLet X={θ∈[0,2π]:sinθ=cosθ}
Assertion (A) : The number of elements in X is 2.
Reason ( R ) : sinθandcosθ are both negative both in second and fourth quadrants.
View Solutioncosθ⋅cscθ−sinθ⋅secθcosθ+sinθ
View Solutioncosθ[cosθsinθ−sinθcosθ]+sinθ[sinθ−cosθcosθsinθ]=
View SolutionIf cosθ=−34andtanθ is negative, the value of sinθ is
View SolutionIf sinθ=2425 and θ lies in II quadrant, then secθ+tanθ=...........
View SolutionIf sinθ=2425 and θ lies in II quadrant, then secθ+tanθ= _________
View SolutionFind the quadrant in which θ lies if
(i)cos θ is negative but sin θ is positive
(ii)sin θ is negative but tan θ is positive
(iii) cos θ is negative and sin θ is negative
(iv) cos θ and cot θ are both negative
(v) sec θ is positive but cosec θ is negative
View SolutionIf tanθ=3 and θ lies in third quadrant then sinθ=
View SolutionIf sinθ+cosθ=0andθ lies in the fourth quadrant, find sinθandcosθ
View Solution
