Evaluate the following indefinite integrals.
(a)∫dx√x
(b)∫dx(2x+3)
(c)∫sin(2πx+30∘)dx
(d)∫sinxcosxdx
(e)∫(3x3−5x2+2)dx
(f)∫(2e−x/3dxSolution in Gujrati
The correct Answer is:(a)2√x+C
(b)12ln(2x+3)+C
(c)−12πcos(2πx+30∘)+C
(d)−14cos2x+C
(e)3x44−5x35+2x+C
(f)−6e−x/3+C
Step by step video & image solution for Evaluate the following indefinite integrals. (a)int(dx)/(sqrt(x)) (b)int(dx)/((2x+3)) (c)intsin(2pix+30^(@))dx (d)intsinxcosxdx (e)int(3x^(3)-5x^(2)+2)dx (f)int(2e^(-x//3)dx by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Graphs))
28 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Co-ordinate & Algebra)
40 Videos
- RACEBOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Differentiation)
40 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLEN CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
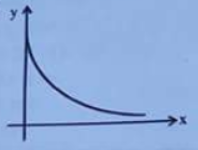
Which of the following equation is best representation of following graph's?
View SolutionFind the value of :-
(i)√4
(ii)√9
(iii)√16
(iv)sqrt(36)
View SolutionFor a straight line y=43x−4. Choose correct alternate(s)
View SolutionAssertion: The driver in a vechicle moving with a constant speed on a straight road is in a non-inertial frame of referance.
Reason: A reference frame in which Newton's laws of motion are applicable is non-inertial.
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionFind the integral∫√x(3x2+2x+3)dx
View SolutionFind the following integrals.
1. ∫3x7dx 2. ∫4√xdx 3. ∫dx√x 4. ∫(x3−5x2+7x−11)dx
5. ∫(3√x−13√x)dx 6. ∫3xdx 7. ∫5x2dx 8. ∫12x3dx
9. ∫0.4x4dx 10. ∫12x3dx 11. ∫15t2dt 12. ∫dthηθ
13. ∫(4x2−5x+1)dx 14. ∫(3x4−5x3)dx 15. ∫x(8x−12)dx 16. ∫6x2(x2+x)dx
View SolutionEvaluate : (i) ∫cos2x dx (ii) ∫e(5x+3)dx (iii) ∫sec2(3x+5)dx (iv) ∫sin3xdx
View Solutionनिम्नलिखित समाकलों (Integrals) का मान ज्ञात कीजिए-
(a) ∫2xdx (b) ∫3xdx (c) ∫bxdx (d) ∫bx+adx
View Solutionनिम्नलिखित समाकलों (Integrals) का मान ज्ञात कीजिए-
(a) ∫sec2xcosec2xdx (b) ∫secx(secx+tanx)dx
(c) ∫[2x2−3sinx+5√5]dx (d) ∫(2x−3cosx+ex)dx
View Solutionनिम्नलिखित समाकलों (Integrals) का मान ज्ञात कीजिए-
(a)∫x3+5x2−4x2dx (b) ∫dtt
(c) ∫x3+3x+4√xdx (d) ∫dxx√x
View Solutionनिम्नलिखित का मान ज्ञात कीजिए -
(a) ∫2xn−1xn+3dx (b) ∫4x3(x4+5)5dx
(c) ∫x9−4x2dx (d) ∫e√x√xdx
View Solutionनिम्नलिखित समाकल का मान ज्ञात कीजिए -
(a) ∫xsin3xdx (b) ∫xsin2xdx
(c) ∫xtan2xdx (d) ∫(x−3)ex(x−1)3dx
View Solutionनिम्नलिखित समाकलों के मान ज्ञात कीजिए-
(a) ∫dxx2−9 (b) ∫dx24−6x2
(c) ∫dxx2−7 (d) ∫dx(x+3)2−4
(e) ∫2xx2+3x+2dx
View Solutionनिम्नलिखित समाकलों के मान ज्ञात कीजिए-
(a) ∫2xx2+3x+2dx (b) ∫(2x+1)dx(x+1)(x−2)
(c) ∫5xdx(x+1)(x2−4) (d) ∫3xdx(x−2)(x+1)
View Solutionसमाकलन करें
(a)∫5dx
(b)∫2x3dx
(c)∫(sin2x)dx
(d)∫cos2xdx
View Solution
