Dimension of electrical resistance is :-
ML2T−3A−1
ML2T−3A−2
ML3T−3A−2
ML−1L3T3A2
The correct Answer is:B
To find the dimension of electrical resistance, we can start from Ohm's Law, which states that:
V=IR
Where:
- V is the voltage (potential difference),
- I is the current,
- R is the resistance.
From this equation, we can express resistance R as:
R=VI
Step 1: Determine the dimensions of voltage V
Voltage V can be expressed in terms of work done W and charge Q:
V=WQ
The dimension of work W is given by:
W=Force×Distance=(MLT−2)×L=ML2T−2
The dimension of charge Q is represented as:
Q=I×T
Where I is the current. Therefore, the dimension of charge Q is:
Q=AT
Now substituting these into the equation for voltage:
V=ML2T−2AT
This simplifies to:
V=ML2T−3A−1
Step 2: Determine the dimensions of current I
The dimension of current I is simply:
I=A
Step 3: Substitute dimensions of V and I into the equation for R
Now substituting the dimensions of V and I into the equation for resistance:
R=VI=ML2T−3A−1A
This simplifies to:
R=ML2T−3A−2
Conclusion
Thus, the dimension of electrical resistance R is:
(R)=ML2T−3A−2
- V is the voltage (potential difference),
- I is the current,
- R is the resistance.
Similar Questions
Which of the following combinations has the dimension of electrical resistance (∈0 is the permittivity of vacuum and μ0 is the permeability of vacuum)?
View SolutionWhich of the following combinations has the dimension of electrical resistance (∈0 is the permittivity of vacuum and μ0 is the permeability of vacuum)?
View SolutionUsing B0=(μ0)H0, find the ratio (E0H0) for a plane electromagnetic wave propagating through vacuum. Show that it has the dimensions of electric resistance. This ratio is a universal constant called the impedance of free space.
View SolutionDimensions of electrical resistence are
View SolutionThe dimensions of thermal resistance are
View SolutionThe dimensions of specific resistance are
View SolutionThe dimension of 1/CR is, where C is capacitance and R is electrical resistance :
View SolutionWhat are the dimensions of electrical permittivity?
View SolutionWhat are the dimensions of electrical permittivity?
View SolutionFigure represents a load consisting of three identical resistances connected to an electric enrgy source of emf 12 V and internal resistance 0.6Ω. The ammeter reads 2A. The magnitude of each resistance is
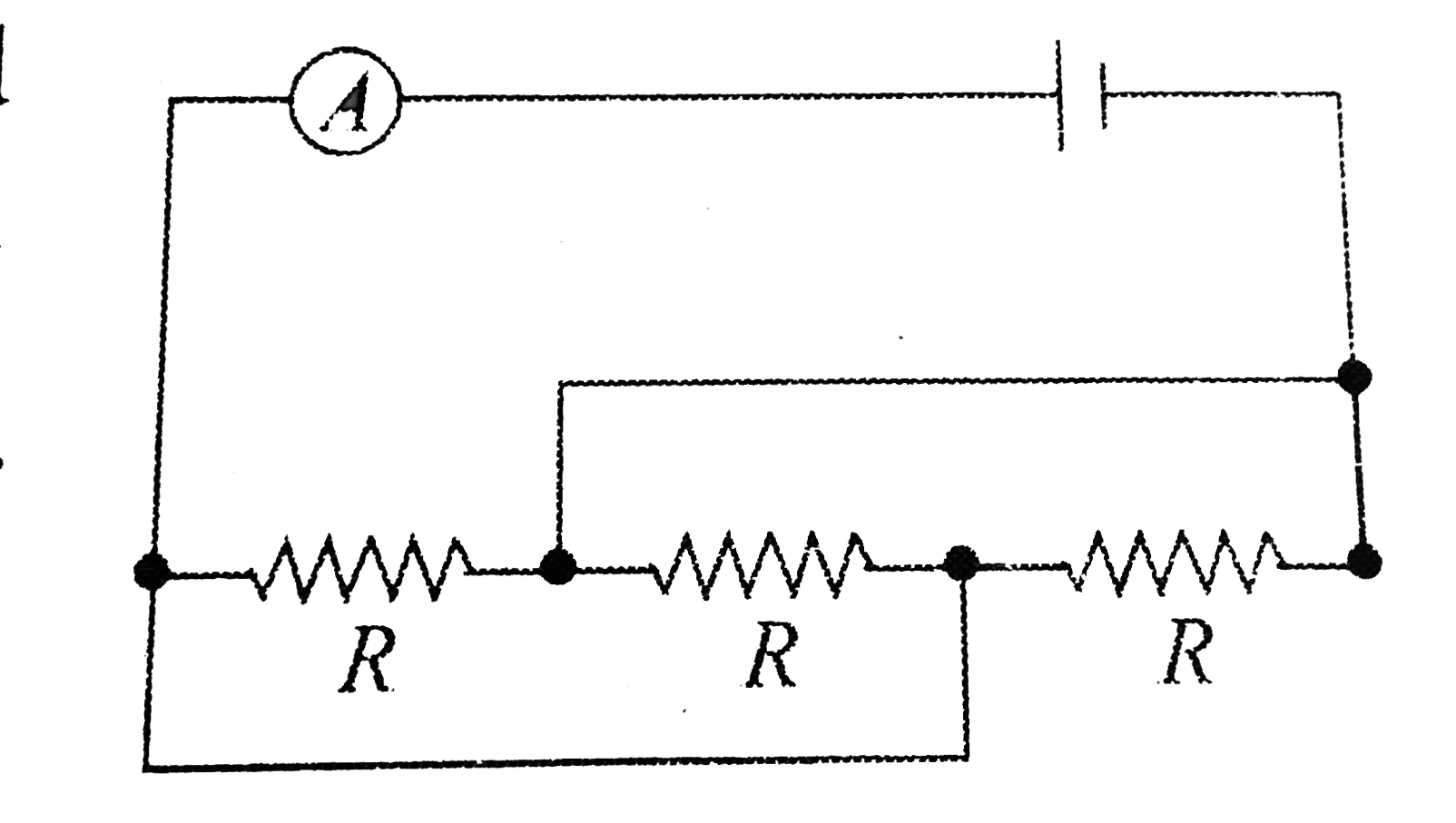
View Solution
