A long cylindrical wire carrying current of 10A has radius of 5mm find its its magnetic field induction at a point 2mm from the centre of the wire
16×10−4T
2.4×10−4T
3.2×10−4T
0.8×10−4T
The correct Answer is:A
To find the magnetic field induction at a point 2 mm from the center of a long cylindrical wire carrying a current of 10 A, we can use Ampere's Law. The formula for the magnetic field (B) inside a long cylindrical wire is given by:
B=μ0Ir2πR2
where:
- μ0 is the permeability of free space, μ0=4π×10−7T m/A
- I is the current (10 A)
- r is the distance from the center of the wire (2 mm = 2 × 10^{-3} m)
- R is the radius of the wire (5 mm = 5 × 10^{-3} m)
1. Convert units:
- The radius of the wire R=5mm=5×10−3m
- The distance from the center r=2mm=2×10−3m
2. Substitute values into the formula:
B=μ0Ir2πR2
Substitute μ0=4π×10−7T m/A, I=10A, r=2×10−3m, and R=5×10−3m:
B=(4π×10−7)×10×(2×10−3)2π(5×10−3)2
3. Simplify the equation:
- The π terms cancel out:
B=(4×10−7)×10×(2×10−3)2×(5×10−3)2
- Calculate (5×10−3)2=25×10−6:
B=(4×10−7)×10×(2×10−3)2×25×10−6
4. Calculate the numerator:
=4×10−7×10×2=80×10−7
5. Calculate the denominator:
=2×25×10−6=50×10−6
6. Combine the results:
B=80×10−750×10−6=8050×10−1=1.6×10−4T
Final Answer:
The magnetic field induction at a point 2 mm from the center of the wire is:
B=1.6×10−4T
- μ0 is the permeability of free space, μ0=4π×10−7T m/A
- I is the current (10 A)
- r is the distance from the center of the wire (2 mm = 2 × 10^{-3} m)
- R is the radius of the wire (5 mm = 5 × 10^{-3} m)
- The radius of the wire R=5mm=5×10−3m
- The distance from the center r=2mm=2×10−3m
B=μ0Ir2πR2
Substitute μ0=4π×10−7T m/A, I=10A, r=2×10−3m, and R=5×10−3m:
B=(4π×10−7)×10×(2×10−3)2π(5×10−3)2
- The π terms cancel out:
B=(4×10−7)×10×(2×10−3)2×(5×10−3)2
- Calculate (5×10−3)2=25×10−6:
B=(4×10−7)×10×(2×10−3)2×25×10−6
=4×10−7×10×2=80×10−7
=2×25×10−6=50×10−6
B=80×10−750×10−6=8050×10−1=1.6×10−4T
The magnetic field induction at a point 2 mm from the center of the wire is:
B=1.6×10−4T
Similar Questions
The magnetic induction at the point O, if the wire carries a current I, is
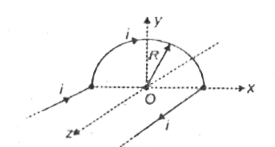
View SolutionA straight wire of length 0.5 metre and carrying a current of 1.2 ampere is placed in a uniform magnetic field of induction 2 tesla. If the magnetic field is perpendicular to the length of the wire , the force acting on the wire is
View SolutionA long wire carries a current of 4.00 A. Find the energy stored in the magnetic field inside a volume of 1.0mm3 at a distance of 10.0 cm from the wire.
View SolutionTwo long straight wires, each carrying a current I in opposite directions are separated by a distance R. The magnetic induction at a point mid way between the wire is
View Solution
A long wire bent as shown in the figure carries current I=10A. If the radius of the semicircular portion is 1 m, the magnetic induction (in μT) at the center C is
View SolutionA wire placed along north-south direction carries a current of 10 A from south to north. Find the magnetic field due to a 1 cm piece of wire at a point 200cm north-east from the piece.
View SolutionA circular coil of 200 mm diameter is made of 100 turns of thin wire and carries a current of 50 mA. Find the magnetic field induction at the centre of the coil.
View SolutionA long straight wire, carrying current I, is bent at its midpoint to form an angle of 45∘. Find the induction of magnetic field at point P, distant R from the point of bending (as shown in)

View SolutionA long straight wire carrying current of 30A is placed in an external unifrom magnetic field of induction 4×104T. The magnetic field is acting parallel to the direction of current. The maggnetic of the resultant magnetic inuduction in tesla at a point 2.0cm away form the wire is
View SolutionA long wire carrying current 'I' is bent into the shape as shown in the figure. The net magnetic field intensity at the centre 'O' is

View Solution
