Find the minimum wavelngth of X - ray emitted by X - ray tube , which is operating at 15kV accelerating voltage.
To find the minimum wavelength of X-rays emitted by an X-ray tube operating at an accelerating voltage of 15 kV, we can follow these steps:
1. Understand the Energy of the Accelerated Electrons:
The energy (E) of the electrons accelerated through a potential difference (V) is given by the formula:
E=eV
where e is the charge of an electron (1.6×10−19 C) and V is the accelerating voltage in volts.
2. Convert the Voltage:
Given that the voltage is 15 kV, we convert this to volts:
V=15kV=15×103V=15000V
3. Calculate the Energy of the Electrons:
Now, substituting the values into the energy formula:
E=(1.6×10−19C)×(15000V)=2.4×10−15J
4. Relate Energy to Wavelength:
The energy of a photon is related to its wavelength (λ) by the equation:
E=hcλ
where h is Planck's constant (6.63×10−34J s) and c is the speed of light (3×108m/s).
5. Rearranging for Wavelength:
Rearranging the equation to find the wavelength gives:
λ=hcE
6. Substituting the Values:
Now substituting the values of h, c, and E:
λ=(6.63×10−34J s)×(3×108m/s)2.4×10−15J
7. Calculating the Wavelength:
Performing the calculation:
λ=1.989×10−252.4×10−15=0.82875×10−10m=8.2875×10−11m
Converting to nanometers:
λ=0.82875Å≈0.83Å
8. Final Answer:
The minimum wavelength of the X-ray emitted is approximately:
λ≈0.83×10−10m=0.83nm
The energy (E) of the electrons accelerated through a potential difference (V) is given by the formula:
E=eV
where e is the charge of an electron (1.6×10−19 C) and V is the accelerating voltage in volts.
Given that the voltage is 15 kV, we convert this to volts:
V=15kV=15×103V=15000V
Now, substituting the values into the energy formula:
E=(1.6×10−19C)×(15000V)=2.4×10−15J
The energy of a photon is related to its wavelength (λ) by the equation:
E=hcλ
where h is Planck's constant (6.63×10−34J s) and c is the speed of light (3×108m/s).
Rearranging the equation to find the wavelength gives:
λ=hcE
Now substituting the values of h, c, and E:
λ=(6.63×10−34J s)×(3×108m/s)2.4×10−15J
Performing the calculation:
λ=1.989×10−252.4×10−15=0.82875×10−10m=8.2875×10−11m
Converting to nanometers:
λ=0.82875Å≈0.83Å
The minimum wavelength of the X-ray emitted is approximately:
λ≈0.83×10−10m=0.83nm
Similar Questions
find the minimum wavelength of the emitted X rays, when an X-ray tube is operated at 50 kV.
View SolutionThe shorted wavelength of X- rays emitted from an X- rays tube depends on
View SolutionThe shorted wavelength of X- rays emitted from an X- rays tube depends on
View SolutionFigure below shows a simple diagram of a modern X ray tube. (i.e. Coolidge tube).
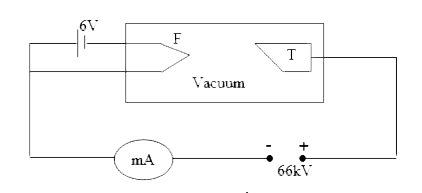
(i) Find the minimum wavelength of the X rays emitted by the X ray tube.
(ii) What will be the effect of replacing the 6V battery with a 9V battery on the emitted X rays?
View SolutionFind the maximum frequency, the X-ray emitted by an X-ray tube operating at 30kV
View SolutionFind the maximum frequency, the X-ray emitted by an X-ray tube operating at 30kV
View SolutionA: If the accelerating potential in an X- ray machine is decreased, the minimum value of the wavelength of the emitted X -rays gets increased.
R: The minimum value of wavelength of the emitted X-rays is inversely proportional to the accelerating potential.
View SolutionFind the cutoff wavelength for the continuous X-rays coming form an X-ray tube operating at 30 kV.
View SolutionFind the cut off wavelength for the continuous X - rays coming from an X-ray tube operating at 40 kV.
View SolutionFind the cut off walelength for the continuous X - rays coming
form an X-ray tube operating at 40 kv.
View Solution
