Assertion: At ordinary temperatures. the Vibrational degrees of freedom do not contribute to the specific heat of gases
Reason:The average charge corresponding to a degree of vibration is not kT and hence the molecular vibrations are not excited.
both Assertion and Reason are both are both wrong
Assertion is right, Reason is wrong
both Assertion and Reason are correct and Reason explains Assertion
both Assertion and Reason are correct, but Reason does not explain Assertion
The correct Answer is:C
To analyze the given assertion and reason, we will break down the concepts step by step.
Step 1: Understanding the Assertion
The assertion states that at ordinary temperatures, the vibrational degrees of freedom do not contribute to the specific heat of gases.
Explanation:
- In thermodynamics, specific heat refers to the amount of heat required to change the temperature of a substance. For gases, the specific heat is influenced by the degrees of freedom available to the molecules.
- At ordinary temperatures, the thermal energy (kT, where k is the Boltzmann constant and T is the temperature) is not sufficient to excite vibrational modes in gas molecules. Hence, these vibrational degrees of freedom do not contribute to the specific heat.
Step 2: Understanding the Reason
The reason provided states that the average energy corresponding to a degree of vibration is not kT, and thus the molecular vibrations are not excited.
Explanation:
- The energy levels for vibrational modes are quantized, meaning that molecules can only vibrate at specific energy levels.
- The lowest energy state for vibration is given by E = hν (where h is Planck's constant and ν is the frequency of vibration).
- At ordinary temperatures, the energy available (kT) is insufficient to reach these vibrational energy levels, leading to the conclusion that vibrational modes remain unexcited.
Step 3: Conclusion
Both the assertion and the reason are correct. The reason accurately explains why the vibrational degrees of freedom do not contribute to the specific heat of gases at ordinary temperatures.
Final Answer
Both the assertion and reason are true, and the reason correctly explains the assertion. Therefore, the correct option is C.
---
The assertion states that at ordinary temperatures, the vibrational degrees of freedom do not contribute to the specific heat of gases.
- In thermodynamics, specific heat refers to the amount of heat required to change the temperature of a substance. For gases, the specific heat is influenced by the degrees of freedom available to the molecules.
- At ordinary temperatures, the thermal energy (kT, where k is the Boltzmann constant and T is the temperature) is not sufficient to excite vibrational modes in gas molecules. Hence, these vibrational degrees of freedom do not contribute to the specific heat.
The reason provided states that the average energy corresponding to a degree of vibration is not kT, and thus the molecular vibrations are not excited.
- The energy levels for vibrational modes are quantized, meaning that molecules can only vibrate at specific energy levels.
- The lowest energy state for vibration is given by E = hν (where h is Planck's constant and ν is the frequency of vibration).
- At ordinary temperatures, the energy available (kT) is insufficient to reach these vibrational energy levels, leading to the conclusion that vibrational modes remain unexcited.
Both the assertion and the reason are correct. The reason accurately explains why the vibrational degrees of freedom do not contribute to the specific heat of gases at ordinary temperatures.
Both the assertion and reason are true, and the reason correctly explains the assertion. Therefore, the correct option is C.
Similar Questions
Knowledge Check
Assertion: Each vibrational mode gives two degrees of freedom.
Reason: By law of equipartition of energy, the energy for each degree of freedom in thermal equlibrium is 2kBT.AIf both assertion and reason are true and reason is the correct explanation os assertion.BIf both assertion and reason are true but reason is not be correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.Assertion : Heat added to a system at lower temperature causes greater randomness than when the same quantity of heat is added to it at higher temperature.
Reason : Entropy is a measure of the degree of randomness or disorder in the system.A(a) If both assertion and reason are true and reason is the correct explanation of assertion.B(b) If both assertion and reason are true but reason is not the correct explanation of assertionC(c) If assertion is true but reason is false.D(d) If both assertion and reason are false.
According to the tranistion state theory, for the formation of on activation complex, one of the vibrational degree of freedom is converted into the tranistion degree of freedom.
Reason (R ): The energy of the activated complex is higher than the energy of the reactant molecules.
View SolutionThe natural vibration frequency of a hydrogen molecule is 1.26×1014 Hz. Find the zero-point energy of the vibrations of the molecule. Can the vibrational degrees of freedom in the molecule be excited at 600 K?
View SolutionAssirtion Degree of freedom of a monjoatomic gas is always three, whether we concider vubrationaol effects or not.
Reason At all temperatures (low or high), vibrational kinetic energy of an ideal gas is zero
View SolutionAssertion: The resistivity of a semiconductor increases with temperature.
Reason: The atoms of a semiconductor vibrate with larger amplitude at higher temperature therby increasing it resistivity.
View SolutionAt ordinary temperatures, the molecules of an ideal gas have only translational and rotational kinetic energies. At high temperatures, they may also have vibrational energy. As a result of this, at higher temperatures, molar specific heat capacity at constant volume, CV is
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
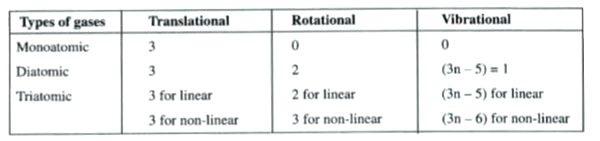
Where 'n' is atomicity of gas.
The vibrational kinetic energy of CO2 molecule is
View Solution
