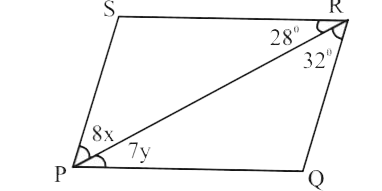
In the figure above, PQRS is a parallelogram and G is the centroid of the triangle PQR . A is the point of intersection of the diagonals PR and SQ. If AG=3 cm, then find the length of SQ.
The correct Answer is:18 cm
Step by step video, text & image solution for In the figure above, PQRS is a parallelogram and G is the centroid of the triangle PQR . A is the point of intersection of the diagonals PR and SQ. If AG=3 cm, then find the length of SQ. by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Similar Questions
Knowledge Check
- Question 1 - Select One
In the given figure, PQRS is parallelogram, then find the area of ΔPQX.
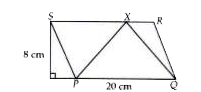 A80 cm2B40 cm2C120 cm2D60 cm2
A80 cm2B40 cm2C120 cm2D60 cm2 - Question 2 - Select One
The centroid of an equilateral triangle ΔPQR is L. If PQ = 18 cm, then the length of PL is :
A4√3cmB6√3cmC3√3cmD5√3cm - Question 3 - Select One
PQRS is a parallelogram in which PR is perpendicular to QS. If PR=8cm and QS=6 cm, then find PS
A5 cmB4 cmC7 cmD6 cm
PQR is an equilateral triangle. O is the point of intersection of altitudes PL,QM and RN. If OP=8cm, then what is the perimeter of the triangle PQR ?
View SolutionPQRS is a rhombus whose diagonals are PR=6 cm and QS=8 cm . If O is the point of intersection of diagonals and ∠PQO=θ then find the value of sinθ,tanθ and secθ
View SolutionPQRS is a square. PR and SQ intersect at O. Then ∠POQ is a
View SolutionPQRS is a Parallelogram and PQM is a triangle. If area of PQM=18cm2. Find the area of PQRS.
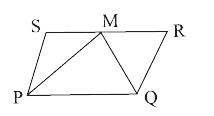 View Solution
View SolutionIn a rhombus PQRS , PR and QR are the diagonals of the rhombus. If PQ=10cm, then find the value of PR2+QS2.
View Solution