In a crystalline solid, atoms of X form fcc packing and atoms of Y occupy all octahedral voids. If all the atoms along one body diagonal are removed, what is the simplest formula of the crystalline lattice solid?
The correct Answer is:
To determine the simplest formula of the crystalline lattice solid when atoms along one body diagonal are removed, we can follow these steps:
Step 1: Understand the FCC Structure
In a face-centered cubic (FCC) structure, the atoms of X occupy:
- 8 corner positions, each contributing 18 of an atom to the unit cell.
- 6 face-centered positions, each contributing 12 of an atom to the unit cell.
Step 2: Calculate the Total Number of X Atoms
The total contribution of X atoms in the FCC unit cell can be calculated as follows:
- Contribution from corners: 8×18=1
- Contribution from face centers: 6×12=3
Thus, the total number of X atoms in the unit cell is:
1+3=4
Step 3: Understand the Octahedral Voids
In the FCC structure, the octahedral voids are located at:
- 12 edge centers (each contributing 14 of an atom to the unit cell).
- 1 body center (contributing 1 atom).
Step 4: Calculate the Total Number of Y Atoms
The total contribution of Y atoms occupying the octahedral voids can be calculated as follows:
- Contribution from edge centers: 12×14=3
- Contribution from body center: 1
Thus, the total number of Y atoms in the unit cell is:
3+1=4
Step 5: Remove Atoms Along the Body Diagonal
When all atoms along one body diagonal are removed, we need to identify which atoms are affected:
- The body diagonal connects two corner atoms and the body center atom.
- Therefore, we remove:
- 2 X atoms from the corners (each corner contributes 18).
- 1 Y atom from the body center.
Step 6: Calculate Remaining Atoms After Removal
After removing the atoms:
- Remaining X atoms:
4−2=2
- Remaining Y atoms:
4−1=3
Step 7: Write the Molecular Formula
The simplest formula can be written as:
X2Y3
Step 8: Simplify the Formula
To express the formula in its simplest form, we can divide both subscripts by their greatest common divisor (which is 1 in this case):
X2Y3
Final Answer
The simplest formula of the crystalline lattice solid is:
X2Y3
---
In a face-centered cubic (FCC) structure, the atoms of X occupy:
- 8 corner positions, each contributing 18 of an atom to the unit cell.
- 6 face-centered positions, each contributing 12 of an atom to the unit cell.
The total contribution of X atoms in the FCC unit cell can be calculated as follows:
- Contribution from corners: 8×18=1
- Contribution from face centers: 6×12=3
1+3=4
In the FCC structure, the octahedral voids are located at:
- 12 edge centers (each contributing 14 of an atom to the unit cell).
- 1 body center (contributing 1 atom).
The total contribution of Y atoms occupying the octahedral voids can be calculated as follows:
- Contribution from edge centers: 12×14=3
- Contribution from body center: 1
3+1=4
When all atoms along one body diagonal are removed, we need to identify which atoms are affected:
- The body diagonal connects two corner atoms and the body center atom.
- Therefore, we remove:
- 2 X atoms from the corners (each corner contributes 18).
- 1 Y atom from the body center.
After removing the atoms:
- Remaining X atoms:
4−2=2
- Remaining Y atoms:
4−1=3
The simplest formula can be written as:
X2Y3
To express the formula in its simplest form, we can divide both subscripts by their greatest common divisor (which is 1 in this case):
X2Y3
The simplest formula of the crystalline lattice solid is:
X2Y3
Similar Questions
A crystal is made of particles X and Y.X form fcc packing and Y occupies all the octahedral voids . If all the particles along one body diagonal are removed then the formula of the crystal would de :
View SolutionA crystal is made of particles X,Y and Z.X form fcc packing . Y occupies all the octahedral void of X and Z occupies all the tetrahedral voids of X . If all the particles along one body diagonal are removed then the formula of the crystal would be:
View SolutionA crystal is made of particles X,Y and Z.X form fcc packing . Y occupies all the octahedral void of X and Z occupies all the tetrahedral voids of X . If all the particles along one body diagonal are removed then the formula of the crystal would be:
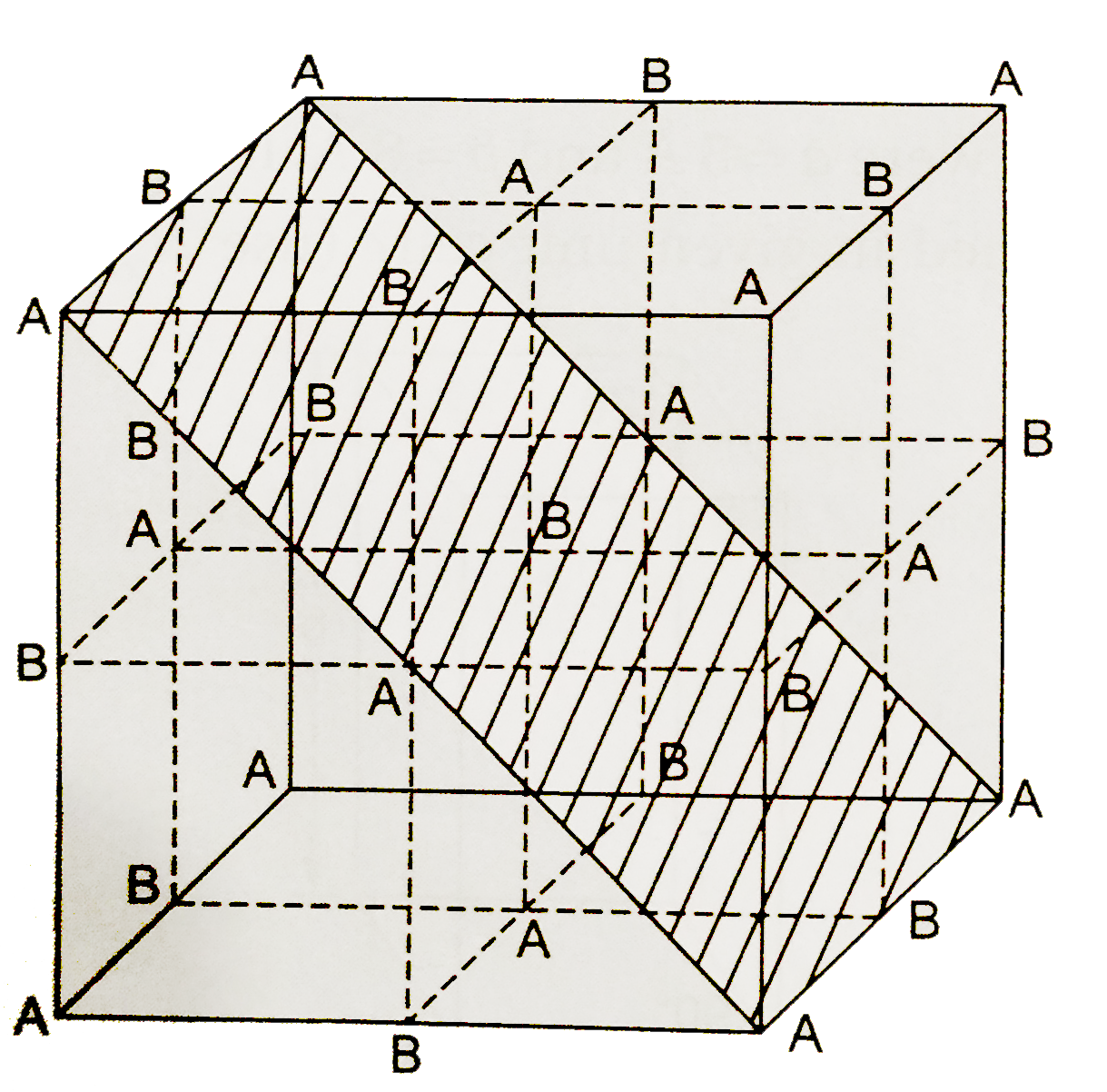
View SolutionA crystal is made of particles A and B . From fcc packing and B occupies all the octahedral voids . If all the particle along the plane as shown in figure are removed , then, the formula of the crystal would be :
View SolutionA2B has antifluorite structure (B forms FCC lattice and A occupies tetrahedral voids).If all ions along any one body diagonal are removed, then new formula of compound will be :
View SolutionA compound made of particles A, B, and C forms p lattice. In the lattice, ions A occupy the lattice points and ions B and C occuphy the alternate TVs. If all the ions along one of the body diagonals are removed, then formula of the compound is
View SolutionIn a solid having rock salt structure, if all tge atoms touching one body diagonal plane are removed (except at body centre), then the formula of the left unit cell is
View SolutionIn a crystalline solid, anions B arranged in ccp lattice and cations A occupy 50% of the octahedral voids. And 50% of the tetrahedral voids. What is the formula of the solid?
View SolutionIn a solid AB, A atoms have a ccp arrangement and B atoms occupy all the octahedral sites. If all the face-centred atoms along one of the axes passing through body centres are removed, then what will be the resultant stoichiometry of compound ?
View SolutionIn a solid between A and B atoms of A are arranged in ccp array and atoms of B occupy all the octahedral voids and half of the tetrahedral voids. The formula of the compound is
View Solution
