Assertion (A) : Hydrogen has only one electron in its 1s orbital but it produces several spectral lines.
Reason (R) : There are many excited energy levels available in H atoms.
Both A and R true and R is the correct explanation of A
Both A and R are true but R is not a correct explanation of A
A is true but R is false
A is false but R is true
The correct Answer is:A
To solve the question, we need to analyze both the assertion and the reason provided.
1. Understanding the Assertion (A):
- The assertion states that hydrogen has only one electron in its 1s orbital but produces several spectral lines.
- Hydrogen's electron configuration is indeed 1s¹, meaning it has one electron in the first energy level (1s orbital).
2. Understanding the Reason (R):
- The reason states that there are many excited energy levels available in hydrogen atoms.
- Hydrogen has multiple energy levels (n = 1, 2, 3, 4, etc.), and when the single electron in hydrogen absorbs energy, it can be excited to these higher energy levels.
3. Linking Assertion and Reason:
- When the electron in hydrogen is excited to a higher energy level and then returns to a lower energy level (ground state), it emits energy in the form of light.
- This emitted light corresponds to specific wavelengths, which result in the spectral lines observed in hydrogen's emission spectrum.
4. Conclusion:
- Both the assertion and reason are true. The reason correctly explains why the assertion is true: the presence of multiple excited energy levels allows the single electron in hydrogen to produce several spectral lines upon returning to lower energy states.
5. Final Answer:
- Therefore, the correct conclusion is that both the assertion and reason are true, and the reason is the correct explanation for the assertion.
- The assertion states that hydrogen has only one electron in its 1s orbital but produces several spectral lines.
- Hydrogen's electron configuration is indeed 1s¹, meaning it has one electron in the first energy level (1s orbital).
- The reason states that there are many excited energy levels available in hydrogen atoms.
- Hydrogen has multiple energy levels (n = 1, 2, 3, 4, etc.), and when the single electron in hydrogen absorbs energy, it can be excited to these higher energy levels.
- When the electron in hydrogen is excited to a higher energy level and then returns to a lower energy level (ground state), it emits energy in the form of light.
- This emitted light corresponds to specific wavelengths, which result in the spectral lines observed in hydrogen's emission spectrum.
- Both the assertion and reason are true. The reason correctly explains why the assertion is true: the presence of multiple excited energy levels allows the single electron in hydrogen to produce several spectral lines upon returning to lower energy states.
- Therefore, the correct conclusion is that both the assertion and reason are true, and the reason is the correct explanation for the assertion.
Similar Questions
How many spectral lines are emitted by atomic hydrogen excited to the n−th energy level?
View SolutionAssertion (A) : When the transition element ionises, the 4s-orbital electrons are removed before the 3d-orbital electrons.
Reason (R ) : The energy of 3d-orbital electrons is lower than that of 4s-orbital electrons.
View Solution
Knowledge Check
Assertion: Hydrogen atom consists of anly one electron but its emission spectrum has may lines.
Reason: Only Lyman series is found in the absorption spectrum of hydrogen atom whereas in the emission spectrum, all the series are found.Aif both assertion and reason are true and reason is the correct explanation of assertion.Bif both assertion and reason are true but reason is not the correct explanation of assertion.Cif assertion is true but reason is false.Dif both assertion and reason are false.
Hydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
The energy required to promote the ground state electron of H-atom to the first excited state is:
When an electron returns from a higher energy level to a lower energy level, energy is given out in the form of UV//Visible radiation.
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
The energy in Joule of an electron in the second orbit of H- atom is:
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
Calculate the following :
(a) the kinetic energy (in eV) of an electron in the ground state of hydrogen atom.
(b) the potential energy (in eV) of an electron in the ground state of hydrogen atom.
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
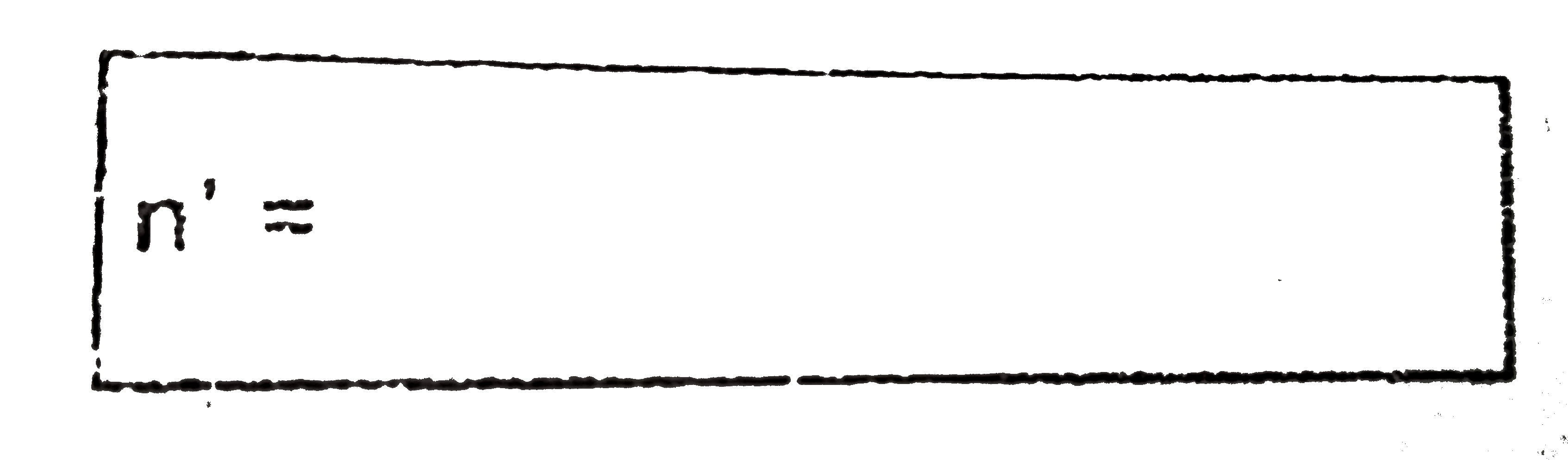
What is the principal quantum number, n' of the orbit of Be3 that has the same Bohr radius as that of ground state hydrogen atom ?
View SolutionHydrogen atom:
The electronic ground state of hydrogen atom contains one electron in the first orbit. If sufficient energy is provided, this electron can be promoted to higher energy levels. The electronic energy of a hydrogen-like species (any atom//ions with nuclear charge Z and one electron) can be given as
En=−RHZ2n2 where RH=Rydberg constant,n=principal quantum number
Calculate the wavelength of light (nm) for the electronic transition of H-atom from the first excited state to ground state.
In the model of hydrogen like atom put forward by Niels Bohr (1913) the electron orbits around the central nucleus. the bohr radius of nth orbit of a hydrogen-like species is given by
r=kn2Z " " where, k is constant
View SolutionAssertion (A) : In an atom, the velocity of electron in the higher orbits keeps on decreasing
Reason (R ) : Velocity of electron in inversely proportional to the radius of the orbit
View SolutionThe energy of the electron in the 3d orbital is less than that in the 4s orbital in the hydrogen atom
View Solution
