Each question contains statements given in two columns which have to be matched. Statements (a,b,c,d) in column I have to be matched with statements (p,q,r,s) in column II. If the correct match are a-p, s, b-r c-p, q. and d-s, then the correctly bubbled 4x4 matrix should be as follows: figure Column I, a) If ∫2x√1−4xdx=ksin−1(f(x))+C, then kis greater than, b) If ∫(√x)5(√x)7+x6dx=aln(xk)xk+1+c, then akis less than, c) If ∫x4+1x(x2+1)2dx=kln|x|+m1+x2+n, where nis the constant of integration, then mkis greater than, d) If ∫dx5+4cosx=ktan−1(mtanx2)+C, then k/mis greater than, COLUMN II p) 0 q) 1 r) 3 s)4Solution in Bengali
Step by step video & image solution for Each question contains statements given in two columns which have to be matched. Statements (a,b,c,d) in column I have to be matched with statements (p,q,r,s) in column II. If the correct match are a-p, s, b-r c-p, q. and d-s, then the correctly bubbled 4x4 matrix should be as follows: figure Column I, a) If int(2^x)/(sqrt(1-4^x))dx=ksin^(-1)(f(x))+C , then kis greater than, b) If int((sqrt(x))^5)/((sqrt(x))^7+x^6)dx=aln(x^k)/(x^k+1)+c , then a kis less than, c) If int(x^4+1)/(x(x^2+1)^2)dx=kln|x|+m/(1+x^2)+n , where nis the constant of integration, then m kis greater than, d) If int(dx)/(5+4cosx)=ktan^(-1)(mt a n x/2)+C , then k//mis greater than, COLUMN II p) 0 q) 1 r) 3 s)4 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGE PUBLICATIONCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type
11 Videos
- DIFFERENTIATIONBOOK - CENGAGE PUBLICATIONCHAPTER - DIFFERENTIATIONEXERCISE - Archives
14 Videos
Similar Questions
Let →a=a1ˆi+a2ˆj+a3ˆk,→b=b1ˆi+b2ˆj+b3ˆkand→c=c1ˆi+c2ˆj+c3ˆk be three non-zero vectors such that →c is a unit vector perpendicular to both →aand→b. If the angle between →aand→bisπ/6 then the value of ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣is
View SolutionLet y be an implicit function of x defined by x2x−2xxcoty−1=0. Then y'(1) equals: a.1 b. log2 c. −log2 d. −1
View SolutionEach question contains statements given in two columns which have to be matched. Statements (a,b,c,d) in column I have to be matched with statements (p,q,r,s) in column II. If the correct match are a-p, s, b-r c-p, q. and d-s, then the correctly bubbled 4x4 matrix should be as follows: figure Column I, a) If ∫2x√1−4xdx=ksin−1(f(x))+C, then kis greater than, b) If ∫(√x)5(√x)7+x6dx=aln(xk)xk+1+c, then akis less than, c) If ∫x4+1x(x2+1)2dx=kln|x|+m1+x2+n, where nis the constant of integration, then mkis greater than, d) If ∫dx5+4cosx=ktan−1(xtanx2)+C, then k/mis greater than, COLUMN II p) 0 q) 1 r) 3 s)4
View Solution∫2x√1−4xdx=ksin−12x+c, then k =
View SolutionConsider
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.
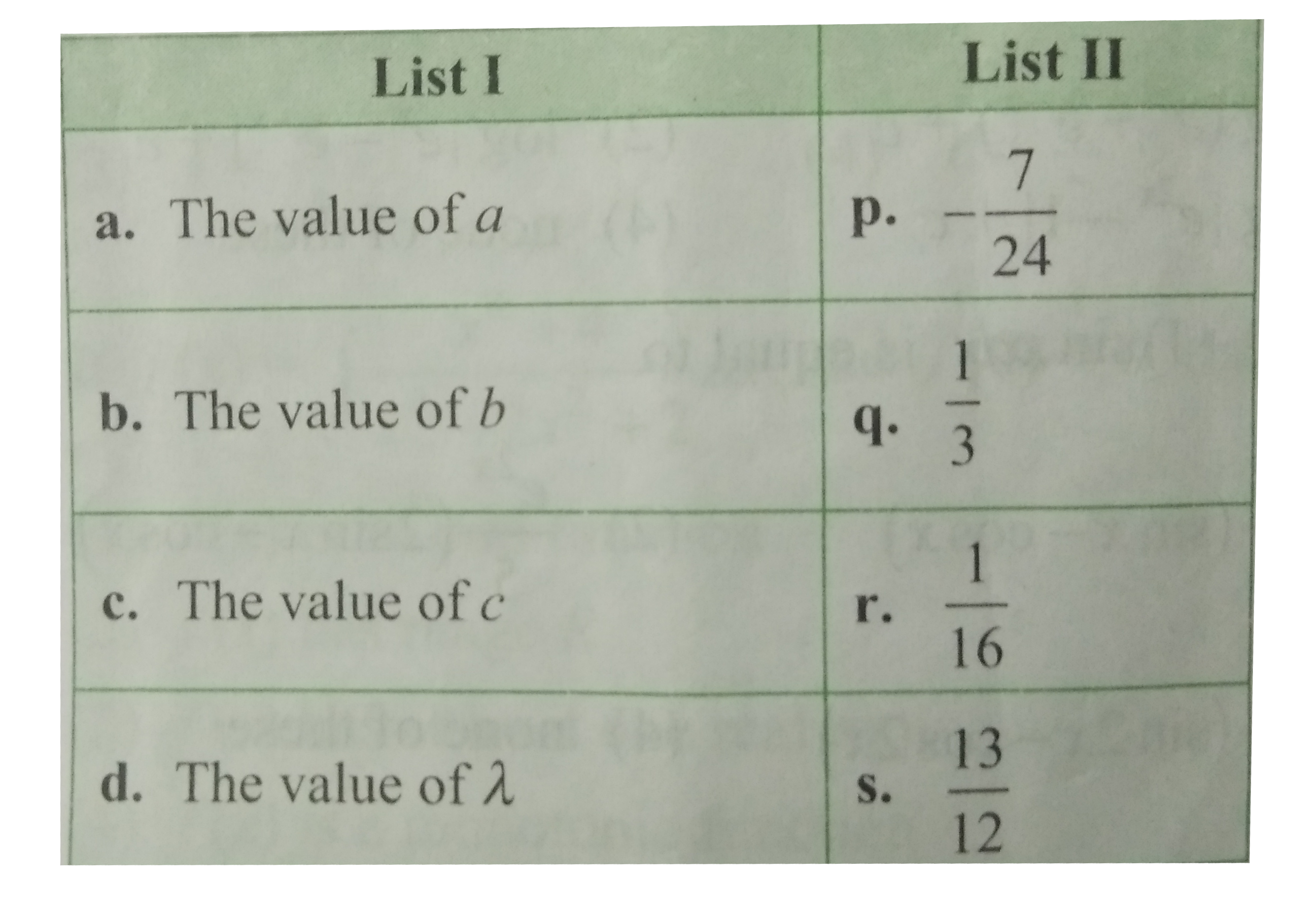
Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr
View SolutionLet f(x)=|x−1|+|x−2|+|x−3| , match the column I for the value of k column II.
Column - I Column -II(A) f(x) = k has no solution (p) 1 (B) f(x)=k has only one solution(q) 2 (C) f(x) = k has two solution of same sign (r) 4(D) f(x)= k has two solution of opposite sign(s) 5(t) 8
View Solution{:(,"Column-I",,"Column-II"),("(a)","Zero",,(p)k=1/(2t)[1/(a-x)^(2)-1/a^(2)]),("(b)","First",,(q)k=1/t[1/(a-x)-1/a]),("(c)","Second",,(r)k=x/t),("(d)","Third",,(s)k=1/tloge(a/((a-x)))):}
View SolutionIf ∫2x√1−4xdx=KSin−1(2x)+c, then K=
View SolutionEach question contains statements given in two columns which have to be matched. Statements (a,b,c,d) in column I have to be matched with statements (p,q,r,s) in column II. If the correct match are a-p, s, b-r c-p, q. and d-s, then the correctly bubbled 4x4 matrix should be as follows: figure Column I, a) If ∫2x√1−4xdx=ksin−1(f(x))+C, then kis greater than, b) If ∫(√x)5(√x)7+x6dx=aln(xk)xk+1+c, then akis less than, c) If ∫x4+1x(x2+1)2dx=kln|x|+m1+x2+n, where nis the constant of integration, then mkis greater than, d) If ∫dx5+4cosx=ktan−1(mtanx2)+C, then k/mis greater than, COLUMN II p) 0 q) 1 r) 3 s)4
View SolutionEach question contains statements given in two columns which have to be matched. Statements a,b,c,d in column I have to be matched with statements p,q,r,s in column II. If the correct matches are a-p, q-s, b-q, r c-p, q and d-s, then the correctly bubbled 4x4 matrix should be as follows: Figure Column I, Column II: Differential equation order 1, p. of all parabolas whose axis is the x-axis order 2, q. of family of curves y=a(x+a)2, whre a is an arbitrary constant degree 1, r. (1+3dydx)23=4d3ydx3 degree 3, s. of family of curve y2=2c(x+√c), where c>0
View SolutionConsider
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.

Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr
View Solutionकॉलम Iकॉलम II(A)यदि∫2x√1−4xdx=ksin−1(f(x))+C,(p)0तो k किससे महत्तम है(B)यदि∫(√x)5(√x)7+x6dx=a lnxkxk+1+c,(q)1हो, तो ak किससे न्यूनतम है(C)∫x4+1x(x2+1)2dx=k ln|x|+m1+x2+n,(r)3जहाँ n समाकलन का अचर है, तो mk किससे महत्तम है(D)∫dx5+4cosx=ktan−1(m tanx2)+C,(s)4 हो k/m किससे महत्तम है
View SolutionLet f(x)=|x−1|+|x−2|+|x−3| , match the column I for the value of k column II.
Column - I Column -II(A) f(x) = k has no solution (p) 1 (B) f(x)=k has only one solution(q) 2 (C) f(x) = k has two solution of same sign (r) 4(D) f(x)= k has two solution of opposite sign(s) 5(t) 8
View Solution∫2x√1−4xdx=ksin−12x+c, then k =
View SolutionConsider
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.

Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr
View SolutionEach question contains statements given in two columns which have to be matched. Statements a,b,c,d in column I have to be matched with statements p,q,r,s in column II. If the correct matches are a-p, q-s, b-q, r c-p, q and d-s, then the correctly bubbled 4x4 matrix should be as follows: Figure Column I, Column II: Differential equation order 1, p.of all parabolas whose axis is the x-axis order 2, q.of family of curves y=a(x+a)2, where a is an arbitrary constant degree 1, r. (1+3dydx)23=4d3ydx3 degree 3, s. of family of curve y2=2c(x+√c), where c>0
View Solution
