Statement 1: The shortest distance between the lines x−3=y−11=z+1−1andx−21=y−32=(z+(13/7)−1)is zero. Statement 2: The given lines are perpendicular.Solution in Bengali
Step by step video & image solution for Statement 1: The shortest distance between the lines x/(-3)=(y-1)/1=(z+1)/(-1)a n d(x-2)/1=(y-3)/2=((z+(13//7))/(-1))is zero. Statement 2: The given lines are perpendicular. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
View SolutionIf the distance between the plane ax 2y + z = d and the plane containing the lines x−12=y−23=z−34 and x−23=y−34=z−45 is √6 , then value of |d| is
View SolutionStatement 1: The shortest distance between the lines x−3=y−11=z+1−1andx−21=y−32=(z+(13/7)−1)is zero. Statement 2: The given lines are perpendicular.
View SolutionEquation of the line of the shortest distance between the lines (x)/(1)=(y)/(-1)=(z)/(1) and (x-1)/(0)=(y+1)/(-2)=(z)/(1) is:
View SolutionFind the length and the equations of the line of shortest distance between the lines
x+17=y+1−6=z+11 and x−31=y−5−2=z−71
View SolutionStatement 1: The shortest distance between the lines x2=y−1=z2 and x−1−2=y−1−2=z−14 is √2. Statement 2: The shortest distance between two parallel lines is the perpendicular distance from any point on one of the lines to the other line.
View SolutionFind the shortest distance distance between the following lines :
x−1−1=y+21=z−3−2,x−11=y+12=z+1−2
View SolutionThe shortest distance between lines
L1:x+13=y+21=z+12,
L2:x−21=y+22=z−33 is
View Solutionरेखा के मध्य लघुतम दुरी ज्ञात कीजिए:
x2=y−3=z1 और x−23=y−1−5=z+22
View SolutionIf 1,m ,n are the direction cosines of the line of shortest distance between the lines x−32=y+15−7=z−95andx+12=y−11=z−9−3 then :
View SolutionShow that the lines x−31=y−5−2=z−71 and x−17=y−16=z−17 are skew lines and find the shortest distance between them.
View SolutionEquation of the line of shortest distance between the lines x1=y−1=z1andx−10=y+1−2=z1 is
View SolutionStatement 1: The shortest distance between the lines x−3=y−11=z+1−1andx−21=y−32=(z+(13/7)−1)is zero. Statement 2: The given lines are perpendicular.
View SolutionShortest distance between the lines x−11=y−11=z−11andx−21=y−31=z−41 is equal to
View SolutionFor an ideal gas, an illustration of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.
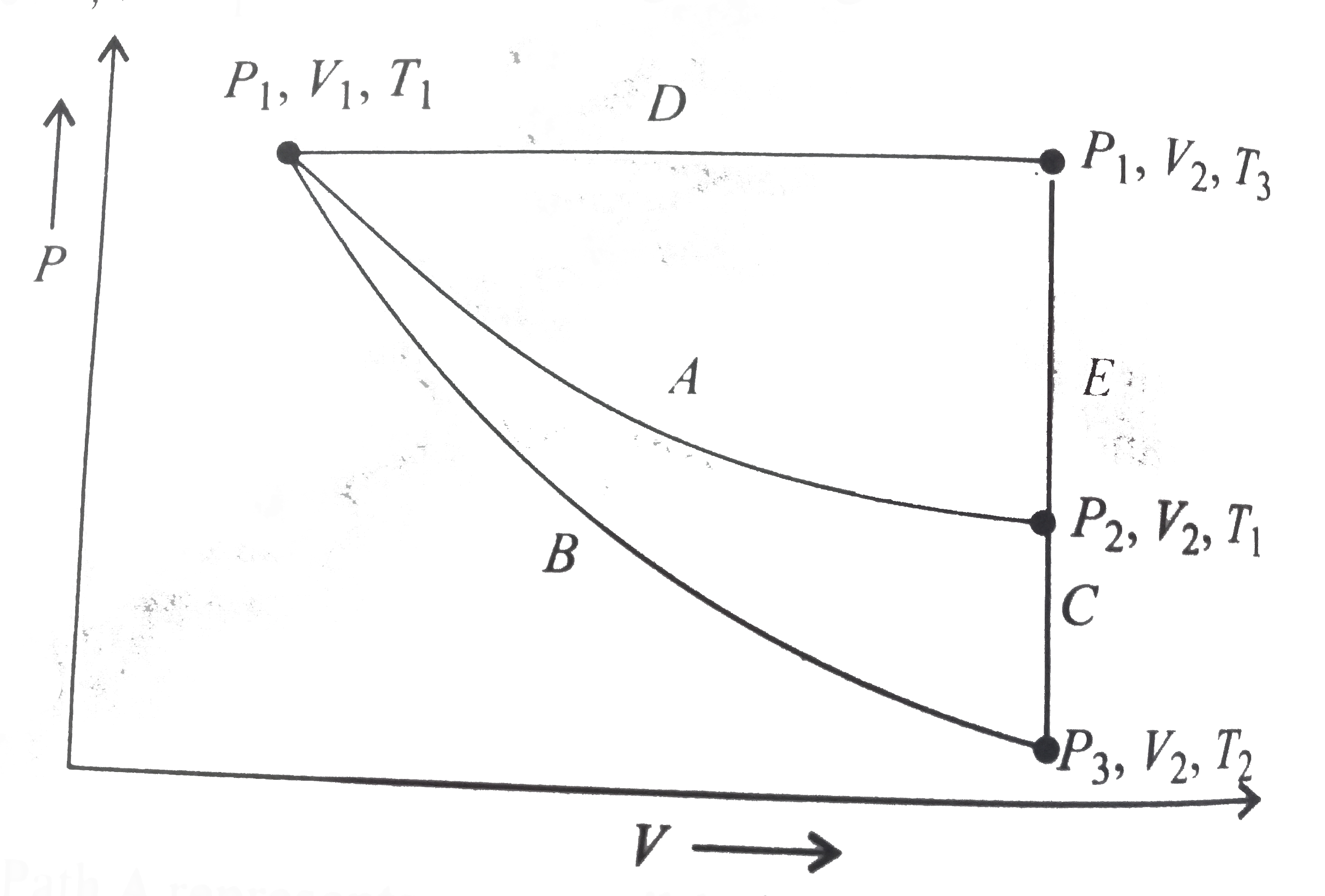
Path A represents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is ΔS for path (D+E)?
View SolutionThe shortest distance between lines L_(1):(x+1)/(3)=(y+2)/(1)=(z+1)/(2) , L_(2):(x-2)/(1)=(y+2)/(2)=(z-3)/(3) is
View Solution
