Draw the graph of y=cot−1x+sec−1x+cosec−1x. Solution in Bengali
Video Solution
Text Solution
We have y=f(x)=cot−1x+sec−1+x+cosec−1
=cot−1x+π2
Clearly, the domain of the function is (−∞,01)]∪[1,∞)
Therefore, to draw the graph of the function, we shift the graph of y=cot−1x,'π'2 units upward for x∈(−∞,−1]∪[1,∞)
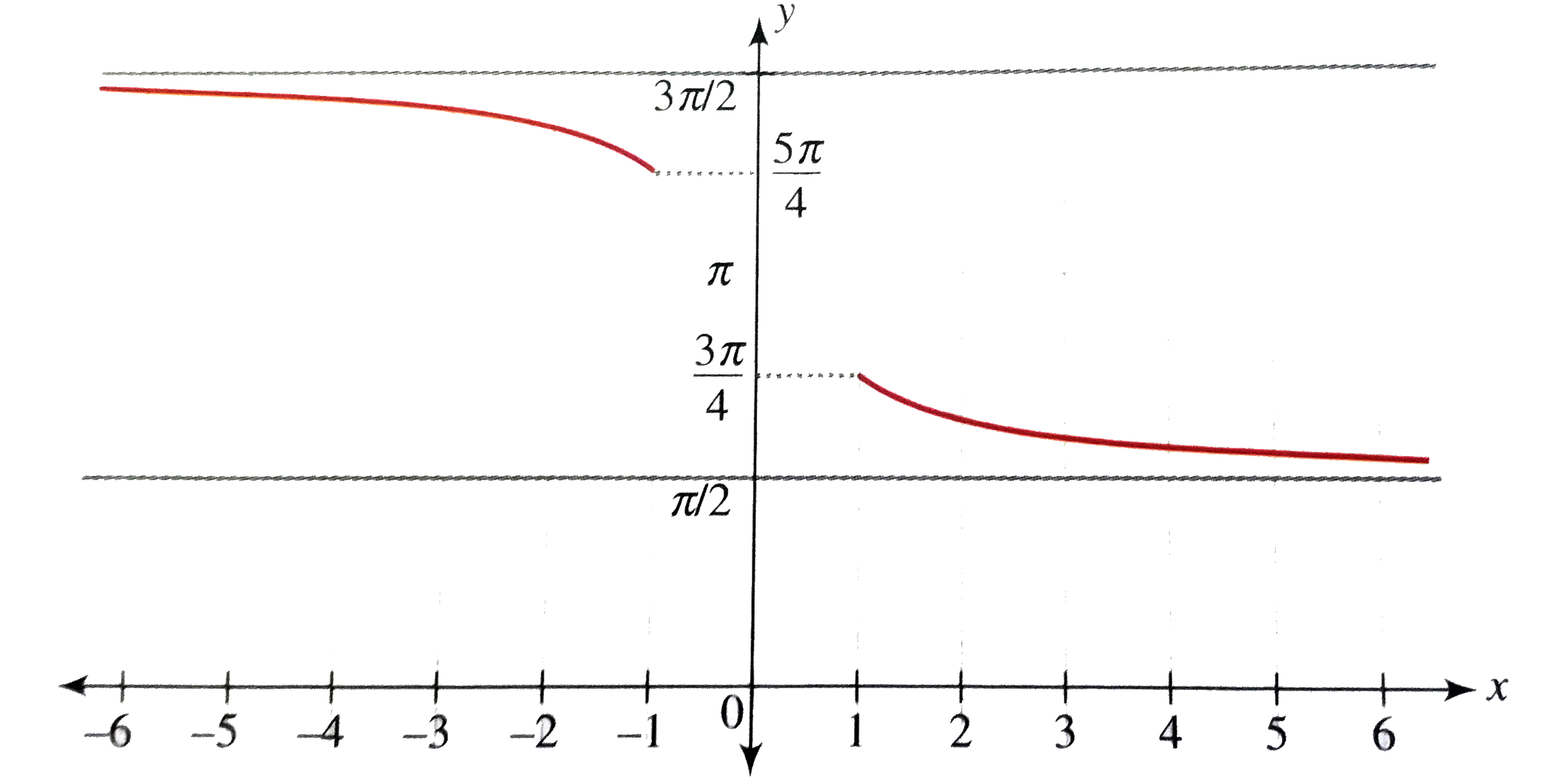
|
Topper's Solved these Questions
Similar Questions
Draw the graph of y=tan−1x−cot−1x.
View SolutionDraw the graph of y=(x−1)(x2−x+1).
View SolutionDraw the graph of y=cot−1x+sec−1x+cosec−1x.
View SolutionDraw the graph of y=sec−1x+cosec−1x
View SolutionDraw the graph of y=cot−1(cotx)
View SolutionDraw in graph of y= cosec−1(cosec x).
View SolutionDraw the graph of y=cosec(cosec−1x) or y=sec(sec−1x).
View SolutionIf y=tan−1x+cot−1x+sec−1+cosec−1x.thendydx is equal to
View Solutiontanx+1cosec x+1secx+cotx
View SolutionDraw the graph of y=cot−1x+sec−1x+cosec−1x.
View SolutionDraw the graph of y=sec−1x+cosec−1x
View SolutionDraw the graph of y=cot−1(cotx)
View SolutionDraw in graph of y= cosec−1(cosec x).
View SolutionDraw the graph of y=cosec(cosec−1x) or y=sec(sec−1x).
View SolutionIf y=tan−1x+sec−1x+cot−1x+cosec−1x,
then dydx is equal to
View SolutionDraw the graph of y=cot^(-1)x+sec^(-1)x+cosec^(-1)x .
View Solution
