The distance of point A(−2,3,1) from the line PQ through P(−3,5,2), which makes equal angles with the axes is a. 2/√3 b. √14/3 c. 16/√3 d. 5/√3Solution in Bengali
2/√3
√14/3
16/√3
5/√3
The correct Answer is:B
Here, α=β=γ
∵cos2α+cos2β+cos2γ=1
∵cosα=1√3
Direction cosines of PQ are (1√3,1√3,1√3)
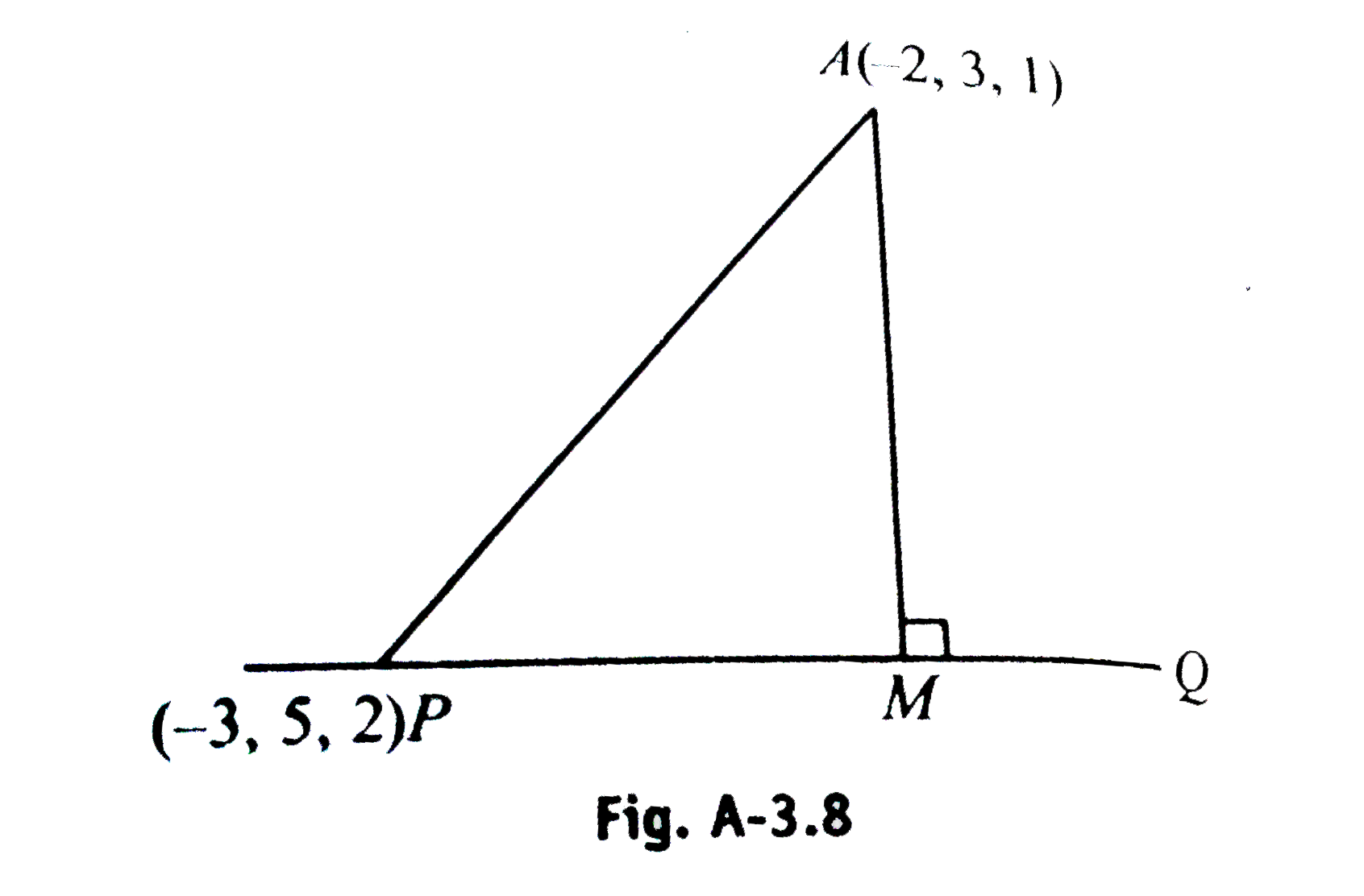
PM=Projection of AP on PQ
=∣∣∣(−2+3)1√3+(3−5)1√3+(1−2)1√3∣∣∣
=2√3
and AP=√(−2+3)2+(3−5)2+(1−2)2=√6
AM=√(AP)2−(PM)2=√6−43=√143
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - MULTIPLE CORRECT ANSWER TYPE17videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - REASONING TYPE10videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - SUBJECTIVE TYPE17videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions291videos
THREE DIMENSIONAL GEOMETRY
- BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Numerical value type)4videos
TRIGONOMETRIC EQUATIONS
Similar Questions
Let PM be the perpendicular from the point P(1,2,3) to the x−y plane. If →OP makes an angle θ with the positive direction of the z− axis and→OM makes an angle φ with the positive direction of x− axis, whereO is the origin and θandφ are acute angels, then a. cosθcosφ=1/√14 b. sinθsinφ=2/√14 c. tanφ=2 d. tanθ=√5/3
03:16View SolutionStatement 1: Lines →r=ˆi+ˆj−ˆk+λ(3ˆi−ˆj)and→r=4ˆi−ˆk+μ(2ˆi+3ˆk) intersect. Statement 2: →b×→d=0 , then lines →r=→a+λ→band→r=→c+λ→d do not intersect.
04:57View SolutionIf the direction cosines of a variable line in two adjacent points be l,m,nandl+δl,m+δm,n+δn the small angle δθas between the two positions is given by
06:25View SolutionFind the angle between the line whose direction cosines are given by l+m+n=0andl2+m2−n2=0.
07:24View SolutionThe number of all possible values of θ, where 0<θ<π, for which the system of equations (y+z)cos3θ=(xyz)sin3θ,xsin3θ=2cos3θy+2sin3θzand(xyz)sin3θ=(y+2z)cos3θ+ysin3θ have a solution (x0,y0,z0) wiith y0z0≠0 is
01:23View SolutionIf the axes are rotated through 30^(@) in the anti- clockwise direction,the coordinates of points (4,-2sqrt(3)) with respect to new axes are (A)(2,sqrt(3)) (B) (sqrt(3),-5) (C) (2,3) (D) ( sqrt(3),2)
04:35View SolutionA hyperbola passes through the point P(sqrt(2),sqrt(3)) and has foci at (+-2,0) them the tangent to this hyperbola at P also passes through the point (A)(sqrt(3),sqrt(2))(B)(-sqrt(2),-sqrt(3))(C)(3sqrt(2),2sqrt(3))(D)(2sqrt(2),3sqrt(3))
03:41View SolutionThe distance of point A(−2,3,1) from the line PQ through P(−3,5,2), which makes equal angles with the axes is a. 2/√3 b. 14/√3 c. 16/√3 d. 5/√3
04:55View SolutionIf P+sqrt(3)Q+sqrt(5)R+sqrt(15)S=(1)/(1+sqrt(3)+sqrt(5)) then the value of P is
03:42View SolutionIf x=√5+√3√5−√3 and y=√5−√3√5+√3, then (x+y) equals (a) 2(√5+√3) (b) 2√15 (c) 8 (d) 16
04:19View SolutionIf p=3−√53+√5andq=3+√53−√5,find the value of p2+q2.
05:18View SolutionP and Q are points on the line joining A(−2,5) and B(3,1) such that AP=PQ=QB . Then the distance of the midpoint of PQ from the origin is (a)3 (b) √372 (c) 4 (d) 3.5
04:29View SolutionIf tangents PQ and PR are drawn from a point on the circle x2+y2=25 to the ellipse x216+y2b2=1,(b<4), so that the fourth vertex S of parallelogram PQSR lies on the circumcircle of triangle PQR , then the eccentricity of the ellipse is
(a)√54
(b) √74
(c) √72
(d) √5307:15View SolutionThe distance of point A(−2,3,1) from the line PQ through P(−3,5,2), which makes equal angles with the axes is a. 2/√3 b. √14/3 c. 16/√3 d. 5/√3
03:34View SolutionThe distance of point A(−2,3,1) from the line PQ through P(−3,5,2), which makes equal angles with the axes is
01:53View SolutionThe distance of point A(-2,3,1)from the line PQ through P( 3,5,2), which make equal angles with the axes is
03:23View Solution

